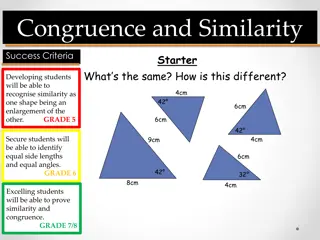
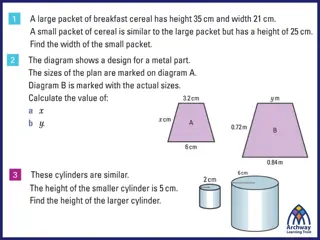
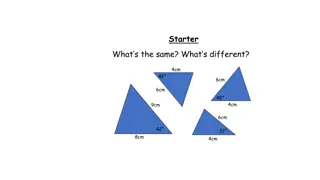
Understanding Triangle Congruence Criteria in Geometry
Explore the concept of congruence criteria for triangles in geometry, including SSS, SAS, ASA Postulates, and AAS Theorem. Learn to identify congruent figures and their corresponding parts, solve problems, and prove relationships in geometric figures. Dive into the principles of congruent polygons, recognizing matching sides and angles. Discover how to prove triangles congruent using different postulates and theorems, which involve specific combinations of sides and angles. Enhance your geometric reasoning and problem-solving skills through engaging examples and visuals.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Chapter 4.1 Common Core - G.SRT.5 Use congruence criteria for triangles to solve problems and prove relationships in geometric figures. Objectives To recognize congruent figures and their corresponding parts.
Ch 4.1 Notes Congruent Polygons have congruent corresponding parts their matching side and angles. When you name congruent polygons, you must list corresponding vertices in the same order.
If 2 triangles are then they have 3 corres- ponding sides and 3 corresponding s. Corr. Sides 1) 2) 3) A X Corr. Angles 1) 2) 3) B C Y Z
Chapter 4.2 Common Core -G.SRT.5 Use congruence criteria for triangles to solve problems and prove relationships in geometric figures. Objectives To prove two triangles congruent using SSS and SAS Postulates.
Ch 4.2 Notes Side-Side-Side Post. (SSS) if 3 sides of one triangle are to 3 sides of another triangle, then the 2 triangles are congruent Side-Angle-Side Post. (SAS) if 2 sides and the included of one triangle are to 2 side and the included angle of a second triangle, then the 2 triangles are .
Chapter 4.3 Common Core - G.SRT.5 Use congruence criteria for triangles to solve problems and prove relationships in geometric figures. Objectives To prove two triangles congruent using ASA Postulate and AAS Theorem.
Ch 4.3 Notes Angle-Side-Angle Post. (ASA) if 2 s and the included side of one triangle are to 2 s and the included side of a second triangle, then the 2 triangles are congruent Angle-Angle-Side Post. (AAS) if 2 s and a nonincluded side of one triangle are to 2 s and the corresponding nonincluded side of a second triangle, then the 2 triangles are .
Chapter 4.4 Common Core - G.SRT.5 & G.CO.12 Use congruence criteria for triangles to solve problems and prove relationships in geometric figures. Objectives To use triangle congruence and corresponding parts of congruent triangles to prove that parts of two triangles are congruent.
Ch 4.4 Notes Once you have 2 triangles then you can say anything you want about their corresponding parts. (It is called Corresponding Parts of Congruent Triangles are Congruent) *You can use the acronym C.P.C.T.C
Chapter 4.5 Common Core Common Core - G.CO.10, G.CO.13 & G.SRT.5 Prove theorems about triangles base angles of isosceles triangles are congruent. Objectives To use and apply properties of isosceles and equilateral triangles.
Ch 4.5 Notes Isosceles Triangle Equilateral Triangle Leg Leg Base
Base Angle Thm if 2 sides of a triangle are , then the angles opposite them are . If then If AB AC, the B C Converse of the Base Angles Thm If 2 s of a triangle are , then the sides opposite them are . If then
If a line bisects the vertex angle of an isosceles triangle, then the line is also the perpendicular bisector of the base. If then
Corollaries If a triangle is equilateral, then it is equiangular. If then If a triangle is equiangular, then it is equilateral. If then
Chapter 4.6 Common Core G.SRT.5 Use congruence criteria to solve problems and prove relationships in geometric figures. Objective To prove right triangles congruent suing the Hypotenuse-Leg Theorem.
Ch 4.6 Notes Hypotenuse-Leg Congruence Thm (HL) If the hypotenuse and a leg of a right triangle are to the hypotenuse and a leg of a second right triangle, then the 2 triangles are . A D B C E F If BC EF and AC DF, then ABC DEF
Chapter 4.7 Common Core G.SRT.5 Use congruence criteria to solve problems and prove relationships in geometric figures. Objectives To identify congruent overlapping triangles. To prove two triangles congruent using other congruent triangles.
Ch 4.7 Notes Congruence in Overlapping Triangles - You can sometimes use the congruent corresponding parts of one pair of congruent triangles to prove another pair of triangles congruent. Review the five way to prove to two triangles congruent. 1) 2) 3) 4) 5)