Understanding Sectional Modulus and Bending Stress in Beams
Explore the concept of sectional modulus in beams and how it relates to bending stress. Learn about calculating maximum stress, moment of inertia, and solving bending stress problems in various beam sections. Dive into case studies to apply these principles practically.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
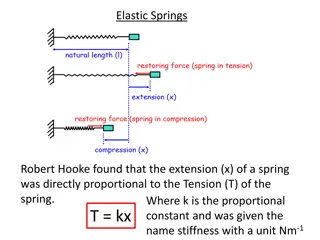
LECTURE 21 By D. V. Ramana Reddy Assistant Professor Department of Mechanical Engineering
Lecture 22 Bending stresses in beams TOPICS : Sectional Modulus Bending stress problems
Sectional Modulus Section modulus is defined as the ratio of moment of Inertia about the centroidal axis/neutral axis of a beam subjected to bending to the distance of outermost layer/fibre/filament from the centroidal axis. It is denoted by Z and unit is mm3 I y = Z M I My I M M Z = = = =I b M= Z b y y b Higher the Z value for a section, the higher the BM which it can withstand for a given maximum stress
Sectional Modulus for Rectangular section 3 bd 3 2 2 d I y bd bd 12 /2 d = = = = Z 12 6
Sectional Modulus for Rectangular section 3 3 BD bd I y 12 12 = = Z / 2 D 3 3 3 3 2 D BD bd BD bd = = ( ) Z 12 12 6 D
Sectional Modulus for Circular section 4 D 4 3 2 D D/2 I y D D 64 /2 D = = = = Z 64 32
Sectional Modulus for Hollow Circular section 4 4 D d 4 4 2 D I y D d 64 64 = = = Z /2 64 D 4 4 D d = Z 32 D
Case Study 1 A cantilever of length 2m fails when a load of 2kN is applied at the free end. If the section of the beam is 40x60 mm2. Find the stress at the failure. M I M y 2kN = = b b y I 60 2m 40 M I M y M I M Z M I E R = = = = b = = b b y I y y
2 2 40 60 6 bd = = = = = 3 3 24000 /12, /2 Z mm I bd y d 6 Maximum Bending Moment= w L M=2000 2000=4 10 N 6 mm M Z = b 6 4 10 24000 = = 2 166.67 / N mm b
Case Study 2 A 250mm depth and 150mm width rectangular beam is subjected to maximum bending moment of 750kN-m. Determine: (a) Maximum stress in the beam (b) If the value of E for the beam material is 200 GPa, Find out the radius of curvature for that portion of the beam where bending is maximum. (c) The value of longitudinal stress at a distance of 65 mm from the top surface of the bam. 750kN-m 250 150
M I M y M I M Z = = = = b b y I y 150 250 2 2 bd = = = = = 6 3 3 1.5625 10 /12, /2 Z mm I bd y d 6 6 6 750 10 1.5625 10 = = 2 480 / N mm M I E R I E b = = 6 R M 3 3 150 250 12 bd = = = 8 4 1.953 10 I mm 12
2 10 8 5 1.953 10 = = 52.08 R m 6 750 10 65 60 250 150 6 750 10 1.953 10 60 M y = = = 2 230.4 / N mm b 8 I
Case study 3 A circular beam of 120mm diameter is simply supported over a span of 10m and carries a uniformly distributed load of 1000 N/m. Find the Maximum bending stress produced. 120mm 1000 N/m A B L = 10m
d = = 3 4 Sectional Modulus Z= [ , ] d I d y 32 64 2 = = 3 3 5 3 Z= 120 1.6964 10 d mm 32 32 2 wl 8 Bending Moment= Maximum 2 1000 10 8 = = = 3 6 12.5 10 12.5 10 M N m N mm
6 12.5 10 1.6964 10 M Z = = = = 2 73.68 / MaximumBendingStress N mm b 5 120mm 73.68 Mpa(Compressive) 73.68 Mpa(Tensile)
Case study 4 An I section as shown in fig. is simply supported over a span of 12m. If the maximum permissible bending stress is 80MPa.what concentrated load can be carried at a distance of 4m from right support. 11.5 W in N C 225 7.5 A B 4m L =12 m 11.5 100
R andR calculation R R R w A b + = w = A B 12 w = 8 0 B = 2 /3 w R and R A B 3 8 8 w w = = 1000 Bending Moment at C N m N mm 3 3 3 3 100 225 12 (100 7.5)(225 23) 12 Moment of Inertia I= = 4 31386647.45 I mm
M I y = y = = 112.5 , 80 mm MPa 8000 w 80 3 = 31386647.45 8369.77 w = 112.5 N
Case study 5 A square beam 20x20mm2 in section and 2m long is simply supported at the ends. The beam fails when a point load of 400N is applied at the centre of the beam. What uniformly distributed load per meter length will break a cantilever of the same material 40x60mm2 and 3m long. Hint: Comparison of simply supported beam with point load and cantilever beam with .
400N C wl 4 Maximum Bending Moment= A B L/2 L =2 m W N/m A B 2 wl 2 Maximum Bending Moment= L = 3m *****Equate the Maximum stress in two cases****
M I M y I M I M Z = = = = b b y y 2 2 20 20 6 400 2000 4 2 10 1333.33 bd = = = = y d = 3 3 1333.333 /12, /2 Z mm I bd 6 WL = = 2 10 = N mm 5 M 4 5 = = 2 150.00 / N mm b
M I M y M I M Z = = = = b b y I y 2 2 40 60 6 w = bd = = = = = 3 3 24000 /12, /2 Z mm I bd y d 6 wL 2 2 3 = = 4.5 N-m w M 2 2 w 3 4.5 10 24000 800 / N m = = 2 150.00 / N mm b = w