Understanding Probability: Key Concepts and Rules
Probability is the proportion of successes in a long series of repetitions. Important ideas include the 0-1 Rule, the 100% Rule, the Complement Rule, the Addition Rule, and the Multiplication Rule. These rules govern the calculation of probabilities in various scenarios. Learn the fundamental principles of probability to enhance your understanding of chance events and outcomes.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
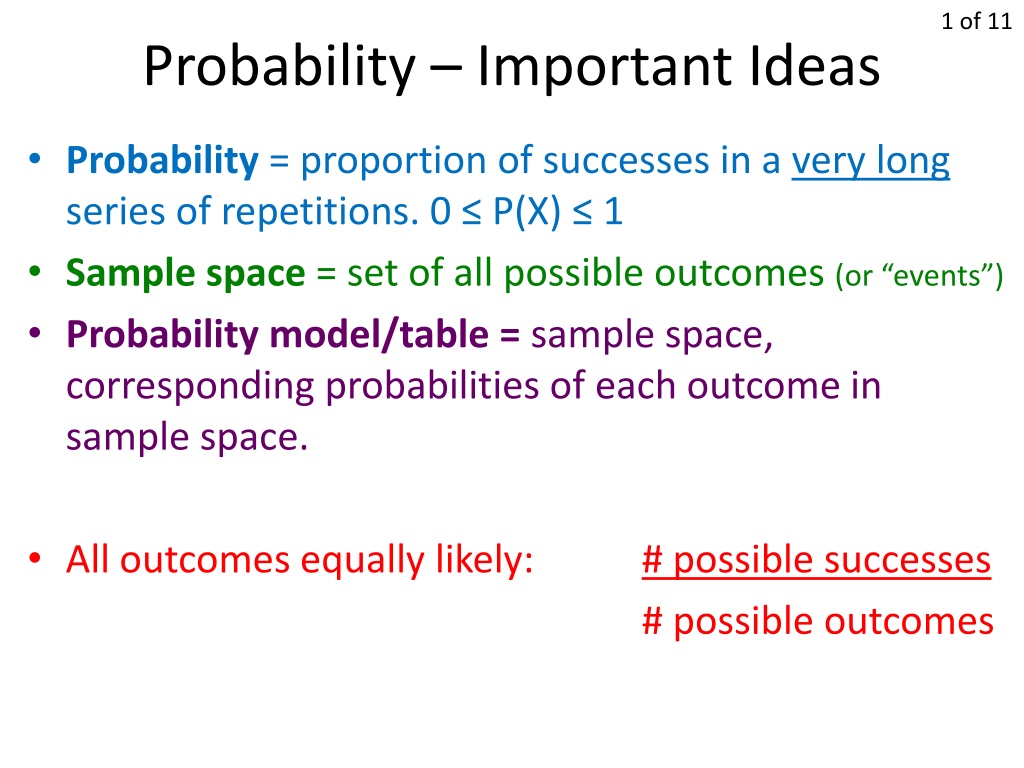
1 of 11 Probability Important Ideas Probability = proportion of successes in a very long series of repetitions. 0 P(X) 1 Sample space = set of all possible outcomes (or events ) Probability model/table = sample space, corresponding probabilities of each outcome in sample space. All outcomes equally likely: # possible successes # possible outcomes
2 of 11 #1: The 0-1 Rule Any probability is a number between 0 and 1. 0 never occurs 1 always occurs
3 of 11 #2: The 100% Rule The sum of the probabilities of all possible outcomes must equal 1. Example: If P(black/brown hair) = .91, and P(blonde hair) = .045, what is P(other colored hair)?
4 of 11 #3: The Complement Rule The probability that A does not occur is 1 P(A). Written as A or AC Called the complement of A Example: If P(A) is the probability of rolling two dice and getting a sum of at least 4, what is P(AC)?
5 of 11 #4: The Addition Rule The probability of Event A or Event B is the sum of their probabilities, minus their overlap . P(A or B) = P(A) + P(B) P(A and B). Can be simplified to P(A) + P(B) if disjoint Examples: What is the probability of drawing a Jack or a diamond from a standard deck? What is the probability of flipping Heads on a coin or rolling a Four on a die?
6 of 11 #5: The Multiplication Rule Probability of Event A and Event B is product of the probability of A and the probability of B given that A has happened. Example: What is the probability of getting an Ace and then a Jack?
7 of 11 Liar, liar, pants on fire What percent (%) of the fired students do you think are really thieves? 10% of employees are stealing. Lie detector, 80% accuracy. Says: Honest 0.8 Honest 0.9 Says: Thief! 0.2 Says: Thief! 0.8 Thief 0.1 Says: Honest 0.2
7 of 11 Liar, liar, pants on fire What percent (%) of the fired students do you think are really thieves? 10% of employees are stealing. Lie detector, 80% accuracy. . 0 72 Says: Honest 0.8 Honest . 0 . 0 18 08 0.9 Says: Thief! 0.2 Says: Thief! 0.8 Thief 0.1 . 0 02 Says: Honest 0.2
8 of 11 Conditional Probability # possible successes # possible outcomes Example (from previous activity) Given that the lie detector has gone off , what are the chances it s right, and you ve caught a thief?
9 of 11 Independent vs. Disjoint, 1 of 2 Two events are independentif whether one occurs does not change the probability that the other occurs. P(A) = P(A|B)
10 of 11 Example of Independence Below are the number of earned degrees in the US for the 2005-2006 academic year. Bachelor s Master s Professional Doctorate TOTAL Female 784 276 39 20 1119 Male 559 197 44 25 825 TOTAL 1343 473 83 45 1944 1. Are the events male and doctorate independent? 2. Are the events female and Bachelor s degree independent?
11 of 11 Independent vs. Disjoint, 2 of 2 Two events are disjoint ( mutually exclusive ) if they have no outcomes in common. Yes: drawing a Jack or a Queen No: drawing a Jack or a heart
FRQ Athletes are often tested for use of performance-enhancing drugs. Drug tests aren t perfect they sometimes say that an athlete took a banned substance when that isn t the case (a false positive ). Other times, the test concludes that the athlete is clean when he or she actually took a banned substance (a false negative ). For one commonly used drug test, the probability of a false negative is 0.03, and the probability of a false positive is 0.09. A random survey (World Anti-Doping Agency, 12/22/2008) of 21,849 professional athletes from 1968-2008 found that 0.49% of professional athletes use/used banned substances. 1. Make a tree diagram of this situation. 2. What is the probability that a randomly selected athlete will test positive for performance-enhancing drugs?
0.00475 Test positive for drugs Drugs Test negative for drugs ( false negative ) 0.00015 Test positive for drugs ( false positive ) 0.0896 No drugs Test negative 0.9055 0.09435