Understanding Permutations in Mathematics

Slide Note

Permutations in mathematics involve arranging items in a specific order, considering factors like repetitions and groupings. Different scenarios are explored, such as selecting friends for lunch, arranging letters in words, and creating lists of favorite books. Formulas and strategies for calculating permutations are illustrated through examples with detailed explanations and factorial notation.

cha_h Follow

Uploaded on Sep 17, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

E N D

Presentation Transcript

11.1B Permutations Fundamental Counting Principle If a task is made up of multiple operations (activities or stages that are independent of each other) the total number of possibilities for the multi-step task is given by mxnxpx . . . arrangements of repeats A permutation determines the number of ways to list or arrange items. A permutation is a linear arrangement of a set of objects for which the order of the objects is important. Items may be identical or may repeat. Many permutations question may be computed by using FCP. A combination determines the number of ways to group items. Items must be unique and may not be repeated. 1

Select the appropriate strategy for calculating each situation choosing 3 friends to go out to lunch determine arrangements of letters arranging vases on a shelf picking your favourite books to make a Top 10 List picking and ranking your favourite books on a Top 10 List Math 30-1 2

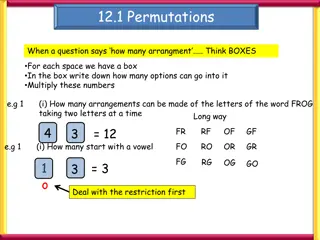
In how many ways can the letters of the word be arranged?7! = 5040 1 HARMONY The number of permutations of n different objects taken all at a time is n! In how many ways can the letters of the word HARMONY be arranged if the arrangement must start with H? 1x6! = 720 1 In how many ways can the letters of the word be arranged if the letters H and A must be together (adjacent)? HARMONY 2!x6! = 1440 1 Math 30-1 3

Finding the Number of Permutations How many three-letter arrangements can be formed from the letters of the word DINOSAUR? ____ x ____ x ____ 1st 2nd 3rd 8 There would be 336 ways. 7 6 336 represents the number of permutations of eight objects taken three at a time. 8! 5! 8! = Write 8 x 7 x 6 in factorial notation. =8P3 (8 3)! 8P3 is read as eight permute three . In general, if we have n objects but only want to select r objects at a time, the number of different linear arrangements is: n! What restrictions are on n and r? nPr= (n r)! 4

Finding the Number of Permutations The number of permutations of n objects taken n at a time is: nPn = n! n! 3! nPr= = 3P3= = 3P3 = 3! (n r)! (3 3)! 3P3= =3! 0! From these two results, we see that 0! = 1. To have meaning when r = n, we define 0! = 1. Math 30-1 5

Finding the Number of Permutations 1. Using the letters of the word PRODUCT, how many four-letter arrangements can be made? 7P4= 840 7 6 5 4 There would be 840 arrangements. 2. Determine the number of different arrangements of four or more letters that can be formed with the letters of the word LOGARITHM if each letter is not used more than once. 9P4+ 9P5 + 9P6 + 9P7 + 9P8 + 9P9 = 985 824 There would be 985 824 arrangements. 3. True or False , rP r n = , rP r n , rP r n n n n Math 30-1 6

Finding the Number of Permutations 4. How many six-letter words can be formed from the letters of TRAVEL? (Note that letters cannot be repeated) a) If any of the six letters can be used. 6P6 = 6! = 720 b) If the first letter must be L : 1 x5P5 = 1 x 5! = 120 c) If the second and fourth letters are vowels: 2 x 1 x 4! = 48 4 3 2 1 ___ ___ ___ ___ ___ ___ V 2 1 V d) If the A and the V must be adjacent: (Treat the AV as one group - this grouping can be arranged 2! ways.) Math 30-1 7 5! x 2! = 240

Finding the Number of Permutations.. with Repeats How many arrangements are there of four letters from the word PREACHING? 9! 9! 5! 9P4 = 3024 = = ( ) 9 4 ! How many distinct arrangements of BRAINS are there keeping the vowels together? 5! x 2! = 240 Math 30-1 8

Finding the Number of Permutations (Grouping) A bookshelf contains five different algebra books and seven different physics books. How many different ways can these books be arranged if the algebra books are to be kept together? Total number of arrangements = 8! x 5! = 4 838 400 A student has 4 different biology books, 5 different chemistry books, and 6 different math books. In how many ways can the books be arranged so that the biology books stand together, the chemistry books stand together and the math books stand together. 4! x 5! x 6! x 3! = 12 441 600 There are 12 441 600 ways of arranging the books. Math 30-1 9

Determine the number of different arrangements using all the letters of the word ACCESSES that begin with at least two S s 3 2 5 5! 3!2!2! 3 2 1 5! 3!2!2! + = 180 Math 30-1 10

Permutations Two objects are selected from a group and arranged in order. If there are 90 possible arrangements, how many objects are there? n! (n 2)!= = 90 n(n 1)(n 2)! (n 2)! n(n - 1) = 90 n2 - n = 90 n2 - n - 90 = 0 (n - 10)(n + 9) = 0 n + 9 = 0 n = -9 nP2 = 90 = = 90 n N n - 10 = 0 n = 10 OR Therefore n = 10. 11

Solving Equations Involving Permutations. Solve nP2 = 30 algebraically. n! (n 2)!= = 30 n(n 1)(n 2)! (n 2)! = = 30 n(n - 1) = 30 n2 - n = 30 n2 - n - 30 = 0 (n - 6)(n + 5) = 0 n = 6 or n = -5 Therefore, n = 6. 12

Solving Equations Involving Permutations. Solve nP4 = 8(n-1P3) algebraically. 8(n 1)! (n 1) 3 ( ( n! (n 4)!= = n! (n 4)!= =8(n 1)! ) )! n 4 ( ( ) )! (n 4)!= =8(n 1)! n! ( ( ) )! (n 4)! ) )! n 4 n 4 ( ( n!= = 8(n 1)! n(n 1)!= =8(n 1)! n = 8 Math 30-1 13