Permutations Explained with Examples in Mathematics
Learn how to calculate arrangements and codes using permutations by understanding the principles of combinations and restrictions. Explore various scenarios such as letter arrangements, digit codes, and number formations along with specific conditions like starting with vowels or meeting certain criteria.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
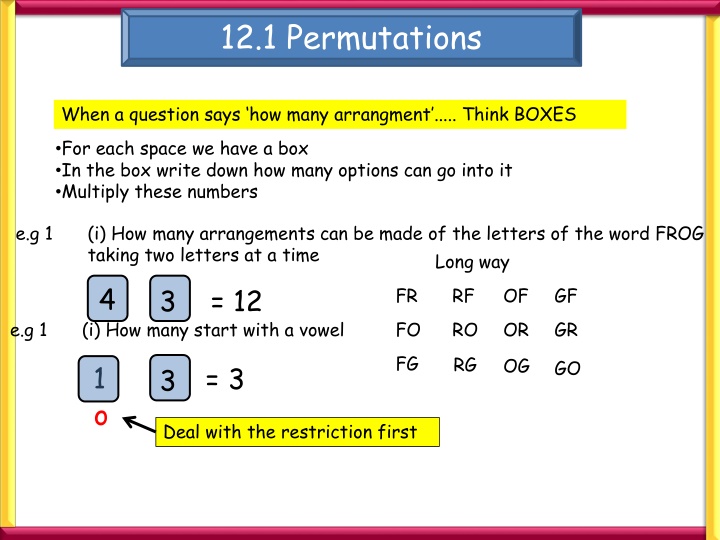
12.1 Permutations When a question says how many arrangment ..... Think BOXES For each space we have a box In the box write down how many options can go into it Multiply these numbers e.g 1 (i) How many arrangements can be made of the letters of the word FROG taking two letters at a time 4 3 = 12 FO FG 1 3 = 3 o Deal with the restriction first Long way FR RF RO OF OR GF GR e.g 1 (i) How many start with a vowel RG OG GO
e,g, 2 The digits 0,1,2,3,4,5 are to form a three digit code. A code cannot begin with a 0 and no digit can be repeated. How many codes can be formed ? 4 = 100 5 5 No o Deal with the restriction first
e.g 3 (i) How many different numbers can be formed from the digits 2, 3, 4,5, 6, if each of the digits can be used only once in each number? (ii) How many of the numbers are less than 400? (iii) How many of the numbers are divisible by 5? (iv) How many of the numbers are less than 400 and divisible by 5?
e.g 3 (i) How many different numbers can be formed from the digits 2, 3, 4, 5, 6, if each of the digits can be used only once in each number? (ii) How many of the numbers are less than 400? (iii) How many of the numbers are divisible by 5? (iv) How many of the numbers are less than 400 and divisible by 5? 2 1 4 (i) = 120 3 5 2 1 4 (ii)2 = 48 3 2,3 Deal with the restriction first 1 3 1 (iii)4 2 = 24 5 1 5 1 3 (iv)2 = 12 2 2,3
e.g 4 A code consists of a four-digit number which is formed from the digits 3 to 9 inclusive. No digit can occur more than once in the code. (i) Write down the smallest possible four-digit code. (ii) How many different codes are possible? (iii) How many of the four-digit codes are greater than 6000? (iv) How many of the four-digit codes are divisible by 2?c
e.g 4 A code consists of a four-digit number which is formed from the digits 3 to 9 inclusive. No digit can occur more than once in the code. (i) Write down the smallest possible four-digit code. (ii) How many different codes are possible? (iii) How many of the four-digit codes are greater than 6000? (iv) How many of the four-digit codes are divisible by 2?c (i) 345 6 4 6 (ii)7 5 = 840 4 6 (iii)4 6,7,8,9 5 = 480 Deal with the restriction first 3 = 360 4,6,8 5 (iv)6 4
e.g 5 (i) there are no restrictions on the order of seating (ii) there must be a boy at the beginning of the row (iii) there must be a boy at the beginning of the row and a boy at the end of the row (iv) the two girls must be seated beside each other? Three boys and two girls are seated in a row as a group. In how many different ways can the group be seated if
e.g 5 (i) there are no restrictions on the order of seating (ii) there must be a boy at the beginning of the row (iii) there must be a boy at the beginning of the row and a boy at the end of the row (iv) the two girls must be seated beside each other? 5 4 3 Three boys and two girls are seated in a row as a group. In how many different ways can the group be seated if 2 1 (i) = 120 to be seated together take one of the seats away 2 1 4 (ii)3 Boy = 72 3 Deal with the restriction first Then multiply the answer by 2 as the girls could swap places with each other 1 2 3 (iii)3 = 120 2 Boy Boy = 24 = 24 x 2 = 48 1 3 (iv)4 2
