Understanding Logarithms: Laws, Examples, and Applications
Logarithms are important mathematical tools used in various calculations. This content covers the basics of logarithms, logarithmic laws, solving logarithmic equations, and practical examples. Explore the concept of logarithms and their applications in different scenarios.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
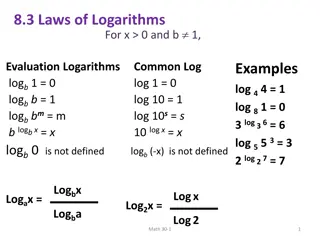
Logarithms the base 10 raised to the power 2 gives 100 102 = 100 2 is the power which the base 10 must be raised to, to give 100 the power = logarithm 2 is the logarithm to the base 10 of 100 Logarithm is the number which we need to raise a base to for a given answer to what power must I raise 2 to give an answer of 64? ans = 6 written as log2 64 = 6
to what power must I raise 2 to give an answer of 64? ans = 6 written as log2 64 = 6 to what power must I raise 5 to give an answer of 625? ans = 4 written as log5 625 = 4 to what power must I raise 9 to give an answer of 3? ans = 1 2 written as log9 3 = 1 2 loga n = p ap = n baseanswer = number inside
1. loga m + loga n = loga mn 2. loga m - loga n = loga m 3. n loga m = loga mn n 4. logn m = loga m loga n change of base law N.B. The log of a negative is impossible to find
Law 2 loga m - loga n = loga m Let loga m = p & loga n = q ap = m ap = aq ap - q =m n m n m n Proofs: Law 1 loga m + loga n = loga m n Let loga m = p & loga n = q ap = m ap . aq = m . n ap + q = m . n loga m . n = p + q loga m n = loga m + loga n n aq = n aq = n m n loga = p - q baseanswer = number inside loga = loga m - loga n
Law 3 n loga m = loga mn Law 4 logn m = loga m loga n Let loga m = p Let logn m = p ap = m We need mn take logs of both sides m np = ( )n = m ( )n ap apn = mn loga mn = pn loga mn = (loga m) n loga mn = n loga m loga np = loga m p loga n = loga m p = loga m baseanswer = number inside loga n logn m = loga m loga n
e.g.1 log4 64 = x e.g.3 log4 (5x + 6) = 2 baseanswer = number inside 42= 5x + 6 16= 5x + 6 10= 5x 10 5 2 = x 4x= 64 4x= 43 x = 3 = x e.g.2 log2 x = 5 25= x x = 32
e.g.4 log3 (2x - 4) = 1 + log3 (4x + 8) log3 (2x 4) log3 (4x + 8) 2 4 log 4 8 x + 2 4 3 4 8 x + 3(4 8) 2 4 x x + = For an unknown power always take logs of both sides e.g.5 6n = 3200 = 1 = x 1 log10 6n = log10 3200 3 n log10 6 = log10 3200 x = 1 n = log10 3200 log10 6 n = 4.5045 12x + 24 = 2x - 4 10x = -28 x = -28 x = -2.8 10
Calculations using log10 logn m = logam change of base law loga n log10 1000 = 3 as 103 = 1000 If we want log2 32 = log10 32 log10 2 e.g.6 log2 55 = log10 x log10 55 log10 2 = log10 x baseanswer = number inside 5.78 = log10 x 105.78 = x x = 604449