Understanding Crystal Field Theory and Atomic Basis Sets
Explore the principles behind crystal field theory, including the concept of effective Hamiltonians to mimic covalent bonding in solids. Learn how to expand potentials on spherical harmonics and understand the selection rules and symmetry considerations in this theoretical framework.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Crystal Field expanded on renormalized spherical Harmonics Clm Maurits W. Haverkort Institute for theoretical physics Heidelberg University M.W.Haverkort@thphys.uni-heidelberg.de
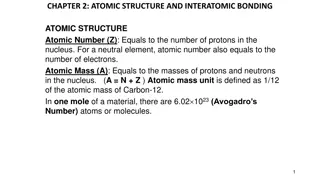
From potentials to matrix elements of the Hamiltonian Crystal-field theory Approximate the solid by a single atom in an effective potential And matrix elements of the Hamiltonian: !!!! WARNING !!!! Crystal-field potentials do not exist. They are effective Hamiltonians introduced to mimic covalent bonding. (crystals bind due to a gain in kinetic energy and a smaller loss in potential energy, i.e. the contribution of the crystal- field potential to binding is negative) Covalent bonds are stronger than ionic bonds
From potentials to matrix elements of the Hamiltonian Atomic basis sets: (also Gaussian, muffin-tin orbitals, ) Expand potential on spherical harmonics.
From potentials to matrix elements of the Hamiltonian Atomic basis sets: (also Gaussian, muffin-tin orbitals, )
Not all values of Akm are allowed (many are zero) Selection rules on angular momentum conservation Hamiltonian is Hermitian
Not all values of Akm are allowed (many are zero) Symmetry related: For all rotations of the point-group Ci we have Cubic: (Oh) eg d t2g
Not all values of Akm are allowed (many are zero) Tetragonal: (D4h) a1g eg b1g d eg t2g b2g