Symmetrical Components and Fortescue's Theorem
An unbalanced set of N related phasors can be resolved into N systems of phasors known as symmetrical components. For a three-phase system, these components include Positive Sequence, Negative Sequence, and Zero Sequence. These components help simplify complex scenarios in electrical systems.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
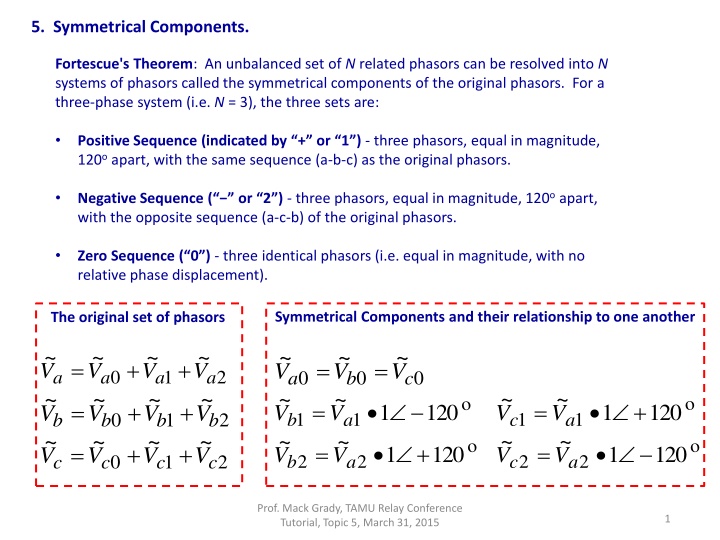
5. Symmetrical Components. Fortescue's Theorem: An unbalanced set of N related phasors can be resolved into N systems of phasors called the symmetrical components of the original phasors. For a three-phase system (i.e. N = 3), the three sets are: Positive Sequence (indicated by + or 1 ) - three phasors, equal in magnitude, 120o apart, with the same sequence (a-b-c) as the original phasors. Negative Sequence ( or 2 ) - three phasors, equal in magnitude, 120o apart, with the opposite sequence (a-c-b) of the original phasors. Zero Sequence ( 0 ) - three identical phasors (i.e. equal in magnitude, with no relative phase displacement). Symmetrical Components and their relationship to one another The original set of phasors ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V = + + = = 0 1 2 a a a a 0 0 0 a b c ~ V ~ V ~ V ~ V ~ V ~ V ~ V ~ V o o = + = + + = 1 120 1 120 1 1 1 1 c a 0 1 2 b a b b b b o o = + + = + = 1 120 1 120 2 2 2 2 0 1 2 b a c a c c c c Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 1
5. Symmetrical Components, cont. The symmetrical components of all a-b-c voltages are usually written in terms of the symmetrical components of phase a by defining complex number a as 120 1 + = a o o 2 120 1 0 4 2 1 = + = a o o 3 0 1 0 6 3 1 = + = a , o ~ ~ ~ , , V V V Substituting into the previous equations for yields ~ ~ ~ ~ a a a a V V V V + + = 2 0 a a a b V a V a V V + + = ~ ~ ~ ~ a a a c V a V a V V + + = a b ~ V c ~ V 1 1 a 1 a 0 1 2 0 a a ~ V ~ V ~ ~ ~ ~ = 2 1 1 2 1 b a ~ V ~ V 2 1 a a 2 2 c a 0 1 2 1 1 1 which in simplified form is 1 1 a 1 a ~ V ~ V ~ V ~ V 1 1 2 = = 1 , , T T = 1 = T a a 2 1 , T 012 012 abc abc 3 2 2 1 a a 1 a a Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 2
5. Symmetrical Components, cont. Why do all this? Answer: to simplify most situations. ~ V 0 ~ V = 1 , V ~ T 012 abc a ~ ~ 1 1 1 V ~ a a 1 = 2 1 V ~ a V ~ 1 a b 3 2 2 1 V a a V 2 a c For balanced set, have only positive-sequence ~ V ~ V + + + + 2 2 1 1 a 1 a 1 1 a 1 a 1 a 1 1 0 0 a a a a ~ V ~ V ~ V ~ V 0 a a 1 ~ V ~ V ~ V = = = + + = + + = = 2 2 2 2 3 3 3 3 1 1 1 1 3 1 a a a a a a a a a 1 a a a 3 3 3 3 3 ~ V ~ V + + + + 2 2 4 2 2 1 1 1 1 0 0 a a a a a a a a a a 2 a a Swap phases b and c (negative rotation), have only negative-sequence ~ V ~ V + + + + 2 2 1 1 a 1 a 1 1 a 1 a 1 a 1 1 0 0 a a a a ~ V ~ V ~ V ~ V 0 a a 1 ~ V ~ V ~ V = = = + + = + 1 + = = 2 2 2 4 2 1 1 1 1 0 0 a a a a a a a a a 1 a a a 3 3 3 3 3 ~ V ~ V + + + + 2 2 2 2 3 3 1 1 1 1 1 3 1 a a a a a a a a 2 a a Common mode (a, b, c identical), have only zero-sequence ~ V ~ V 1 1 a 1 a 1 1 a 1 a 1 3 3 1 ~ V ~ V ~ V 0 a a 1 ~ V ~ V ~ V = = = + + = = 2 2 2 1 1 1 1 0 0 a a a a a 1 a a a 3 3 3 3 ~ V ~ V + + 2 2 2 1 1 1 1 0 0 a a a a a a 2 a a Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 3
5. Symmetrical Components, cont. Zero-Sequence (i.e., Common Mode) Transformer Models, again Zero-sequence current can flow ONLY when there is a ground path R + jX Grounded Wye - Grounded Wye Grounded Wye - Delta R + jX Grounded Wye - Ungrounded Wye R + jX Ungrounded Wye - Delta R + jX Delta - Delta R + jX Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 4
5. Symmetrical Components, cont. Three-phase circuits can be solved in full a-b-c, or in using 0-1-2 sequence components. For a balanced circuit, solve it in positive-sequence because negative- and zero- sequences are zero, so that the positive-sequence solution IS the phase A solution. Hence, the one-line diagram. Consider the following a-b-c equations for a symmetric network. Symmetric means that self-impedances S for each phase are equal, and mutual-impedances M between phases are equal. ~ ~ V ~ S M M I ~ a a ~ ~ = abc V Z I = V ~ M S M I ~ abc abc b b V M M S I c c Converting to 0-1-2 ~ V ~ = abc T Z I T 012 012 ~ V ~ = 1 1 T ~ V T T ~ I Z I T 012 012 abc = = 1 , Z Z T Z T where 012 012 012 012 abc Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 5
5. Symmetrical Components, cont. = 1 Z T Z T 012 abc 1 1 a 1 a 1 1 a 1 a S M M 1 = 2 2 1 1 M S M 3 2 2 1 1 a a M M S a a + + S + S 2 2 2 1 1 a 1 a S M S M S M 1 = 2 2 ( ) ( S ) 1 S M a M a M 3 2 2 ( ) ( ) 1 S M a S M a M a a + 0 ( 3 2 ) 0 0 S M 1 = 0 ( 3 ) 0 S M 3 0 ( 3 ) S M This is huge! If network impedances are balanced, then a coupled a-b-c network can be solved using three simple uncoupled 0-1-2 networks. + ( 2 ) 0 0 S M = 0 0 ( ) 0 S M 0 ( ) S M Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 6
5. Symmetrical Components. Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 7
5. Symmetrical Components. Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 8
5. Symmetrical Components. Prof. Mack Grady, TAMU Relay Conference Tutorial, Topic 5, March 31, 2015 9