Lami's Theorem in Physics
LEMI’s Theorem
Lami’s Theorem Statement:
Lami's theorem is an equation
relating the magnitudes of
three coplanar, concurrent and
non-collinear forces, which
keeps an object in static
equilibrium, with the angles
directly opposite to the
corresponding forces
.
Derivation:
•
Now, let’s see how the
theorem’s equation is
derived.
•
Let FA, FB, and FC be the
forces acting at a point. As
per the statement of the
theorem, we take the sum
of all forces acting at a
given point which will be
zero.
Let 45 degrees be the angle made by the strings with the
signboard having a mass of 6 kg, then what is the value of the
tension T in both the strings?
Given, m = 6 kg, g = 9.8 m/s², 𝜃 = 45 degrees
Using the derived formula from example 1, we get,
T = mg / 2cosθ
i.e. T = 6 x 9.8 / 2cos45 = 41.6 N
So, the tension in both the strings to hold the signboard exactly
horizontal is 41.6 N.
Examples:
2: Consider an advertisement board hangs with the
help of two strings making an equal angle with the
ceiling. Calculate the tension in both the strings in
this case?
Resolving the forces we will apply
the required theorem to get the
value of tension in both the strings.
Here, the weight of the signboard is
in a downward direction, and the
other force is the tension generated
by the signboard in both the
strings. In this case, the tension T in
both the strings will be the same as
the angle made by them with the
signboard is equal.
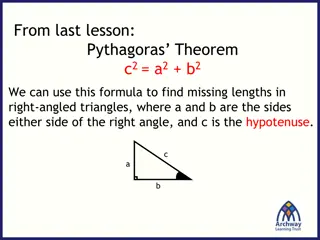
Lami's Theorem is an equation that explains how the magnitudes of forces acting on a point keep an object in equilibrium. This theorem relates the forces with corresponding angles and is derived by understanding the sum of forces acting on a point. By utilizing complementary angles and the sine rule, the theorem is applied to determine tension forces in various scenarios. Examples show how Lami's Theorem is used to calculate tensions in different setups involving forces and angles.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Lamis Theorem Statement: Lami's theorem is an equation relating the magnitudes of three coplanar, concurrent and non-collinear keeps an object equilibrium, with the angles directly opposite corresponding forces. forces, which static in to the
Derivation: Now, let s see how the theorem s equation is derived. Let FA, FB, and FC be the forces acting at a point. As per the statement of the theorem, we take the sum of all forces acting at a given point which will be zero.
i.e. FA + FB + FC = 0 We write angles in terms of complementary angles and use triangle law of vector addition. Then, by applying the sine rule we get, ? ? ? sin(180 ?)= sin(180 ?)= sin(180 ?) Then, ? ? ? ????= ????= ???? Hence, it is clearly seen that by applying sine rule to complementary angles we arrive at the required result for Lami s theorem.
Examples: Let 45 degrees be the angle made by the strings with the signboard having a mass of 6 kg, then what is the value of the tension T in both the strings? Given, m = 6 kg, g = 9.8 m/s , ? = 45 degrees Using the derived formula from example 1, we get, T = mg / 2cos i.e. T = 6 x 9.8 / 2cos45 = 41.6 N So, the tension in both the strings to hold the signboard exactly horizontal is 41.6 N.
2: Consider an advertisement board hangs with the help of two strings making an equal angle with the ceiling. Calculate the tension in both the strings in this case? Resolving the forces we will apply the required theorem to get the value of tension in both the strings. Here, the weight of the signboard is in a downward direction, and the other force is the tension generated by the signboard in both the strings. In this case, the tension T in both the strings will be the same as the angle made by them with the signboard is equal.
Above figure represents the free body diagram of the signboard. Applying the Lami s Theorem we get, ? ? ?? sin(180 ?)= sin(180 ?)= sin(2?) Since sin (180 ) = sin and sin (2 ) = 2sin cos So, we get, final tension force in the string T as,
? ?? ????= 2???? ???? I.e. T = mg / 2cos The similar concept and equations can be applied for a boy playing on a swing, and we arrive at the same result.
