Steps to Determine Function Continuity

Steps to determine if a function is continuous involve checking if the function is defined at a point, evaluating the limit from both sides, and verifying the limits are the same. Horizontal and vertical tangent lines can be found by analyzing derivatives, points of zero slopes, and unbounded functions. The content provides detailed explanations and examples on identifying horizontal and vertical tangent lines in functions.

• Function continuity
• Tangent lines
• Derivatives
• Limits
• Unbounded functions

kund_rt Follow

Uploaded on Feb 23, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

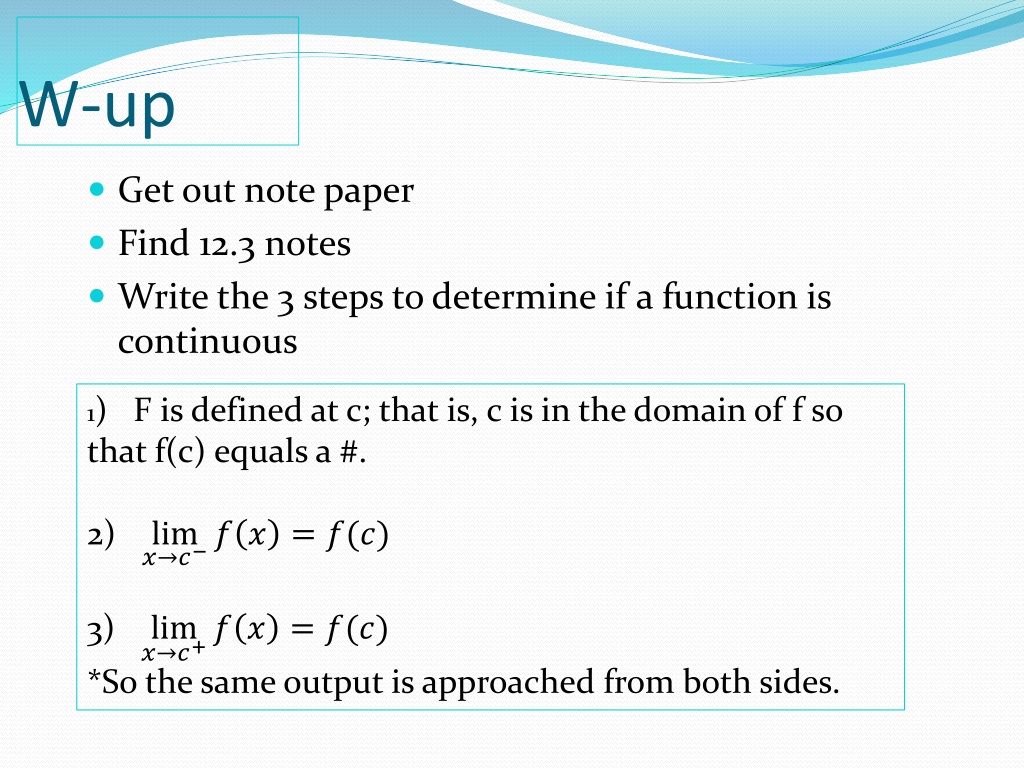
1. W-up Get out note paper Find 12.3 notes Write the 3 steps to determine if a function is continuous 1) F is defined at c; that is, c is in the domain of f so that f(c) equals a #. 2) lim ? ? ? ? = ?(?) 3) lim ? ?+? ? = ?(?) *So the same output is approached from both sides.

2. Find horizontal and vertical tangent lines Discuss the graph of a function f where the derivative of f does not exist

3. Horizontal tangent line If function is differentiable at point c, horizontal tangent line occurs when f (c) = 0 Ex1: At what points is the tangent line of f(x)=x3 + 3x2 24x horizontal? Find derivative: f (x) = 3x2 + 6x 24 Set f (x) = 0 and solve 0 = 3x2 + 6x 24 factor Plug x values into ORIGINAL equation to find the y-value where tangent line is horizontal f(-4) = (-4)3 + 3(-4)2 24(-4) = 80 f(2) = (2)3 + 3(2)2 24(2) = -28 3(x2 + 2x 8) = 0 (x + 4 ) (x 2) = 0 x = -4 and x = 2 Horizontal tangent lines will occur at (-4,80) and (2, -28)

4. Conditions for Vertical Tangent Lines If a vertical tangent line is present at a point (c, f(c)) on a continuous function then 1) x = c is in the domain 2) f (x) must be unbounded at x = c Unbounded will be where the denominator of the derivative = 0

5. Ex1: find horizontal and vertical tangent lines of f(x) = -3x2 + 12x Step 1 state domain all real numbers Step 2- find derivative f (x) = -6x + 12 To find horizontal set f (x) = 0 and solve 0 = -6x + 12 x = 2 Plug 2 into original to find y f(2) = -3(2)2 + 12(2) = 12 Horizontal tangent line at (2, 12) When will there be a vertical tangent line? NO VERTICAL TAN LINE there is no denominator (no unbounded points)

6. Ex2: find horizontal and vertical tangent lines of 2/3 ( ) 1 x x = f x 1) FIND domain ? 1 2) find the derivative good times ( 3 ) 2 1 x x 2 3 x ( ) ( 2/3 1/3 2/3 x 1 x x x Get common denominator 1/3 = = '( ) x f ( ) ) 2 2 ) 1/3 1 x 1 ( 1 2 1 x 1 3 x x 2 x = = '( ) x f ( ) 2 ( ) x 1 2 3 x 1/3 3 1 x x = ( ) 2 1

7. 2 x 2/3 x x = '( ) x f = ( ) f x ( ) 2 1/3 3 1 x x 1 Find horizontal tangent line set derivative = 0 and solve = To find vertical tangent line set denominator of derivative = 0 and solve ( 0 3 x = 2 x 0 ) ( ) 2 2 1/3 3 1 x x 1/3 1 x Set each factor = 0 and solve = A fraction will be zero when numerator is zero x=-2 Find y by plugging x into original equation 2 ( 2) 2 1 ( ) 2 = 1/3 0 3 1 0 x and x x = 0 and x = 1 Throw out 1 NOT IN DOMAIN Find y by plugging x into original equation 2/3 0 (0 1 0 2/3 = 0.53 f = ) 0 f Horizontal tangent line at (-2,.53) Vertical tangent line a (0,0)

8. Continuity and Differentiability If c is a number in the domain of f and f is differentiable at the number c, then f is continuous at c If it is not continuous it is not differentiable. Converse is not always true if a graph is continuous at c, it may not be differentiable

9. = 2/5 ( ) f x x Given: a) determine if it is continuous at x = 0 = = 2/5 (0) 0 0 f Is it defined find left and right side limits = = = = 2/5 2/5 lim x 0 lim x 0 x x + 0 0 Yes continuous all equal zero B) does f (0) exist? Plug in zero 2 '(0) 5 (0) 2 5 2 x = = 3 /5 '( ) f x x 3/5 5 2 0 Will this be a VERTICAL tangent line or NO tangent line? = = . . . f D N E 3/5

10. 6 + 1 x if x = = ( ) f x 1 at x Given: a) determine if it is continuous at x = 1 2 5 1 x if x Is it defined f(1) = 12 + 5 = 6 find left and right side limits lim 1 5 6 x = + = = = 2 lim x 6(1) 6 + 1 1 Yes continuous all equal six

11. B) does f(c) exist? GRAPH 6 + 1 x if x = = ( ) f x 1 at x 2 5 1 x if x f(x) = x2+5; x> 1 f(x) = 6x; x< 1 1 0 6 0 1 2 6 9 -1 -6 3 14 f (1) does not exist, there are two different slopes at 1 Find the derivative of each part f (x) = 6 f (1) = 6 f (x) = 2x f (1) = 2

12. Homework 14.1 # 1 33 odds Don t forget to graph # 27-33