Right Triangles and Pythagorean Theorem
A right triangle has a 90-degree angle with sides known as legs and a hypotenuse opposite the right angle. The Pythagorean theorem states that in a right triangle, the square of the hypotenuse equals the sum of the squares of the other two sides, forming Pythagorean triples. Learn how to find hypotenuse or leg lengths using this theorem and explore practical examples.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
WHAT IS A RIGHT TRIANGLE? (altitude) side hypotenuse right angle side (base) It is a triangle which has an angle that is It is a triangle which has an angle that is 90 90 degrees. degrees. The two sides that make up the right The two sides that make up the right angle are called angle are called legs legs (base & altitude). The side opposite to the right angle is the The side opposite to the right angle is the hypotenuse hypotenuse. . (base & altitude).
WHICH GOLD PLATE WOULD YOU CHOOSE? Suppose I were to beat gold into place as shown below, what option would you Suppose I were to beat gold into place as shown below, what option would you choose? choose? a. a. Plate c Plate c2 2 b. b. Plate a Plate a2 2 and plate b and plate b2 2? ?
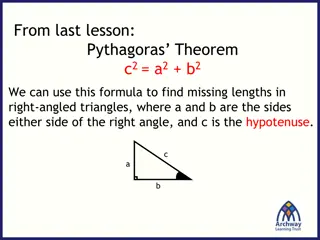
PYTHAGOREAN THEOREM In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides. c a b c2 = a2 + b2
USING THE PYTHAGOREAN THEOREM A Pythagorean triple is a set of three positive integers a, b, and c that satisfy the equation c2 = a2 + b2 For example, the integers 3, 4 and 5 form a Pythagorean Triple because 52 = 32 + 42.
EX. 1: FINDING THE LENGTH OF THE HYPOTENUSE. Find the length of the hypotenuse of the right triangle. Tell whether the sides lengths form a Pythagorean Triple. 12 5 x
EX. 2: FINDING THE LENGTH OF A LEG Find the length of the leg of the right triangle. x 7 14
FIND THE LENGTH OF THE HYPOTENUSE IF A = 12 AND B = 16. 12 122 2 + 16 + 162 2 = c 144 + 256 = c 144 + 256 = c2 2 400 = c 400 = c2 2 = c2 2 Take the square root of both sides. Take the square root of both sides. = 2 20 = c 20 = c 400 c
FIND THE LENGTH OF THE HYPOTENUSE IF A = 5 AND B = 7. 52 + 72 = C2 25 + 49 = C2 74 = C2 TAKE THE SQUARE ROOT OF BOTH SIDES. 74 = 2 c 8.60 = C 8.60 = C
AREA OF TRIANGLES h b The area of a triangle is half of the area of a parallelogram. A = bh
WHATS THE AREA? 4 cm 8 cm 16 cm2 of 8 x 4 =
WHATS THE AREA? 8 cm 32 cm2 8 cm
WHATS THE AREA? 8 cm 12 cm2 3 cm
PROOF: 1. Area of the square = (a + b)2 = a2 + b2 + 2ab 2. The area of the square is also the area of the pink square as well as the 4 blue triangles. i. ii. Area of the pink square = c2 Area of the blue triangles = ab Area of 4 triangles = 4( ab) = 2ab iii. Therefore total area = c2 + 2ab 1. (iii) = (1) Therefore , a2 + b2 + 2ab = c2 + 2ab or a2 + b2 = c2 Hence the proof!
SIDE LENGTHS OF SPECIAL RIGHT TRIANGLES Right triangles whose angle measures are 45 -45 -90 or 30 -60 -90 are called special right triangles. The theorems that describe these relationships of side lengths of each of these special right triangles follow.
45-45-90 TRIANGLE THEOREM In a 45 -45 -90 triangle, the hypotenuse is 2 times as long as each leg. 45 2x x 45 x Hypotenuse = 2 leg
30-60-90 TRIANGLE THEOREM In a 30 -60 -90 triangle, the hypotenuse is twice as long as the shorter leg, and the longer leg is 3 times as long as the shorter leg. 60 2x x 30 3x Hypotenuse = 2 shorter leg Longer leg = 3 shorter leg
EX. 1: FINDING THE HYPOTENUSE IN A 45-45-90 TRIANGLE Hypotenuse = 2 leg Hypotenuse = 2 leg 3 3 x = 2 3 x = 2 3 x = 3 2 x = 3 2 45 x 45 -45 -90 Triangle Theorem Substitute values Simplify
EX. 2: FINDING A LEG IN A 45-45-90 TRIANGLE Find the value of x. Find the value of x. Because the triangle is an isosceles right triangle, its base angles are congruent. The triangle is a 45 -45 - 90 right triangle, so the length of the hypotenuse is 2 times the length x of a leg. 5 x x
EX. 3: FINDING SIDE LENGTHS IN A 30-60-90 TRIANGLE Find the values of s and t. Find the values of s and t. Because the triangle is a 30 -60 -90 triangle, the longer leg is 3 times the length s of the shorter leg. 60 t s 30 5
CONVERSE OF THE PYTHAGOREAN THEOREM: If If ABC is a triangle with sides of lengths a, b, and c ABC is a triangle with sides of lengths a, b, and c such that a such that a2 2 + b + b2 2 = c = c2 2; then ABC is a right angled ; then ABC is a right angled triangle with the right angle opposite to the side c. triangle with the right angle opposite to the side c.
EXERCISE Determine if the following lengths can be the sides of a right triangle: Determine if the following lengths can be the sides of a right triangle: 1. 1. 51, 68, 85 51, 68, 85 2. 2. 2, 3, 13 2, 3, 13 3. 3. 3, 4, 7 3, 4, 7