Relative Motion
The concept of relative motion in physics through examples like a bee flying in the wind and projectiles launched from a moving vehicle. Learn important facts and relationships, and solve 1D and 2D relative motion problems. Discover how to calculate velocities, durations, and paths in different frames of reference.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
A Bee in the Wind A bee wishes to fly 100m south to a flower, then return to its hive. The first time it completes this trip, the air is calm. The second time there is a breeze blowing north to south. The third time there is a breeze blowing south to north, the same speed as the previous breeze. The bee always flies at the same airspeed (speed relative to the air). Rank the durations for these three trips.
Projectiles to Relative Motion A cannon that launches projectiles 50.0m/s horizontally is mounted in the back of a truck, facing out the rear of the truck. The truck is driven forward at 50.0m/s. Describe the path of the ball as seen from: the truck the ground (earth)
Projectiles to Relative Motion https://www.youtube.com/watch?v=qQVDAM zo4mE Now what do you think? Need some more help? Yes, you do! http://www.youtube.com/watch?v=v0a7vVB8 tq8
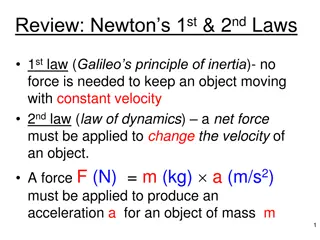
Important facts and relationships in relative motion VA/B= the velocity of object A as seen from reference frame B VA/B= - VB/A VA/C= VA/B+ VB/C All kinematics equations are valid, as long as values are input for ONE frame of reference, in ONE dimension at a time Only frames with zero acceleration should be used to describe motion VA/A= 0
Relative Motion 1D example A motorboat that can move at 10 m/s relative to the water. How long will it take the boat to travel from Philadelphia to New Hope, via the Delaware River. Relative to land, this is a 30.0 km trip. The river flows at 2.5m/s.
Relative Motion in 2-Dimensions Essential Questions How do you cross a current in the least time? How do you solve 2D relative motion problems quantitatively?
Relative Motion in 2-Dimensions Recommended Behavior VA/C= VA/B+ VB/C is still valid! Vectors can only be added or subtracted in COMPONENT form, or with a scale drawing. Remember to use variables from ONE REFERENCE FRAME and in only ONE DIRECTION when solving any kinematics equation (ex: x = vit + at2). In other words, don t mix up directions A current can be crossed in the shortest time by aiming perpendicular to the current (and allowing it to carry you down-stream
2-D Example On a particular spring day, a steady wind blows from the west to the east at 10.0 m/s. (That is called a westerly wind.) An RC airplane with an airspeed of 40.0 m/s is aimed due north while it is in flight. What is the plane s velocity (you need magnitude and direction) as seen from the ground?
2- D Example Part Two For the same airplane and wind conditions, determine the direction the plane must be aimed, and duration required for the plane to travel 1.00 km due north (relative to the ground).
A 100m wide river flows south at 3.0 m/s. A boat can go at 5.00 m/s relative to the water. What direction should the boat aim to travel from a point on the east bank to a position directly west on the west bank? How long does it take to cross?
A cop traveling 30 m/s north sees a car which appears to be traveling at 50 m/s 37 south of east. What is the car s velocity relative to the ground?
Relative Motion Examples 1D: P 88 # 70, 71 2D: P88,89 # 77, 79 (a&b), 73