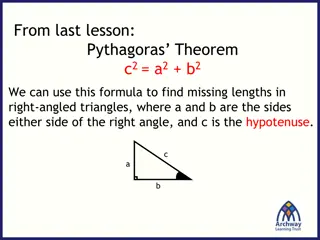
Pythagoras’ Theorem
The Pythagoras Theorem explains the relationship between the sides of a right-angled triangle. Discover the history, visualization, and application of this fundamental mathematical concept through a detailed exploration. Uncover how to use Pythagoras Theorem to calculate the length of the hypotenuse in various triangles, enhancing your geometry skills. Dive into the essence of this influential theorem that has transcended time and civilizations, shaping modern mathematics.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
22 February 2025 Pythagoras Theorem LO: To use Pythagoras theorem in right-angled triangles. www.mathssupport.org
Right-angled triangles A right-angled triangle contains a right angle. The other two sides are called legs of the triangle. The longest side opposite the right angle is called the hypotenuse. legs The right angle always points to the hypotenuse www.mathssupport.org
The history of Pythagoras Theorem Pythagoras Theorem concerns the relationship between the sides of a right- angled triangle. The theorem is named after the Greek mathematician and philosopher, Pythagoras of Samos, who was born in about 581 BC on the island of Samos, just off the coast of Turkey. www.mathssupport.org
The history of Pythagoras Theorem Although the Theorem is named after Pythagoras, the result was known to many ancient civilizations including the Babylonians, Egyptians and Chinese, at least 1000 years before Pythagoras was born. www.mathssupport.org
Pythagoras Theorem Pythagoras Theorem states that the square formed on the hypotenuse of a right-angled triangle has the same area as the sum of the areas of the squares formed on the other two sides. www.mathssupport.org
Pythagoras Theorem If we label the length of the sides of a right-angled triangle a, bandcas follows, then the area of the largest square is c cor c2. The areas of the smaller squares are a2andb2. c2 c a2 a We can write Pythagoras Theorem as b b2 c2 =a2 + b2 www.mathssupport.org
The Theorem of Pythagoras: A Visual Demonstration Perigal s Dissection In a right-angled triangle, the square on the hypotenuse is equal to the sum of the squares on the other two sides. Draw 2 lines through the centre of the middle square, parallel to the sides of the large square c2 This divides the middle square into 4 congruent quadrilaterals a2 a c Henry Perigal (1801 1898) These quadrilaterals, plus the small square fit exactly into the large square b b2 c2 =a2 + b2 www.mathssupport.org
Finding the length of the hypotenuse Use Pythagoras Theorem to calculate the length of sidea. a 5 cm 12 cm The missing side labelled a is the hypotenuse Using Pythagoras Theorem, a2 =52 + 122 a2 =25 + 144 a2 =169 a = 169 a =13 cm www.mathssupport.org
Finding the length of the shorter sides C Use Pythagoras Theorem to calculate the length of side labelled x. 4 cm x A 5 cm B The side labelled x is a shorter side Using Pythagoras Theorem 42 + b2 =52 b2 =52 42 b2 =25 16 b2 =9 = 3 b = ? We reject the negative value as the length must be positive b = 3 www.mathssupport.org
Finding the length of the hypotenuse Q Use Pythagoras Theorem to calculate the length of side PR. Give the answer in the simplest surd form. 4 m 2 m P R Using Pythagoras Theorem PR2 =PQ2 + QR2 Substituting the values we have been given, PR 2 =22 + 42 PR 2 =4 + 16 PR 2 =20 PR = ?? PR =? ? The solution ? ? is the surd in the simplest form. www.mathssupport.org
Finding the length of the shorter sides A Use Pythagoras Theorem to calculate the length of side AC. Give the answer in the simplest surd form. 5 cm C B 8 cm Using Pythagoras Theorem AB2 + AC2 =BC2 Substituting the values we have been given, 52 + AC2 =82 AC 2 =82 52 AC 2 =39 AC = ?? The solution ?? is the surd and cannot be simplified. www.mathssupport.org
Applying Pythagoras Theorem twice A Use Pythagoras Theorem to calculate the length of the unknowns. 5 cm B y x 1 cm C D 6 cm Using Pythagoras Theorem AB2 + BC2 =AC2 In ABC the hypotenuse AC isxcm long, 52 + 12 =AC2 25 + 1 =AC2 AC2 =26 AC = ?? = x In ACD the hypotenuse DC is 6 cm long, AD2 + ( 26)2 =62 AD2 + 26 =36 AD2 =36 26 AD2 =10 AD = ??= y www.mathssupport.org
Applying Pythagoras Theorem twice Sometimes we have to apply Pythagoras Theorem twice to find a required length. For example, Find the length of side a. To solve this problem we need to find the length of the other missing side which we can call b. b2 = 182 (4 + 9)2 b2 = 324 169 b2 = 155 Now, a2 = b2 + 42 a2 = 155 + 16 a2 = 171 a = 3 ?? 18 cm b a 4 cm 9 cm www.mathssupport.org
Thank you for using resources from A close up of a cage Description automatically generated For more resources visit our website https://www.mathssupport.org If you have a special request, drop us an email info@mathssupport.org Get 20% off in your next purchase from our website, just use this code when checkout: MSUPPORT_20 www.mathssupport.org