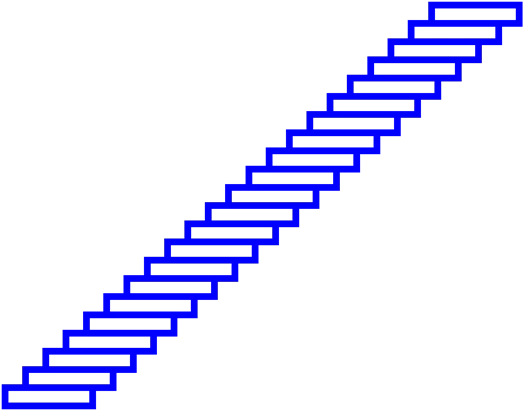
Leaning Tower Stacking Challenge: How Far Can You Go?

Discover the fascinating challenge of stacking 2-inch wide blocks of a leaning tower as far over as possible without them falling. Follow the journey through images as the center of mass shifts and find out where to strategically place the next block to maintain stability.

• Leaning Tower
• Stacking Challenge
• Center of Mass
• Block Stacking
• Physics

sica_lly Follow

Uploaded on Oct 05, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

1. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. With out falling How far over can you shift it go over? More of the output? 1

2. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. But if I keep adding blocks it will falling Is this a proof that it is not possible? Try again More of the output: I have produced the first i-1 objects. 2

3. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. it will falling Where should we put the next block? More of the output: I have produced the last i-1 objects. Extra info: Center of mass is within bottom block Leaning distance so far 3

4. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. it will falling Where should we put the next block? More of the output: I have produced the last i-1 objects. Extra info: Center of mass is within bottom block Leaning distance so far 4

5. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. No progress Where should we put the next block? More of the output: I have produced the last i-1 objects. Extra info: Center of mass is within bottom block Leaning distance so far 5

6. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. With the center of mass of the above blocks teetering on the edge of supporting block. Where should we put the next block? More of the output: I have produced the last i-1 objects. Extra info: Center of mass is within bottom block Leaning distance so far 6

7. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. Where is the new center of mass? More of the output: I have produced the last i-1 objects. Extra info: Center of mass is within bottom block Leaning distance so far 7

8. Leaning Tower Mass = m1 Mass = m2 Where is the new center of mass? Center of mass = r1 Center of mass = r2 Center of mass m = + r m r R 1 m 1 2 2 + m (Ignoring veridical) 1 2 8

9. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. mass2 mass1 To make the math easier, lets call this zero. Where is the new center of mass? Mass = m1= 1 Center of mass = r1 = 1 Mass = m2= i-1 Center of mass = r2 = 0 Center of mass m = Center of mass is 1/i from right edge of ith block. + r m r R 1 m 1 1 2 2 + ( m i ( 1 + 2 ) 1 1 0 1 = = + 1 ) 1 i i 9

10. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. 1 1/i Add the next block. = 1 = = ln( ) d n i 1 .. i n n= de More of the output: I have produced the last i-1 objects. Extra info: Center of mass is 1/i from right edge of ith block. Leaning distance so far? 10

11. Leaning Tower Input: Lots of 2in wide blocks Output: Stack them leaning as far over as possible. 1 1/i = 1 = = ln( ) d n i 1 .. i n = d 2 n 11