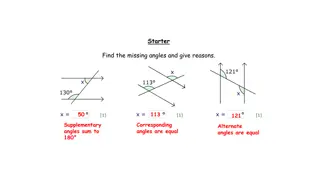
Internal Tangents of Circles Construction
When constructing internal tangents between two circles, it is important to follow certain steps such as adding the smaller circle's radius to the bigger circle, joining points, bisecting lines, and creating semi-circles. This process ensures the tangent is formed correctly.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Tangents Tangents An interior tangent of two circles
Draw on the whiteboard what you think an Draw on the whiteboard what you think an INTERNAL tangent would look like INTERNAL tangent would look like KEYWORDS KEYWORDS
Take the radius of the smaller circle KEYWORDS: KEYWORDS: Internal Internal Radius Radius
Add the smaller radius to the bigger circle = = KEYWORDS: KEYWORDS: Internal Internal Radius Radius
For internal tangents always add the small circles radius to the bigger circles radius. This is so you are now constructing a tangent from a point to a circle = = KEYWORDS: KEYWORDS: Internal Internal Radius Radius
Now that the smaller circle has been reduced to a point, join that point to the centre of the new circle (same) KEYWORDS: KEYWORDS: Internal Internal Radius Radius
Bisect line that joins the point to the circle KEYWORDS: KEYWORDS: Internal Internal Radius Radius Bisect Bisect
Bisect line that joins the point to the circle KEYWORDS: KEYWORDS: Internal Internal Radius Radius Bisect Bisect
Bisect line that joins the point to the circle KEYWORDS: KEYWORDS: Internal Internal Radius Radius Bisect Bisect
Construct a semi-circle with the diameter from the centre of the circle to the point P KEYWORDS: KEYWORDS: Internal Internal Radius Radius Bisect Bisect Diameter Diameter
Always construct a triangle in the semi-circle with the diameter as the base and apex where the circle intersects the semi-circle. KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Bisect Bisect Diameter Diameter
Always construct a triangle in the semi-circle with the diameter as the base and apex where the circle intersects the semi-circle. KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Bisect Bisect Diameter Diameter
Transfer the tangent in parallel KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Diameter Diameter
Find other POC by constructing a line at 90 degrees to the tangent through the centre NORMAL TANGENT NORMAL KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Point of Contact Point of Contact Diameter Diameter
Point of Contact NORMAL TANGENT NORMAL Point of Contact KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Point of Contact Point of Contact Diameter Diameter
Point of Contact NORMAL TANGENT NORMAL Point of Contact KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Point of Contact Point of Contact Diameter Diameter
Lets try a question Let s try a question Example 2: pg 188 Understanding Technical Graphics Construct an internal tangent between these 2 circles KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Point of Contact Point of Contact Diameter Diameter
Lets try a question Let s try a question Question 2: pg 189 Understanding Technical Graphics KEYWORDS: KEYWORDS: Internal Base Base Internal Apex Apex Radius Radius Parallel Parallel Bisect Bisect Point of Contact Point of Contact Diameter Diameter
Lets make definitions for our keywords Let s make definitions for our keywords Internal Internal Radius Radius Bisect Bisect Diameter Diameter Base Base Apex Apex Parallel Parallel Point of Contact Point of Contact