Geometry Fundamentals Overview
Explore key concepts in geometry such as points, lines, planes, segments, and measurements. Learn about postulates, coordinates in 2D and 3D, and calculating distances between points.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
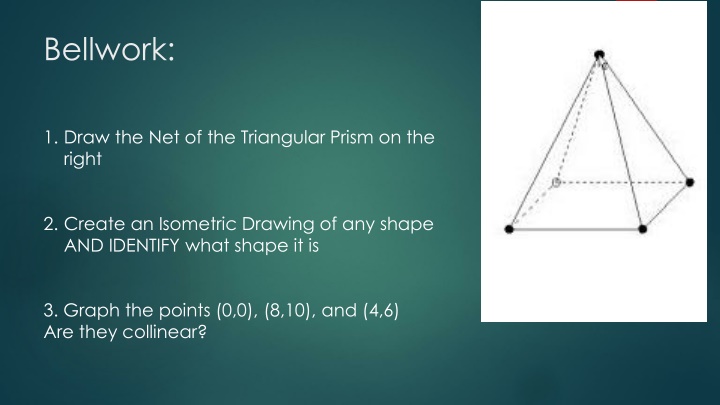
Bellwork: 1. Draw the Net of the Triangular Prism on the right 2. Create an Isometric Drawing of any shape AND IDENTIFY what shape it is 3. Graph the points (0,0), (8,10), and (4,6) Are they collinear?
1.2-Points, Lines, and Planes(Cont.)
How to name things Lines? Points? Planes? Segments? Rays?
Postulates Postulate 1-1 Through any two points there is exactly one line Postulate 1-2 If two distinct lines intersect, they do so in exactly one point What about Planes?
What is the intersection of planes BFE and FEH? In general, what is the intersection of two planes?
Postulate 1-4 Through any three non-collinear points there is exactly one plane
1.3 Measuring Segments What is a Coordinate? How do we find the distance between two points? How do we cut a segment in half?
Measuring Segment Lengths To find the distance between two coordinates (in 1D) find the absolute value of the difference in coordinates
What is the distance between point A and B? A B
Postulate 1-6 If A,B, and C are collinear points, and B is between A and C, then AB+BC=AC
Using Postulate 1-6 What is the length of AC?
If AC=232, find AB and BC 5x+3 10x+4
Definition Two objects are said to be congruent when they are similar. If two figures are congruent, they will appear the same when superimposed Two line segments are congruent when they have the same length
Problem 3 page 22 Are ?? and ?? congruent? A B C D
Definition: The Midpoint of a segment is the point that divides the segment into two congruent segments. A point, line, ray, or other segment that intersects a segment at its midpoint is said to bisect the segment. That point, line, ray, or segment is called a segment bisector
Using the Midpoint: Problem 4 page 23 If we know that Q is the midpoint of ??, how can we find PQ, QR, and PR?
Homework: Section 1.1: 17, 20, 22 Section 1.2: 8, 9, 12, 15, 33, 53, 65, 75, 78 Section 1.3 : 9, 13, 17, 20, 35, 37
Online textbook: pearsonrealize.com Username: student_ID@edu.leonschools.net. This is actually the students' computer login information as well. Password is: Lcs6789! Students can change passwords when they are in the system.