Exploring Sample Space Diagrams for Probability Analysis
Understand sample space diagrams through visual representations of dice games and coin toss scenarios to determine probabilities of different outcomes. Explore fair game scenarios and practice calculating probabilities based on various game rules involving dice and coins.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
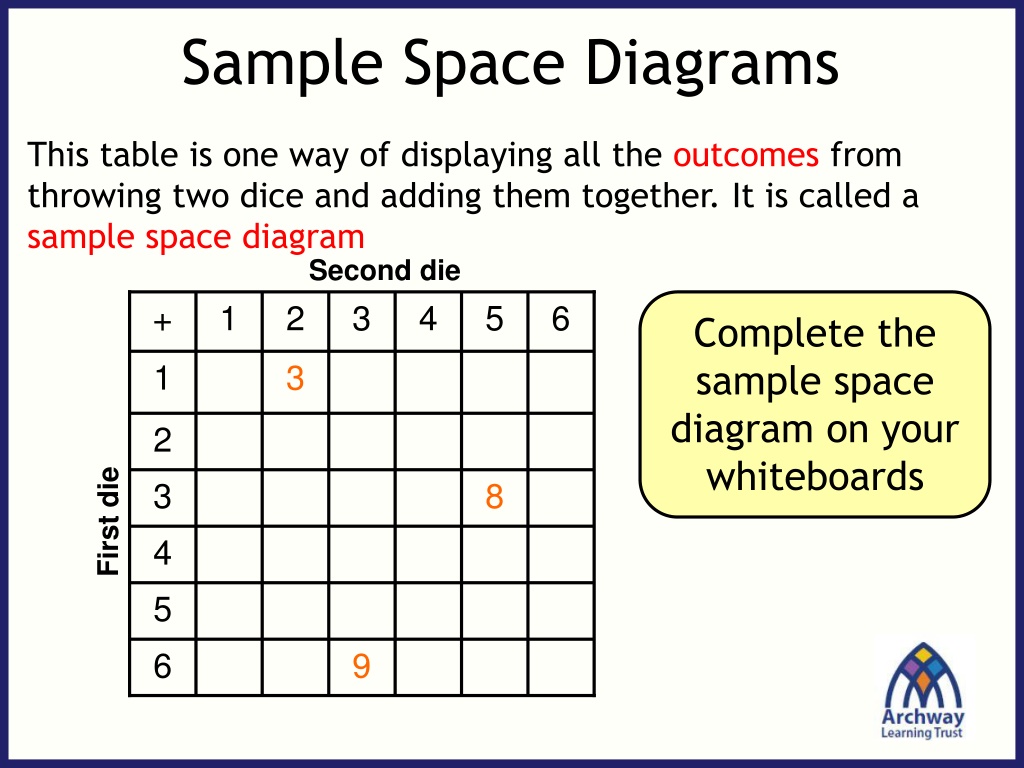
Sample Space Diagrams This table is one way of displaying all the outcomes from throwing two dice and adding them together. It is called a sample space diagram Second die + 1 2 3 4 5 6 Complete the sample space diagram on your whiteboards 1 3 2 First die 3 8 4 5 6 9
Sample Space Diagrams The sample space diagram can be used to find probabilities. 1) What is the probability of getting a total more than 5? 2) What is the probability of getting a total less than 10? 3) What is the probability of getting a total that is a square number? 4) What is the probability of getting an even total? 5) What is the probability of getting an odd total? 6) What is the probability of getting a total less than 13? Second die + 1 2 3 4 5 6 1 2 2 3 3 4 4 5 5 6 6 7 7 2 3 3 4 4 5 5 6 6 7 7 8 8 First die 3 4 4 5 5 6 6 7 7 8 8 9 9 4 5 5 6 6 7 7 8 8 9 9 10 10 5 6 6 7 7 8 8 9 9 10 10 11 11 6 7 7 8 8 9 9 10 10 11 11 12 12
Two Dice Games: Use sample space diagrams to show how you decide whether the game is fair. (1) (2) two dice are rolled and the numbers on each dice are subtracted (smaller from larger) two dice are rolled and the numbers on each dice are added if the total is prime: Adrian wins if the total is composite (not prime): Bella wins if the difference is 1 or 2: Anton wins if the difference is 0, 3, 4 or 5: Brisa wins is this a fair game? is this a fair game? (3) (4) two dice are rolled and the numbers on each are multiplied and then this is divided by 3 the score is the remainder two dice are rolled and the numbers on each are multiplied and then this is divided by 5 the score is the remainder e.g. for (2, 5) you get 10 3 the remainder is 1 e.g. for (1, 3) you get 3 5 the remainder is 3 if the remainder is 0: Alwyn wins if the remainder is 1 or 2: Bart wins if the remainder is 0 or 1: Alice wins if the remainder is 2, 3 or 4: Bram wins is this a fair game? is this a fair game?
Sample Space Diagrams A coin and a ten sided die are thrown and the outcomes recorded in the two-way table below. Dice + 1 2 3 4 5 6 7 8 9 10 Coin H 2,H 7,H T 8,T Complete the sample space diagram on your whiteboards
Sample Space Diagrams A coin and a ten sided die are thrown and the outcomes recorded in the two-way table below. Die + 1 2 3 4 5 6 7 8 9 10 Coin H 1,H 2,H 3,H 4,H 5,H 6,H 7,H 8,H 9,H 10,H T 1,T 2,T 3,T 4,T 5,T 6,T 7,T 8,T 9,T 10,T 1) How many outcomes are there? 2) What is the probability of getting a head and an even number? 3) What is the probability of getting a tail and a square number?
Sample Space Diagrams Two four sided dice are thrown and the numbers added together. Complete the sample space diagram on your whiteboards What is the probability of getting: 1) a total more than 4? 2) a total less than 8? 3) a prime number total? 4) a total that is at least 3? 5) a total of 4 or 5? 6) the same number on both dice? 7) a lower number on the first dice? Second die + 1 2 3 4 1 2 3 4 5 First die 2 3 4 5 6 3 4 5 6 7 4 5 6 7 8
Sample Space Diagrams Second die + 1 2 3 4 Use the table to find the probability of getting a score of 3 or 4. 1 2 2 3 3 3 4 4 4 5 5 5 First die 2 3 3 3 4 4 4 5 5 5 6 6 6 The probability of getting a score of 3 or 4 can be written as P(3 or 4). 3 4 4 4 5 5 5 6 6 6 7 7 4 5 5 5 6 6 6 7 7 8 5 16 P(3 or 4) = 7 16 P(5 or 6) = 3 16 P(2 or 7) =