Electrostatic Properties of Polyelectrolyte Solutions for DNA Molecules
D
N
A
c
o
b
y
p
o
l
y
e
l
e
k
t
r
o
l
y
t
:
M
a
n
n
i
n
g
o
v
a
t
e
o
r
i
e
k
o
n
d
e
n
z
a
c
e
p
r
o
t
i
i
o
n
t
ů
Manning, G. S. (1978).
"The molecular theory of polyelectrolyte solutions with
aplications to the electrostatic properties of polynulceotides."
Quart. Rev. Biophys.
11:
179-246.
Prof. Jiří Kozelka, Biofyzikální Laboratoř, Ústav fyziky kondenzovaných
látek, PřF MU, Kotlářská 2,kozelka.jiri@gmail.com
F
5351
Základy molekulární biofyziky
Masarykova Univerzita
Podzimní semestr
2021
26.11.2021
DNA double helix
Double-stranded DNA can be considered as a linear long rod composed of segments, each
carrying one unit charge. The segments repel each other. The repulsion is diminished if
positively charged counter-ions associate with the DNA surface.
The distance
b
between segments harboring a unit charge
(1
q
e
=
1.60x10
-19
C
)
defines the
charge density
of the polymer.
For single-stranded DNA,
b = 3.4
Å
For double-stranded DNA,
b =1.7
Å
Before we continue, let us learn about two definitions:
Bjerrum* length
l
B
:
the distance between two elementary charges (
q
e
)
at which their repulsion energy is equal to the thermal energy
k
B
T
Manning** structural parameter
:
the ratio between
l
B
and
b
, that is,
the product of the Bjerrum length (a constant
[
Å
]
) and the linear
charge density (
[
Å
-1
]
).
It is thus a dimensionless parameter
proportional to the charge density
.
*
N
i
e
l
s
J
a
n
n
i
c
k
s
e
n
B
j
e
r
r
u
m
(
1
8
7
9
-
1
9
5
8
)
,
d
á
n
s
k
ý
c
h
e
m
i
k
*
*
G
e
r
a
l
d
S
.
M
a
n
n
i
n
g
,
p
r
o
f
e
s
s
o
r
e
m
e
r
i
t
u
s
,
R
u
t
g
e
r
s
U
n
i
v
e
r
s
i
t
y
,
N
e
w
J
e
r
s
e
y
,
U
S
A
At each segment, a
fraction
of positive counterions „condenses“ within a volume
V
P
G
/
=
0
1 equation
2 unknowns
Univalent salt in water
(e.g., NaCl)
DNA double helix
G
(
,
V
P
)
/
=
0
The charge of 1 segment is reduced to (-1+
)e
Gain in enthalpy
H, since repulsion between segments is diminished
Loss in entropy
S, since order increases
Nature will „choose“ such a
fraction, that
G =
H – T
S is minimal
q
net
q
net
q
net
q
net
q
net
q
net
q
net
q
net
Calculation of the electrostatic repulsion term
G
el
Phosphate N°:
1
2
3……………..s………………………n
p
Each pair contributes:
b
.
.
|i-j| = s: number of
pairs = n
p
-s
|i-j| > s: interaction negligible
if n
p
>> s
G
ij
≈ 0
Charge:
r
D
= „Debye screening length“
proportional to c
-1/2
c: concentration of univalent salt
q
net
= q
e
(
1-
)
Simplifying the sum over pairwise electrostatic repulsion terms
Looks like a Taylor series
….
C
v
i
č
e
n
í
1
:
o
d
v
o
ď
t
e
T
a
y
l
o
r
ů
v
r
o
z
v
o
j
p
r
o
f
u
n
k
c
i
f
(
x
)
=
l
n
(
1
+
x
)
(
v
y
ž
a
d
o
v
á
n
o
j
e
ř
e
š
e
n
í
,
n
i
k
o
l
i
v
j
e
n
v
ý
s
l
e
d
e
k
)
T
a
y
l
o
r
ů
v
r
o
z
v
o
j
:
with a=0:
with
series converges
for x
<0
List of Taylor series of some common functions
http://en.wikipedia.org/wiki/Taylor_series
Simplifying the sum over pairwise electrostatic repulsion terms
=
C
v
i
č
e
n
í
3
:
z
j
e
d
n
o
d
u
š
t
e
(
r
D
>
>
b
)
Simplifying the sum over pairwise electrostatic repulsion terms
Sum has become a simple
logarithmic term!
Cvičení 4:
Vypo
čtěte
pro dvoj
šroubovicovou DNA (b
= 1.7
Å)
při
25 °C
k = 1.38x10
-23
J.K
-1
T
= 298 K
k
e
= 9.0x10
9
Nm
2
C
-2
=
78
q
e
= 1.60x10
-19
C
= 4.2 (single-stranded DNA:
= 2.1)
A
fraction
of positive counterions „condenses“ within a volume
V
P
Loss in entropy
S, since order increases
DNA double helix
c
local
= n
P
(
n
P
V
P
)
-1
=
V
P
-1
c
bulk
= c = concentration [mol/L] of univalent salt
KNOWN
Let‘s minimize the total free energy with respect to
Mixing entropy term dependent on
V
p
UNKNOWN → HOPELESS CALCULATION???
V
P
[L/mol] = volume to which the
counterions/phosphate are confined
i.e., loss in entropy when the fraction
of counterions associates with each segment
Cvičení 5:
Vypo
čtěte první derivaci následují funkc
e
podle x :
Minimizing total free energy
From Debye-Hückel Theory of electrolytes
C
v
i
č
e
n
í
6
:
v
y
p
o
č
t
ě
t
e
d
e
r
i
v
a
c
i
.
N
a
z
n
a
č
t
e
p
o
s
t
u
p
v
ý
p
o
č
t
u
.
We want to know
at
the low concentration
limit,
c→0
→
Singular
terms containing
ln c
must vanish!
Now we have
got a second
equation
!
We want to know
at
the low concentration
limit,
c→0
Now we have
got a second
equation
!
no association of counterions
association of counterions
76% of the negative
charge of the phosphates
is neutralized by
condensed counterions!
→
Singular
terms containing
ln c
must vanish!
Now we can determine V
P
, the volume to which the
θ
counterions/nucleotide condense in double-stranded DNA
k = 1.38x10
-23
J.K
-1
= 8.85x10
-12
C
2
N
-1
m
-2
DNA in water at 25°C
k
e
= 9.0x10
9
Nm
2
C
-2
L
AV
= 6.02x10
23
mole
-1
= 4.2
q
e
= 1.60x10
-19
C
e = 2.718
=78
T
= 298 K
= 0.76
=
6.46x10
26
L
AV
-1
Å
3
/nucleotide = 1073 Å
3
/nucleotide
Counterion Condensation Theory: Manning, G. S. (1978)
Quart. Rev. Biophys.
11:
179-246
The volume to which the condensed univalent counter-cations
are confined, V
P
Per mole: 646 cm
3
Per nucleotide: 1073
Å
3
This corresponds to a layer of ~7 Å around the DNA double-helix
(
w
h
i
c
h
c
o
r
r
e
s
p
o
n
d
s
~
t
o
a
c
y
l
i
n
d
e
r
w
i
t
h
a
d
i
a
m
e
t
e
r
o
f
2
0
Å
)
E
x
p
e
r
i
m
e
n
t
a
l
a
n
d
t
h
e
o
r
e
t
i
c
a
l
r
e
s
u
l
t
s
s
u
p
p
o
r
t
i
n
g
t
h
e
C
o
u
n
t
e
r
i
o
n
C
o
n
d
e
n
s
a
t
i
o
n
T
h
e
o
r
y
1
.
T
i
t
r
a
t
i
o
n
o
f
C
a
r
b
o
x
y
m
e
t
h
y
l
c
e
l
l
u
l
o
s
e
(
C
M
C
)
w
i
t
h
d
i
f
f
e
r
e
n
t
h
y
d
r
o
x
i
d
e
s
m
o
n
i
t
o
r
e
d
b
y
u
l
t
r
a
s
o
n
i
c
a
b
s
o
r
p
t
i
o
n
CMC
T
i
t
r
a
t
i
o
n
w
i
t
h
X
O
H
;
X
=
N
a
,
K
,
L
i
,
N
(
C
H
3
)
4
…
…
.
Upon titration, the
COOH groups
are successively deprotonated, become
COO
-
,
and
the negative charge density increases. This modifies the structure of the water layer at
the surface of the polymer, which can be monitored by measuring the absorption of
ultrasound. If there is no association of cations with the polyanion, the structure of the
water layer should be independent of the cation.
<
1
:
n
o
d
i
f
f
e
r
e
n
c
e
b
e
t
w
e
e
n
t
h
e
v
a
r
i
o
u
s
h
y
d
r
o
x
i
d
e
s
n
o
a
s
s
o
c
i
a
t
i
o
n
b
e
t
w
e
e
n
t
h
e
c
o
u
n
t
e
r
i
o
n
s
a
n
d
t
h
e
p
o
l
y
m
e
r
>
1
:
g
r
e
a
t
d
i
f
f
e
r
e
n
c
e
s
b
e
t
w
e
e
n
t
h
e
v
a
r
i
o
u
s
h
y
d
r
o
x
i
d
e
s
a
s
s
o
c
i
a
t
i
o
n
b
e
t
w
e
e
n
t
h
e
c
o
u
n
t
e
r
i
o
n
s
a
n
d
t
h
e
p
o
l
y
m
e
r
,
a
s
p
r
e
d
i
c
t
e
d
b
y
t
h
e
M
a
n
n
i
n
g
t
h
e
o
r
y
R
.
Z
a
n
a
e
t
a
l
.
,
J
.
C
h
i
m
.
P
h
y
s
,
6
8
,
1
2
5
8
-
1
2
6
6
(
1
9
7
1
)
Radial distribution of Na
+
counterions around a DNA duplex 12 base-pairs long,
from a molecular dynamics simulation (Yang et al.,
J. Am. Chem. Soc.
1997,
119
, 59-69). The calculation predicts 82% of the phosphate charge to be neutralized,
with cations moving within a radius of 15
Å from the helix axis. Manning theory
predicts 76% of cations within 17 Å from the helix axis.
2
.
M
o
l
e
c
u
l
a
r
d
y
n
a
m
i
c
s
s
i
m
u
l
a
t
i
o
n
s
o
f
a
s
o
l
v
a
t
e
d
D
N
A
d
u
p
l
e
x
[
Å]
% phosphate
charge
In a diluted solution of counterions,
is concentration-independent!
Remember:
http://matematika-online-a.kvalitne.cz/derivace-funkce.htm
(
a
x
)
*
l
n
a
Double-stranded DNA molecules exhibit repulsion due to their linear charge distribution, which can be mitigated by positively charged counter-ions. The Bjerrum length and Manning structural parameter play crucial roles in defining charge density. Electrostatic repulsion terms and calculations involving univalent salt interactions in water further shed light on the complex nature of DNA behavior in solution.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
DNA coby polyelektrolyt: Manningova teorie kondenzace protiiont F5351 Z klady molekul rn biofyziky Masarykova Univerzita Podzimn semestr 2021 26.11.2021 Prof. Ji Kozelka, Biofyzik ln Laborato , stav fyziky kondenzovan ch l tek, P F MU, Kotl sk 2, kozelka.jiri@gmail.com Manning, G. S. (1978). "The molecular theory of polyelectrolyte solutions with aplications to the electrostatic properties of polynulceotides." Quart. Rev. Biophys. 11: 179-246.
Double-stranded DNA can be considered as a linear long rod composed of segments, each carrying one unit charge. The segments repel each other. The repulsion is diminished if positively charged counter-ions associate with the DNA surface. 1 segment: charge -1e DNA double helix _ _ _ _ _ __ _ _ _ _ _ b = 1.7 The distance b between segments harboring a unit charge (1 qe=1.60x10-19 C) defines the charge density of the polymer. For single-stranded DNA, b = 3.4 For double-stranded DNA, b =1.7
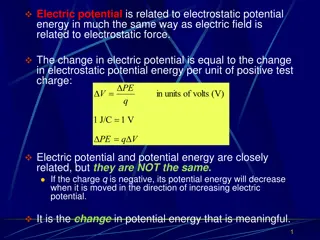
Before we continue, let us learn about two definitions: Bjerrum* length lB: the distance between two elementary charges (qe) at which their repulsion energy is equal to the thermal energy kBT 2 2 ??? =???? ??=???? ??? ???? *Niels Jannicksen Bjerrum (1879-1958), d nsk chemik Manning** structural parameter : the ratio between lBand b, that is, the product of the Bjerrum length (a constant [ ]) and the linear charge density ([ -1]). It is thus a dimensionless parameter proportional to the charge density. =?? ?= 2 ???? ????? ** Gerald S. Manning, professor emeritus, Rutgers University, New Jersey, USA
Univalent salt in water (e.g., NaCl) + + + + + At each segment, a fraction of positive counterions condenses within a volume VP + VP _ DNA double helix _ _ _ _ __ _ _ _ _ _ + The charge of 1 segment is reduced to (-1+ )e Gain in enthalpy H, since repulsion between segments is diminished Loss in entropy S, since order increases Nature will choose such a fraction, that G = H T S is minimal + + + 1 equation 2 unknowns + + G/ = 0 G( , VP ) / = 0
Calculation of the electrostatic repulsion term Gel Phosphate N : 1 Charge: 2 3 ..s np qnet qnet qnet qnet qnet qnet qnet qnet b 2b efekt soli qnet = qe(1- ) i jb r 2 e 2 k q (1- ) i- b = e Each pair contributes: G e D ij j efekt vody Debye-H ckel screening factor if np>> s np np Coulomb rD= Debye screening length proportional to c-1/2 c: concentration of univalent salt All pairs contribute: |i-j| = 1: number of pairs = np-1 |i-j| = 2: number of pairs = np-2 . . |i-j| = s: number of pairs = np-s |i-j| > s: interaction negligible i-jb r 2 e 2 n L k q (1- ) b 1 i-j s p AV e G = e D el np Gij 0 i-j =1
Simplifying the sum over pairwise electrostatic repulsion terms i-jb r 2 e 2 n L k q (1- ) b 1 i-j s p AV e G = e D el i-j=1 i-jb r b r 2b r 3b r 1 i-j 1 2 1 3 s e = e + e + e +... D D D D i-j=1 Looks like a Taylor series . Cvi en 1: odvo te Taylor v rozvoj pro funkci f(x)=ln(1+x) (vy adov no je e en , nikoliv jen v sledek) Taylor v rozvoj : f(x) =
f(x) = 1 x = ( " f ) x = + ( ) ln( 1 ) f x x + 2 1 ( ) 1 2 = ( ' ) f x = ( ' " f ) x + 1 x + 3 1 ( ) x with a=0: 2 3 series converges for x<0 x x = + = + + ( ) ln( 1 ) ln 1 ...... f x x x 2 3 = /D r b : x e with 2 / 3 / b r b r e e D D = / / b r b r ln( 1 ) 0 ...... e e D D 2 3
List of Taylor series of some common functions http://en.wikipedia.org/wiki/Taylor_series
Simplifying the sum over pairwise electrostatic repulsion terms i-jb r 2 e 2 n L k q (1- ) b 1 i-j s p AV e G = e D el Cvi en 2: vypo t te sumu i-j=1 -i-jb r -b r -b r -2b r -3b r -b r s 1 i-j 1 2 1 3 since e e = e + e + e +... 1 = -ln(1-e ) D = D D D D D i-j=1 2 3 4 x 2 x 6 x 24 x e = 1+ x + + + +... -b r b r -ln(1-e ) xe = 1+x for x<<1 = -ln( ) since r b Cvi en 3: zjednodu te (rD>>b) D D D
Simplifying the sum over pairwise electrostatic repulsion terms i-jb r 2 e 2 n L k q (1- ) b 1 i-j s p AV e G = e D el Sum has become a simple logarithmic term! i-j=1 i-jb r b r 2b r 3b r 1 i-j 1 2 1 3 b r s e = e + e + e +...= -ln( ) D D D D i-j=1 D 2 e 2 n L k q (1- ) b b r p AV e G =- ln( ) el D is the dimensionless Manning structural factor proportional to charge density 2 e k q b kT 1 with = e b r b r 2 ln( ) < 0; G 0 G = - RTn (1- ) ln( ) el p el D D
Cvien 4: Vypotte pro dvojroubovicovou DNA (b = 1.7 ) p i 25 C 2 e e k q 1 with = b kT = 4.2 (single-stranded DNA: = 2.1) k = 1.38x10-23J.K-1 ke= 9.0x109Nm2C-2 T = 298 K = 78 qe= 1.60x10-19C
cbulk + + clocal= nP (nPVP)-1= VP-1 + + + A fraction of positive counterions condenses within a volume VP + VP _ DNA double helix _ _ _ _ __ _ _ _ _ _ + Loss in entropy S, since order increases + + + + +
Mixing entropy term dependent on 1 c V = = G RTln( ) RTln( ) local P n n mix P P c c bulk number of moles of couterions that is confined from cbulkto clocal cbulk= c = concentration [mol/L] of univalent salt KNOWN VP [L/mol] = volume to which the counterions/phosphate are confined VpUNKNOWN HOPELESS CALCULATION??? Let s minimize the total free energy with respect to ( G)/ = 0 1 b V = + = + 2 RTn [ ln( ) ln( )] P G G G (1 ) el mix P r c D i.e., loss in entropy when the fraction of counterions associates with each segment
Cvien 5: Vypotte prvn derivaci nsleduj funkce podle x : 1 = ( ) ln ( ) f x + 1 2x 1 = ) 1 1 ( + 1 - 2 - ( ' ) ( ) ( 2 x) 2 f x + 1 2x 2 = + 1 2 x
Minimizing total free energy 1 b V = + = + 2 RTn [ ln( ) ln( )] P G G G (1 ) el mix P r c D ( G) 1 b 1 V = + + Cvi en 6: vypo t te derivaci. Nazna te postup v po tu. RTn [ 2 (1 (-1) ln( ) 1 ln( )] P ) P r c D 1 b V = + + RTn [2 (1 ln( ) 1 ln( )] P ) P r c D 2 e k q 1 -3 10 RT 2(L b = e kT m = r 0 D 2 q ) c AV e Dependence of Gmixon c Dependence of Gelon c From Debye-H ckel Theory of electrolytes
1 b ( G) V = + + = RTn [2 (1 ln( ) 1 ln( )] 0 P ) P r c D 2 e k q 1 -3 10 RT 2(L m = b = e kT r 0 D 2 q ) c AV e 1 2 1 2 2 e 2 AV kT 10 2 e 2 e 6 e k q 2L q c 2k q L ( kT) 10 c b r 1( = = ) ( ) e kT L AV 3 -3 2 -3 D 0 AV 0 2 e 6 e 2k q L c 1 c + = 2 (1 ln[ ] 1 ln( ) 0 AV ) kT) 2 3 3 1 2 ( 10 V 0 P 2 e 6 e 2k q L c c + = (1 ln[ ] 1 ln( ) 0 AV ) kT) 2 3 3 1 ( 10 V 0 P
2 e 6 e 2k q L c c + = (1 ln[ ] 1 ln( ) 0 AV ) kT) 2 3 3 1 ( 10 V 0 P Singular terms containing ln c must vanish! We want to know at the low concentration limit, c 0 ( ) ln c ln c - - (1- ) - 1 = 0 Now we have got a second equation! -1 = 1 - dimensionless charge density of polymer c concentration of univalent electrolyte dielectric coefficient of electrolyte T absolute temperature fraction of counterions condensed VPvolume to which counerions condense k Boltzmann s constant keelectrostatic constant qeelectron charge vacuum permittivity LAVAvogadro s number knowns unknowns
2 e 6 e 2k q L c c + = (1 ln[ ] 1 ln( ) 0 AV ) kT) 2 3 3 1 ( 10 V 0 P Singular terms containing ln c must vanish! We want to know at the low concentration limit, c 0 ( ) ln c ln c - - (1- ) - 1 = 0 DNA in water: 1 Now we have got a second equation! = 1 1 = - - -1 = 4.2 = 0.76 76% of the negative charge of the phosphates is neutralized by condensed counterions! no association of counterions association of counterions
Now we can determine VP, the volume to which the counterions/nucleotide condense in double-stranded DNA c L q 2k ln[ ) (1 3 0 2k [ ln ) (1 ln(c) ) (1 0 2k [ ln ) (1 0 =1 2 e 6 e c + = AV ] 1 ln( ) 0 2 3 1 ( kT) 10 V base of natural logarithms ln(c) ] + P 2 6 e q L e AV + = ln( ) 0 kT) 2 3 3 ( 10 V P 2 e 6 e q L e 3 = AV ] 0 2 3 ( kT) 10 V P =0 2 e 6 e 2k q L e 3 = AV L 1 kT) 2k 2 3 ( 10 V 0 P 2 e ( 6 e kT) q e = AV V P 2 3 3 10 0
2 e ( 6 e kT) 2k q L e = AV V P 2 3 3 10 0 2 e 6 e 2k 2 q L e = = = 4 - 3 AV V . 0 646 L/mole 6.46x10 m / mole P kT) 3 3 ( 10 0 = 6.46x1026LAV-1 3/nucleotide = 1073 3/nucleotide = 8.85x10-12C2N-1m-2 LAV= 6.02x1023mole-1 e = 2.718 k = 1.38x10-23J.K-1 ke= 9.0x109Nm2C-2 qe= 1.60x10-19C DNA in water at 25 C = 4.2 =78 T = 298 K = 0.76
The volume to which the condensed univalent counter-cations are confined, VP Per mole: 646 cm3 Per nucleotide: 1073 3 This corresponds to a layer of ~7 around the DNA double-helix (which corresponds ~to a cylinder with a diameter of 20 ) 7 20 7 Counterion Condensation Theory: Manning, G. S. (1978) Quart. Rev. Biophys. 11: 179-246
Experimental and theoretical results supporting the Counterion Condensation Theory 1. Titration of Carboxymethylcellulose (CMC) with different hydroxides monitored by ultrasonic absorption O O OH OH O O OH OH O OH O OH O O CMC OH OH O O O OH OH O O OH + OH- OH O O O- + H2O O Titration with XOH; X = Na, K, Li, N(CH3)4 . Upon titration, the COOH groups are successively deprotonated, become COO-, and the negative charge density increases. This modifies the structure of the water layer at the surface of the polymer, which can be monitored by measuring the absorption of ultrasound. If there is no association of cations with the polyanion, the structure of the water layer should be independent of the cation.
> 1: great differences between the various hydroxides association between the counterions and the polymer, as predicted by the Manning theory < 1: no difference between the various hydroxides association between the counterions and the polymer no R. Zana et al., J. Chim. Phys, 68, 1258-1266 (1971)
2. Molecular dynamics simulations of a solvated DNA duplex % phosphate charge 7 20 7 [ ] Radial distribution of Na+counterions around a DNA duplex 12 base-pairs long, from a molecular dynamics simulation (Yang et al., J. Am. Chem. Soc. 1997, 119, 59-69). The calculation predicts 82% of the phosphate charge to be neutralized, with cations moving within a radius of 15 from the helix axis. Manning theory predicts 76% of cations within 17 from the helix axis.
Remember: -1 = 1- In a diluted solution of counterions, is concentration-independent! = fraction of monovalent cations predicted by Counterion Condensation Theory to condense on each unit charge of the poly-anion If then a fraction of 1- -1counterions condense at each unit charge If , there is no counterion condensation. duplex DNA in H2O at 298 K: = 4.2 single-stranded DNA: = 2.1
(ax)*lna http://matematika-online-a.kvalitne.cz/derivace-funkce.htm
