Electric Potentials and Energy Considerations

Concepts of electric potentials and energy in Chapter 25, courtesy of Mr. White. Dive into topics like stopping electrons with electric fields, direction considerations, and understanding electrical potential energy. Unravel the complexities of field directions, force interactions, and the conservative nature of electrostatic forces. Enhance your knowledge through engaging visuals and detailed explanations.

• Electricity Concepts
• Energy Considerations
• Electric Fields
• Potential Energy
• Mr. White

engelmann_t Follow

Uploaded on Feb 18, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

Presentation Transcript

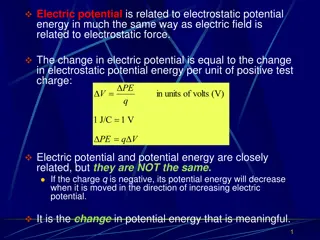
1. CHAPTER 25: Electric Potentials and Energy Considerations courtesy of Mr. White 1.)

2. General announcements 2.)

3. Problem 15.54 What will be the electric field needed to stop an electron e with kinetic energy K in distance d? In what direction should the the field be, opposite the direction of the electron s motion or with the electron s motion? 3.)

4. What electric field will be needed to stop an electron with kinetic energy K in distance d. In what direction should the the field be, opposite the direction of the electron s motion or with the electron s motion. direction of force on positive charge This dot product is a little tricky. To determine the angle between the electric field E and d, where d is in the direction of the velocity vector, we need to think a little bit about how an electron behaves in an electric field, and about what THIS electron is doing in this problem. E direction of force on negative charge The electric force on an electron in an electric field will be exactly opposite that of the electric force on a positive charge. (Remember, the direction of an electric field is defined as the direction of force on a positive charge in the field, so the direction of force on an electron will be OPPOSITE the electric field direction.) 4.)

5. If the electron is moving OPPOSITE the direction of an electric field (i.e., in the direction of the force on it), it will speed up. If the electron is to slow down, it must be moving WITH the electric field. That means the angle between E and d must be zero and the dot product will, due to its cosine factor, be positive. direction of force on negative charge in E-field But we KNOW the work being done on the electron must be negative as it s slowing down, so where does the negative sign come from? It comes from the fact that the electron feeling the force is negative. That is, q = -e = -1.6x10^-19 for an electron. That is where the negative sign comes from. Mathematically, this can be written as: direction electron must be moving if force is to slow it down 5.)

6. Electrical potential energy Electrostatic force is a conservative force - what does that mean? The work done by this force depends only on initial and final positions (not the path taken), and can be defined by a potential energy function, or W = U To help visualize this, imagine a test charge in a uniform electric field: --The field will cause the test charge to accelerate to the right, with a force qE as shown --The charge will displace an amount ? --Thus, the work done from A to B can be found by: Wab = Fx ? = qEx( ?) + + + + + + + + + - - - - - - - - - ? qE + --Using our definition from above, and calling ? = d (for displacement)we can say: Ue= Wab= qExd 6.) xi xf

7. Electrical potential energy Some things to note about this definition: The equation Ue= Wab= qEx x is only valid for a uniform electric field, for a particle displacing along a given axis This equation is valid for both positive and negative charges! Moving in the direction of the electric field yields a drop in potential energy for a positive charge, but a gain in potential energy for a negative charge. In this equation, the sign of q and sign of E should be included in the calculation For a negative charge, you need a negative sign included in your calculation. E is usually positive, but say you have a problem that asks what would happen if the field reversed direction - you would just put a negative sign in front of E as well. Electrical potential energy depends on both the charge and the field (like gravitational potential energy, mgh, this is now qEd) Also remember the work-energy theorem: W = ?KE If you know the change in electrical potential energy, you can find its change in kinetic energy! 7.)

8. Electrical potential and voltage Just as the force-per-unit-charge at a given location is called the electric field E = F/q at that point, the electric-potential-energy-per-unit-charge at a given location is called the absolute electric potential, or V = U/q. Its units, joules per coulomb, is called a VOLT. As was the case with electric fields, this tells us the amount of potential-energy per unit charge AVAILABLE to charge at a point, whether there is a charge present to feel the effect or not! By itself,this isn t very useful. On the other hand, the difference in V between two points IS very useful! This is called an electric potential difference. This has several implications! DV =DU q (units volts) means: (this gives us the work-per-unit-charge done by the firld as a charge moves from point A to point B in an electric field); U = q V (this version is useful for a uniform electric field, like one between two parallel plates. Technically, it is a dot product! qEd q = Ed V = So: V = ? ? 8.)

9. Quick check: How much potential energy does a 2 C charge have at a point where the absolute electrical potential is 3 J/C? V = U/q so U = qV = (2 C)(3 J/C) = 6 J How much potential energy does a -2 C charge have at that same point? V = U/q so U = qV = (-2 C)(3 J/C) = -6 J How much potential energy does a -2 C charge have at a point where the absolute electrical potential is -3 V? V = U/q so U = qV = (-2 C)(-3 J/C) = 6 J 9.)

10. Potential vs potential energy Electric field lines always point from high potential to low potential --> this is regardless of whether there is a positive, negative, or no charge present at that location at any time High V Low V Where high UE is, however, depends on whether the charge feeling the effect is positive or negative charge! The high UE position for a positive charge is the high V plate -- it wants to go towards the low voltage (negative) plate The high UE position for a negative charge is the low V plate -- it wants to go towards the high V (positive) plate + + + + + + + + + - - - - - - - - - High UE for a + charge High UE for a - charge 10.)

11. Another quick example An electron (e-) in a TV picture tube is accelerated from rest through a potential difference of 5000 V. The mass of an electron is 9.11 x 10-31 kg. What is the change in U of the electron? U = q V U = 1.602x10 19 C 5000V 0V U = 8x1016 J = 5000eV* What is the final speed of the electron? W = U = 8x1016 J = KE mvf 2(8x1016 J) 9.11x10 31kg= 4.05x107 m/s 2 0 = 8x1016 J 0 V 5000 V vf= *Fletch s note: An electron-volt (eV) is defined as the amount of energy an electron picks up when accelerated through a 1 volt electrical potential difference. 11.)

12. Visual practice (thanks Mr. White!) low Ue low Ue low Ue low Ue high Ue high Ue high Ue high Ue 12.)

13. Quick practice If an electron is released from rest in a uniform electric field, the potential energy of the charge-field system (a) increases (b) decreases (c) stays the same Explain! When released from rest, the electron will accelerate in the direction opposite the electric field, due to the electric force on it by that field. This means electrical potential energy is converted to kinetic energy, and the potential energy will decrease. True or false: If a proton and electron both move through the same displacement in an electric field, the change in PE for the proton must be equal in magnitude and opposite in sign to the change in PE for the electron. True: ?U = -qEd, and since both have the same magnitude charge, same displacement, and are in the same electric field, the magnitude of the change in PE will be the same, and since the electron is -, the signs will be opposite. 13.)

14. Problem 16.7 - preliminary questions An oppositely-charged set of parallel plates 5.33 mm apart have a potential difference of 600 volts between them. Preliminary questions: i.) What does the electric field look like between the plates (draw it in)? ii.) Which plate has the higher electrical potential? iii.) If you were to assume the higher potential plate had a voltage of 600 volts, what assumption are you making about the lower potential plate? iv.) In general, do negative charge move from higher to lower electrical potential, or vice versa, and do they move from higher potential energy to lower, or vice versa? 14.)

15. Problem 16.7 - preliminary questions An oppositely-charged set of parallel plates 5.33 mm apart have a potential difference of 600 volts between them. Preliminary questions: i.) What does the electric field look like between the plates (draw it in)? See sketch ii.) Which plate has the higher electrical potential? The positive plate always has higher voltage - E fields point from high to low voltage.. iii.) If you were to assume the higher potential plate had a voltage of 600 volts, what assumption are you making about the lower potential plate? That it has a relative voltage of 0 compared to the higher potential plate. iv.) In general, do negative charge move from higher to lower electrical potential, or vice versa, and do they move from higher potential energy to lower, or vice versa? Negative charges move opposite electric field lines, so they must move from the lower potential plate to the higher potential plate -- opposite the way a positive charge would move. 15.)

16. Problem 16.7 modified An oppositely-charged set of parallel plates 5.33 mm apart have a potential difference of 600 volts between them. a.) What is the E field magnitude between the plates? V = Ed 0 V 600 V = E 0.00533m E = 1.126x105 V/m(or N/C) b.) What is the force on an electron when sitting between the plates? F = qE = 1.602x10 19C 1.126x105N/C j = 1.8x104 j N c.) How much work to move the electron to the negative plate from 2.9 mm from the positive plate? You ll have to do work to force the electron to the negative plate. If you start where the electron is shown in the sketch and proceed upward, we can write: W = q V = qEd W = 1.602x10 19C 1.126x105N/C 000243 m W = 4.4x10 14J 16.)