Basic Competition Concepts in Economics

Explore fundamental economic concepts like supply and demand, consumer surplus, producer surplus, total surplus, price discrimination, and game theory. Delve into cost definitions, cost technologies, and the calculation of total surplus and consumer surplus in different market scenarios.

• Economics
• Competition Concepts
• Supply and Demand
• Consumer Surplus
• Producer Surplus

whi_ehr Follow

Uploaded on Oct 08, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Business of Platforms: Summary of Basic Competition Concepts (version 02162023) Supply and demand Costs Consumer surplus (CS) Producer surplus (PS) Total surplus (TS) Price discrimination Market organization Game Theory For a more extensive discussion, see Pindyck and Rubinfeld, Microeconomics 1

2. Demand, supply, price and quantity determination 2

3. Cost definitions Total costs: Variable costs: Fixed costs: Breakdown of total costs C(q) = F + V(q). Average total cost: Average variable cost: Average fixed cost: Breakdown of average costs ATC(q) = F/q + AVC(q). Incremental (marginal) cost: MC(q) = C'(q) = dC/dq = V'(q) = dV/dq. C(q). V(q). F, constant. ATC(q) = C(q)/q. AVC(q) = V(q)/q. AFC(q) = F/q. 3

4. Three cost technologies: (1) Constant Returns to Scale 4

5. Cost technologies: (2) Increasing Returns to Scale, F > 0, MC constant 5

6. Cost technologies: (3) Increasing and Then Decreasing Returns to Scale 6

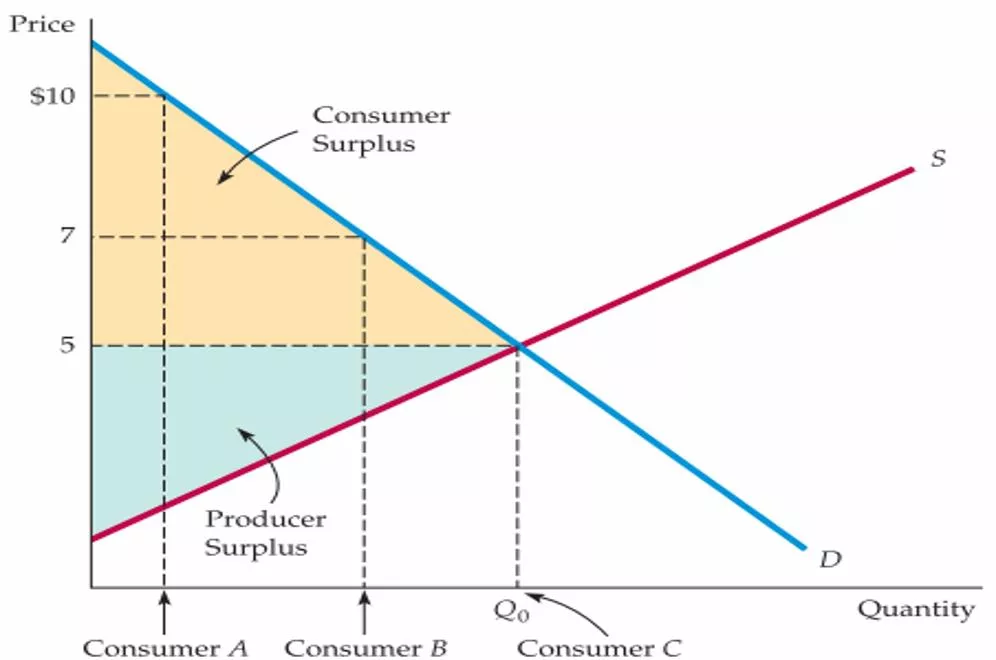
7. Total surplus = Value of a market to society, varies with Q Black lines: willingness to pay per unit, add one by one Red lines: cost per unit Black lines minus red lines: total surplus TS Benefit to society of unit Q1: $30-$20 = $10 Unit Q1 + 1 also has positive benefit, should be produced And so on up to unit Qc, where TS is maximized As output increases from Q1 to Qc, TS increases by the green triangle, also called DWL=dead weight loss p 40 Demand 30 Supply TS 20 Q Qc Q1 100 0 7

8. Consumers surplus CS = Total willingness to pay for q units minus consumers expenditure = Area under demand up to q units minus consumers expenditure = A(q) E(q) E(q) = R(q) = pq when all units are sold at the same price p Companies could sell using other pricing schemes Keep in mind that, in network industries, the CS calculation will be different Because value increases with higher sales 8

9. 9

10. Producers surplus PS = Revenue up to q units minus variable costs = Revenue minus area under marginal cost curve = pq V Note that Variable Cost V(q) is the area under Marginal Cost MC(q) 10

11. 11

12. Total surplus has two parts: Consumer surplus (CS): benefit of consumers over and above what they pay Producer surplus (PS): benefit of producers over and above their costs TS = CS + PS 12

13. 13

14. Total surplus TS(q) = CS(q) +PS(q) = A(q) E(q) + E(q) V(q) = A(q) V(q) = net contribution to society of a market for q units (excluding fixed costs) We judge markets according to contribution to TS TS is maximized at qc. Difference TS(q)-TS(qc) = dead weight loss = loss to society from less or more production than qc. Example of less production than qc: monopoly Example of more production than qc: subsidy 14

15. Total surplus is maximized at qc TS Keep in mind that this will NOT be true in network industries 0 qc q 15

16. Price discrimination: ways to reduce consumer surplus and increase profits Offer different prices to the same buyer for different units of the same good Offer different prices for the same good to different buyers Requirement 1: Need to know what each person is willing to pay for each unit Requirement 2: Need to have no resale If there is resale, price discrimination fails, and prices are arbitraged to the same level 16

17. Some forms of price discrimination Tying: good A not sold without good B For many years, Apple was not selling Macs without an Apple monitor! Mixed bundling: selling collections of goods at lower prices than a-la-carte Microsoft sells the Office bundle as well as a- la-carte Word, Excel, etc. Loyalty-requirement contract: Discounts are offered if the buyer commits to buying all or most of his needs from same seller 17

18. Price discrimination towards the same buyer Selling different units of the same product to the same buyer at different prices Electricity bills: higher marginal price for more units In some other cases, last unit(s) sold at a lower price (quantity discount) Collecting a fixed fee with or without a variable fee Mobile, fixed telecom service bills Amusement park charges at entrance and per ride 18

19. SKIP Price discrimination towards the same buyer Loyalty-requirement contract: Offer a discount on some or all units only if buyer buys 90% of his needs from you Or offer a discount on some or all units of good A only if the buyer buys 90% of his needs of goods A & B Essentially forces buyer to buy B from dominant firm in A 19

20. SKIP Price discrimination towards the same buyer. Problems of requirement contracts What if there is a monopolist in good A but there are rivals in good B that produce superior quality of B? Under the requirement contract, rivals in B are eliminated or marginalized with significant TS loss 20

21. SKIP Price discrimination towards the same buyer Problems of requirement contracts A multi-unit buyer has some CS left in product A even under monopoly The monopolist seller can extract more CS by requiring that the product be only sold with B 21

22. Price discrimination across buyers Example: IBM in the 1930s leased tabulating machines; required buyers to buy IBM cards Card use proportional to machine use Machine use roughly proportional to value to buyer IBM used tying to quantify the value to individual buyer IBM used tying to effectively lease different units of the tabulating machines to different buyers at different prices Often tying and bundling are illegal under antitrust law, but need to show consumer surplus loss under tying or bundling 22

23. Market organization Perfect competition Many rivals Market price is equal to incremental cost No need to take rivals into account Example: tiny wheat farmers Monopoly Single seller Market price significantly exceeds incremental cost No rivals Example: patent holder 23

24. Market organization (cont.) Oligopoly Few firms Market price above cost but below monopoly price Need to take rivals actions and reactions into account Use game theory to find market equilibrium 24

25. Market concentration index Herfindahl-Hirschman index of concentration HHI = in=1 si2 Sum of squares of market shares Not sum of shares, which is always 1! Range from 1 (monopoly) to 0 (perfect competition) At egalitarian equality, all si = 1/n, HHI = n(1/n2) = 1/n As n becomes very large, HHI goes to zero Lawyers multiply in=1 si2 by 10,000 so for them the HHI range is [0, 10000] 25

26. Market classifications by HHI defined by DOJ and FTC http://www.justice.gov/atr/public/guidelines/hhi.html HHI between 1,500 and 2,500: moderately concentrated HHI > 2,500: highly concentrated Mergers that increase HHI by more than 200 in highly concentrated markets are presumed likely to enhance market power and require additional scrutiny 26

27. Game Theory Application to Oligopoly Game in extensive form: N players; decision tree; moves; outcomes; information sets; payoffs 1, 2; Game in normal form: strategies Perfect information, perfect recall Complete information; Harsanyi s analysis of incomplete information 27

28. Games in extensive form: sequential incumbent-entrant game Payoffs Decision nodes Moves 28

29. Perfect information means That you know all the previous events and moves of opponent in the game Example: sequential entry/incumbent game (above) Imperfect info means that you are not sure of what has happened in the past Example: simultaneous entry/incumbent game where the incumbent does not know if the entrant has entered (see below) 29

30. Games in extensive form: simultaneous games Information set 30

31. Incomplete informationmeans That you do not know the utility of the opponent at various outcomes For example, in the incumbent/entrant game, under complete information, entry + high Q results in (-3, 6) If there was incomplete information, the entrant would not know if the utility of the incumbent was 6 31

32. Incomplete information was important during the cold war The United States did not know the utility function of the USSR The issue was resolved by Harsanyi by considering that the US played against different types of Russians. In the incumbent/entrant game, for example, the entrant may be playing against a tough incumbent whose utility at entry + high Q is 10 or a soft incumbent with utility 4 for the same outcome 32

33. John Harsanyi 33

34. Two solution approaches Cooperative approach, when there are legally binding, enforceable contracts available Find the strategies that maximize total profits Figure out how to split the profits among players Keep in mind that cooperation among rivals is typically an antitrust violation Non-cooperative approach, when there are no legally binding contracts available 34

35. John Nash 35

36. Non-cooperative equilibrium Basic idea of Nash: Assume that player 1 plays a particular (arbitrary) strategy s1 Let player 2 maximize her profits, resulting in her strategy s2 = b2(s1), her best reply to s1 Similarly, assume that player 2 plays a particular strategy s2 Let player 1 maximize her profits, resulting in her strategy s1 = b1(s2), her best reply to s2 Non-cooperative equilibrium is (s1*, s2*) when both players play their best replies: s1* = b1(s2*), s2* = b2(s1*) 36

37. Non-cooperative games Non-cooperative equilibrium (Nash equilibrium, NE): A pair of strategies (s1*, s2*) such that no player can increase his payoff provided that the rival does not change his strategy Best reply: b1(s2) maximizes 1(s1, s2) Best reply: b2(s1) maximizes 2(s1, s2) At the NE, both sides play their best replies At NE, s1* = b1(s2*), s2* = b2(s1*) 37

38. Prisoners dilemma: arrows show the best replies Player 2 talk (2, 2) (0, 6) silent (6, 0) (5, 5) Player 1 Talk Silent Non-cooperative equilibrium is where two arrows point to, at (2, 2) Top red arrow shows that if pl. 1 talks, it is optimal for pl. 2 to talk Left blue arrow shows that if pl. 2 talks, it is optimal for pl. 1 to talk 38

39. Prisoners dilemma: arrows show the best replies Player 2 talk (2, 2) (0, 6) silent (6, 0) (5, 5) Player 1 Talk Silent Non-cooperative equilibrium is at (2, 2) even though (5, 5) maximizes total utility Players cannot commit to stay silent! Talk is a dominant strategy for pl. 1 because it is the optimal strategy for pl. 1 no matter what pl. 2 does talk is a dominant strategy for pl. 2 because it is the optimal strategy for pl. 2 no matter what pl. 1 does 39

40. Arms Race Game between the US and USSR Player 2 high Q low Q (6, 0) (5, 5) Player 1 High Q (2, 2) Low Q (0, 6) Non-cooperative equilibrium is at (2, 2) even though (5, 5) maximizes total utility Players would like to commit to low Q amount of arms, but there is no higher authority to enforce it 40

41. Prisoners Dilemma as an Oligopoly Game Player 2 high Q low Q (6, 0) (5, 5) Player 1 High Q (2, 2) Low Q (0, 6) Non-cooperative equilibrium is at (2, 2) even though (5, 5) maximizes total profits Companies would like to commit to low Q, but an agreement to both produce low Q is price fixing collusion, a violation of antitrust law 41

42. Simultaneous Incumbent- Entrant Game Pl. 2 (I) high Q (-3, 6) low Q (8, 8) (0, 9) Pl. 1 (E) Enter Stay Out (0, 18) There are two non-cooperative equilibria at (0, 18) and (8, 8) Comparing the equilibria, note that pl. 1 prefers (8, 8) while pl. 2 prefers (0, 18) 42

43. Battle of the Sexes Coordination Game Player 2 ballet (2, 1) boxing (1, 1) (1, 2) Player 1 Ballet Boxing (0, 0) Three non-cooperative equilibria at (2, 1), (1,1) and (1, 2) Which one will occur? 43

44. Matching Pennies Zero sum game Player 2 heads (1, -1) (-1, 1) tails (-1, 1) (1, -1) Player 1 Heads Tails There is no non-cooperative equilibrium Could use mixed strategies probability distributions over the pure strategies {H, T} John Nash (1951): there exists a non- cooperative equilibrium in mixed strategies for any matrix game 44

45. Finding the Nash equilibrium in sequential games Work backwards If E enters, what is the best choice of I? Low Q (8, 8) If E stays out, what is the best choice of I? High Q (0, 18) Now go back to E s choice If E chooses Enter I chooses Low Q (8, 8) If E chooses Stay out I chooses High Q (0, 18) Therefore, E chooses Enter and receives profit of 8 Then I chooses Low Q and the Nash equilibrium is (8, 8) 45

46. Is cooperation needed? To establish a network link between A and B it needs to be profitable for both A and B 46

47. Review questions (1) Define the difference between average and marginal costs Define the various costs concepts Describe three different technologies of production Define consumer surplus, producer surplus, total surplus. Show in diagram. What is deadweight loss? Show in diagram. Does it arise in perfect competition? Monopoly? Oligopoly? How do we measure market concentration? 47

48. Review questions (2) Define price discrimination and its types. Give current real-world examples. True or False: We can describe sequential games in normal (matrix) form. What is perfect information? Imperfect info.? What is complete info.? Incomplete info.? What is a non-cooperative (Nash) equilibrium? Can there be more than one in a game? Design a normal form game where the non- cooperative (Nash) equilibrium does not maximize the overall surplus of all the players. Why is this true? 48