Applications of the Normal Distribution and Assessing Normality

This content delves into the practical applications of the normal distribution, covering topics like finding Z-scores for given probabilities, probability calculations, and assessing normality. Examples and detailed explanations are provided to enhance understanding.

• Normal Distribution
• Z-score
• Probability
• Assessing
• Statistics

rine_ty Follow

Uploaded on Feb 21, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Modular 12 Ch 7.2 Part II to 7.3

2. Ch 7.2 Part II Applications of the Normal Distribution Objective B : Finding the Z-score for a given probability Objective C : Probability under a Normal Distribution Objective D : Finding the Value of a Normal Random Variable Ch 7.3 Assessing Normality Ch 7.4 The Normal Approximation to the Binomial Probability Distribution (Skip) Objective A : Continuity Correction Objective B : A Normal Approximation to the Binomial

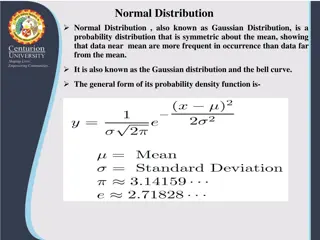
3. Ch 7.2 Applications of the Normal Distribution Objective B : Finding the Z-score for a given probability Area Area = 5 . 0 Area 5 . 0 5 . 0 Example 1 : Find the Z-score such that the area under the standard normal curve to its left is 0.0418. _____) 73 . 1 P Z = ( 0.0418 From Table V . 0 03 0418 . 0 7 . 1 0418 . 0 = ? Z 0

4. Example 2 : Find the Z-score such that the area under the standard normal curve to its right is 0.18. ( ______ ) 0.18 P Z = 1 82 . 0 = From Table V . 0 92 . 0 02 . 0 18 . 0 18 9 . 0 8186 . 0 8212 . 0 (Closer to 0.82) = ? Z Example 3 : Find the Z-score such that separates the middle 70%. = (_____ . 1 _____) 04 . 1 0.70 P Z 04 70 % From Table V . 0 04 = = ? Z ? Z 15 . 0 % 15 15 . 0 % 15 0 . 1 1515 . 0 0.1492 (Closer to 0.15) or or . 1 = = . 1 04 ( Due to symmetry ) 04 Z Z

5. Ch 7.2 Part II Applications of the Normal Distribution Objective B : Finding the Z-score for a given probability Objective C : Probability under a Normal Distribution Objective D : Finding the Value of a Normal Random Variable Ch 7.3 Assessing Normality Ch 7.4 The Normal Approximation to the Binomial Probability Distribution Objective A : Continuity Correction Objective B : A Normal Approximation to the Binomial

6. Ch 7.2 Applications of the Normal Distribution Objective C : Probability under a Normal Distribution Step 1 : Draw a normal curve and shade the desired area. =X Step 2 : Convert the values of to scores using . X Z Z Z Step 3 : Use Table V to find the area to the left of each score found in Step 2. Step 4 : Adjust the area from Step 3 to answer the question if necessary.

7. X Example 1 : Assume that the random variable is normally distributed with mean and a standard deviation . (Note: This is not a standard normal curve because and .) 1 = = 50 7 0 ( 58 ) P X (a) =X Z X 50 58 58 50 = 7 8 0.8729 = . 1 14 7 ( Z . 1 14 ) P Z . 1 14 0 From Table V = 0.8729

8. X ( 45 = 63 ) P (b) 9686 . 0 = 63 X 45 X 2389 . 0 =X =X Z Z 63 50 45 50 = = 7 7 13 5 = = Z . 1 86 . 0 71 0 7 7 . 1 . 0 86 Z 71 Z . 0 . 1 ( 71 86 ) P From Table V 71 . 0 86 . 1 Z 2389 . 0 9686 . 0 = 2389 . 0 the ( 9686 . 0 7297 . 0 = whole blue area )

9. Example 2 : GE manufactures a decorative Crystal Clear 60-watt light bulb that it advertises will last 1,500 hours. Suppose that the lifetimes of the light bulbs are approximately normal distributed, with a mean of 1,550 hours and a standard deviation of 57 hours, what proportion of the light bulbs will last more than 1650 hours? ( = 1650 ) = P X 9599 . 0 0401 . 0 = 1650 , 1550 , 57 X =X Z Z . 1 75 0 ) 1650 ( Z 1550 . 1 75 P = 57 From Table V 75 . 1 100 = 9599 . 0 57 = = 9599 . 0 1 0401 . 0 . 1 75 Z

10. Ch 7.2 Part II Applications of the Normal Distribution Objective B : Finding the Z-score for a given probability Objective C : Probability under a Normal Distribution Objective D : Finding the Value of a Normal Random Variable Objective E : Applications Ch 7.3 Assessing Normality Ch 7.4 The Normal Approximation to the Binomial Probability Distribution Objective A : Continuity Correction Objective B : A Normal Approximation to the Binomial

11. Ch 7.2 Applications of the Normal Distribution Objective D : Finding the Value of a Normal Random Variable Step 1 : Draw a normal curve and shade the desired area. Step 2 : Find the corresponding area to the left of the cutoff score if necessary. Z Step 3 : Use Table V to find the score that corresponds to the area to the left of the cutoff score. =X Step 4 : Obtain from by the formula or . Z x = + z x Z

12. Ch 7.2 Applications of the Normal Distribution Objective D : Find the Value of a Normal Distribution Example 1 : The reading speed of 6th grade students is approximately normal (bell-shaped) with a mean speed of 125 words per minute and a standard deviation of 24 words per minute. (a) What is the reading speed of a 6th grader whose reading speed is at the 90% percentile? = = . 1 = 125 , 24 , 28 Z 90 9 . 0 % Solve for X or =X Z . 1 = 28 Z From Table V 08 . 0 125 =X . 1 28 24 = X . 1 28 ( 24 ) 125 2 . 1 8997 . 0 (Closer to 0.9) 9015 . 0 . 1 = + 28 ( 24 ) 125 X = 155 72 . X words per minute

13. (b) Determine the reading rates of the middle 95% percentile. = = 125 , 24 , solve for X 95 . 0 % 95 or Z = Z = 1.96 1.96 = = ? Z ? Z =X =X Z Z 5 . 2 . 0 % 025 5 . 2 . 0 % 025 or or X X 125 24 125 24 1.96 = = 1.96 Z = Z = 1.96 due to symmetry 1.96 1.96(24) = = 125 X 1.96(24) 125 X From Table V 0.06 X = + 1.96(24) 125 X = + 1.96(24) 125 X = words per minute 172.04 X = words per minute 77.96 1.9 . 0 025

14. Ch 7.2 Part II Applications of the Normal Distribution Objective B : Finding the Z-score for a given probability Objective C : Probability under a Normal Distribution Objective D : Finding the Value of a Normal Random Variable Ch 7.3 Assessing Normality Ch 7.4 The Normal Approximation to the Binomial Probability Distribution Objective A : Continuity Correction Objective B : A Normal Approximation to the Binomial

15. Ch 7.3 Assessing Normality Ch 7.3 Normality Plot Recall: A set of raw data is given, how would we know the data has a normal distribution? Use histogram or stem leaf plot. Histogram is designed for a large set of data. For a very small set of data it is not feasible to use histogram to determine whether the data has a bell-shaped curve or not. We will use the normal probability plot to determine whether the data were obtained from a normal distribution or not. If the data were obtained from a normal distribution, the data distribution shape is guaranteed to be approximately bell-shaped for n is less than 30.

17. Perfect normal curve. The curve is aligned with the dots. Almost a normal curve. The dots are within the boundaries. Not a normal curve. Data is outside the boundaries.

18. Example 1: Determine whether the normal probability plot indicates that the sample data could have come from a population that is normally distributed. (a) Not a normal curve. The sample data do not come from a normally distributed population.

19. (b) A normal curve. The sample data comes from a normally distribute population.