Most recommended Solved CBSE Question Bank Class 10 French for language proficie
Together with CBSE Question Bank Class 10 Board Exam 2025 has been prepared as per the latest syllabus for Board Exams for Academic Session 2024-25. French Study Material Class 10 for exam 2024-25 is the best CBSE reference book with Section -wise Reading Material, MCQs, case\/source based questions
1 views • 1 slides
Best CBSE Question Bank Class 10 Science with answers for 2025 Board exam prepar
Together with CBSE Question Bank Class 10 Science for Session 2024-25 released. Solved Study Material for Grade X includes Chapter-wise Mind Maps & Topic-wise questions. CBSE 2024 class 10 Question Bank comprises MCQs, Short & Long answer type questions, Case\/source based questions to get excellent
1 views • 1 slides
CBSE Question Bank Class 12 Chemistry for 2025 Board exam preparation |Rachna Sa
Together with CBSE 2024 Question Bank Class 12 Chemistry is based on latest Syllabus for Session 2024-25. Best CBSE Question Bank for Class 12 includes Study Material and Topic-wise questions with solution. Chapter-wise Mind Maps, Solved & Practice Questions, NCERT Textbook Exercises along with Prac
2 views • 1 slides
Introduction to Constraint Satisfaction Problems
A Constraint Satisfaction Problem (CSP) involves assigning values to a set of variables while satisfying specific constraints. This problem-solving paradigm is utilized in constraint programming, logic programming, and CSP algorithms. Through methods like backtracking and constraint propagation, CSP
9 views • 78 slides
Understanding the Formulation of Hypothesis and Research Problem Definition
Research problems arise from situations requiring solutions, faced by individuals, groups, organizations, or society. Researchers define research problems through questions or issues they aim to answer or solve. Various sources such as intuitions, research studies, brainstorming sessions, and consul
5 views • 25 slides
5 Common Soil Stabilization Problems Solved by Rhino Snot
Rhino Snot offers effective solutions for soil stabilization issues, making it a versatile choice for dust control products.
1 views • 1 slides
5 Common Soil Stabilization Problems Solved by Rhino Snot
Rhino Snot offers effective solutions for soil stabilization issues, making it a versatile choice for dust control products.
1 views • 9 slides
Fluid Mechanics Problem Solving with Bernoulli and Momentum Equations
Explore solved problems related to Bernoulli and momentum equations in fluid mechanics, including calculations of discharge, velocity, flow types, pressure losses, and energy lines. Dive into scenarios involving conduit profiles, pipeline configurations, and Reynolds number calculations for water an
0 views • 21 slides
Understanding the Importance of Pattern Recognition in Computational Thinking
Pattern recognition is a vital skill in computational thinking, enabling the identification of similarities and differences between concepts and objects. By recognizing patterns, individuals can efficiently solve complex problems, create shortcuts, and avoid duplications in problem-solving processes
0 views • 10 slides
Understanding The Simplex Method for Linear Programming
The simplex method is an algebraic procedure used to solve linear programming problems by maximizing or minimizing an objective function subject to certain constraints. This method is essential for dealing with real-life problems involving multiple variables and finding optimal solutions. The proces
0 views • 56 slides
Linear Programming Models for Product-Mix Problems and LP Problem Solutions
This unit covers the formulation of linear programming (LP) models for product-mix problems, including graphical and simplex methods for solving LP problems along with the concept of duality. It also delves into transportation problems, offering insights into LP problem resolution techniques.
0 views • 137 slides
Learning Objectives in Mathematics Education
The learning objectives in this mathematics course include identifying key words, translating sentences into mathematical equations, and developing problem-solving strategies. Students will solve word problems involving relationships between numbers, geometric problems with perimeter, percentage and
0 views • 30 slides
Understanding Applied Geomorphology for Sustainable Environmental Management
Applied geomorphology plays a crucial role in urban planning, natural hazard mapping, land use planning, and environmental management. By applying geomorphic knowledge, problems related to land occupancy, resource exploitation, and environmental planning can be analyzed and solved effectively. Geomo
0 views • 26 slides
Introduction to Mathematical Programming and Optimization Problems
In optimization problems, one aims to maximize or minimize an objective based on input variables subject to constraints. This involves mathematical programming where functions and relationships define the objective and constraints. Linear, integer, and quadratic programs represent different types of
0 views • 25 slides
Examples of Optimization Problems Solved Using LINGO Software
This content provides examples of optimization problems solved using LINGO software. It includes problems such as job assignments to machines, finding optimal solutions, and solving knapsack problems. Detailed models, constraints, and solutions are illustrated with images. Optimization techniques an
1 views • 41 slides
Formulation of Linear Programming Problems in Decision Making
Linear Programming is a mathematical technique used to optimize resource allocation and achieve specific objectives in decision-making. The nature of Linear Programming problems includes product-mix and blending problems, with components like decision variables and constraints. Various terminologies
1 views • 14 slides
Engaging Mathematics Problems for Critical Thinking and Fun Learning
Explore a collection of engaging mathematics problems and classical brain teasers that challenge students to think critically, problem-solve creatively, and have fun while learning. From dissection tasks to card dealing challenges, these problems encourage students to readjust, reformulate, and exte
0 views • 36 slides
Understanding Number Systems and Conversions in Computer Systems
Explore the fundamentals of number systems in computer science, including decimal, binary, octal, and hexadecimal. Learn conversion methods between different bases and practical examples like converting decimal to binary. Dive into octal-binary conversions with solved problems. Enhance your knowledg
1 views • 16 slides
Algorithm Design Techniques: Divide and Conquer
Algorithm design techniques such as divide and conquer, dynamic programming, and greedy algorithms are essential for solving complex problems by breaking them down into smaller sub-problems and combining their solutions. Divide and conquer involves breaking a problem into unrelated sub-problems, sol
1 views • 13 slides
Understanding and Treating Sleep Problems in Children with Autism
Sleep problems in children with autism are viewed as skill deficits that can be addressed through relevant skills teaching. Good sleep is crucial for children's overall well-being, as it affects mood, behavior, learning, and physical health. Lack of good sleep can lead to irritability, fatigue, unin
0 views • 75 slides
Computational Complexity and NP-Complete Problems
In today's discussion, we delved into computational complexity and the challenges faced in finding efficient algorithms for various problems. We explored how some problems defy easy categorization and resist polynomial-time solutions. The concept of NP-complete problems was also introduced, highligh
0 views • 38 slides
The Oldest Applications of Linear Algebra in Ancient Civilizations
Linear algebra has roots in ancient civilizations like Egypt, where mathematical problems related to land measurement, resource distribution, and taxation were solved using techniques like Gaussian elimination and Cramer's Rule. The Rhind Papyrus from 1650 B.C. contains examples of linear systems an
0 views • 30 slides
Automatically Generating Algebra Problems: A Computer-Assisted Approach
Computer-assisted refinement in problem generation involves creating algebraic problems similar to a given proof problem by beginning with natural generalizations and user-driven fine-tuning. This process is useful for high school teachers to provide varied practice examples, assignments, and examin
0 views • 16 slides
Fermi Problems and Estimation Techniques in Science
Understand Enrico Fermi's approach to problem-solving through estimation in science as demonstrated by Fermi Problems. These problems involve making educated guesses to reach approximate answers, fostering creativity, critical thinking, and estimation skills. Explore the application of Fermi Problem
0 views • 23 slides
Optics Solved Problems: How to Solve for Focal Lengths
This content provides solutions to various optics problems involving thick lenses, double convex lenses, bi-convex lenses, compound lenses, and more. It covers topics such as identifying principal and focal points, calculating image distances, determining the effective focal length of lens systems,
1 views • 12 slides
Greedy Algorithms and Optimization Problems Overview
A comprehensive overview of greedy algorithms and optimization problems, covering topics such as the knapsack problem, job scheduling, and Huffman coding. Greedy methods for optimization problems are discussed, along with variations of the knapsack problem and key strategies for solving these proble
0 views • 17 slides
Understanding Linear Optimization in MS&E 214
Linear optimization involves maximizing or minimizing a linear function subject to constraints. This week's focus in MS&E 214 is on linear programming, basic feasible solutions, duality theory, and extreme point solutions. The concept of linear programs, such as the example of maximizing x + 3y subj
0 views • 36 slides
Optimization Problems and Solutions Using LINGO Programming
Explore optimization problems solved using LINGO programming. Examples include minimizing total job assignment costs, finding optimal solutions, and solving knapsack problems. Follow along with detailed images and instructions for each scenario presented.
0 views • 14 slides
Understanding Signatures, Commitments, and Zero-Knowledge in Lattice Problems
Explore the intricacies of lattice problems such as Learning With Errors (LWE) and Short Integer Solution (SIS), and their relation to the Knapsack Problem. Delve into the hardness of these problems and their applications in building secure cryptographic schemes based on polynomial rings and lattice
0 views • 44 slides
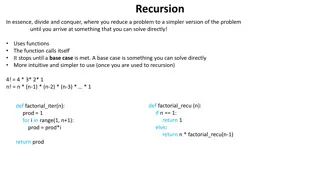
Understanding Recursion in Computer Science
Recursion is a powerful concept in computer science that involves breaking down a problem into smaller, more manageable parts until a base case is reached and solved directly. By utilizing functions that call themselves, recursion offers an elegant way to solve complex problems. This post delves int
0 views • 13 slides
Offline Mobile Data Solutions Overview & Live Demo by Mi-Corporation
Explore Mi-Corporation's Offline Mobile Data Solutions through speaker introductions, corporate overview, customer problems solved, and more. Discover how their enterprise mobility software optimizes processes, enhances productivity, and ensures data accuracy, all while positively impacting the worl
0 views • 29 slides
Plane Sweep Algorithms in Computational Geometry
Plane sweep algorithms are a powerful technique in computational geometry for solving various problems efficiently. By simulating the sweep of a vertical line across the plane and maintaining a cleanliness property, these algorithms can process events at discrete points in time to update the status
0 views • 26 slides
Understanding Divide and Conquer Algorithms for Big Data
Divide and Conquer Algorithms are a powerful paradigm in computer science where a problem is broken down into smaller parts, solved individually, and then combined to solve the original problem. This approach is exemplified in concepts like fast multiplication algorithms and finding the kth element
1 views • 27 slides
Understanding Divide and Conquer Algorithms in Computer Science
In the recent lecture, we revisited topics such as the exam review, data compression, and mergesort. We also delved into a captivating puzzle set on the planet Og, exploring the logic behind truth-telling and lying natives. Furthermore, we discussed the transformation of recursive functions into non
2 views • 36 slides
Evolution of Interface Fracture Mechanics: A Historical Perspective
Interface Fracture Mechanics has evolved over the years with significant contributions from researchers like Griffith, Irwin, and Williams. The early years focused on linear elastic fracture mechanics, leading to the development of stress intensity factors and understanding crack propagation. Specif
0 views • 27 slides
The Remarkable Life and Legacy of Mathematician Carl Friedrich Gauss
Carl Friedrich Gauss was a prodigy who made significant contributions to mathematics at a very young age. He famously solved the problem of adding numbers from 1 to 100 quickly by recognizing a pattern. Despite personal tragedies, including the loss of his first wife and child, Gauss continued to ex
0 views • 15 slides
Theory of Computation: Decidability and Encoding in CSE 105 Class
Explore the concepts of decidability, encoding, and computational problems in CSE 105 Theory of Computation class. Learn about decision problems, encodings for Turing Machines, framing problems as languages of strings, and examples of computational problems and their encodings. Gain insights into th
0 views • 26 slides
Insights into NP-Hard Problems in Molecular Biology and Genetics
Understanding the complexity of NP-Hard Problems arising in molecular biology and genetics is crucial. These problems involve genome sequencing, global alignment of multiple genomes, identifying relations through genome comparison, discovering dysregulated pathways in human diseases, and finding spe
0 views • 24 slides
Understanding P, NP, NP-Hard, NP-Complete Problems and Amortized Analysis
This comprehensive study covers P, NP, NP-Hard, NP-Complete Problems, and Amortized Analysis, including examples and concepts like Reduction, Vertex Cover, Max-Clique, 3-SAT, and Hamiltonian Cycle. It delves into Polynomial versus Non-Polynomial problems, outlining the difficulties and unsolvability
0 views • 32 slides
Understanding Pattern Recognition in Computational Thinking
Pattern recognition in computational thinking involves identifying common elements, interpreting differences, and predicting based on patterns. It helps simplify complex problems by recognizing similarities and characteristics shared among them. Through repetition and algorithmic processes, patterns
0 views • 6 slides