FAEST: Post-Quantum Signatures and Zero-Knowledge Proofs
Delve into the world of FAEST, a post-quantum signature scheme, with a focus on publicly verifiable zero-knowledge proofs. The presentation covers VOLE-in-the-Head, families of ZK proofs, and the application of VOLE in creating VOLE-ZK proofs. Learn about the background of VOLE, its use in the desig
3 views • 26 slides
Mathematical Definitions and Theorems Illustrated
In this collection of images, various mathematical concepts are visually presented, including definitions, theorems, and proofs. The slides cover a range of topics in a structured manner, providing a concise overview of key mathematical principles. From foundational definitions to detailed proofs, t
0 views • 12 slides
Mathematical Modeling and Error Analysis in Engineering
Mathematical modeling plays a crucial role in solving engineering problems efficiently. Numerical methods are powerful tools essential for problem-solving and learning. This chapter explores the importance of studying numerical methods, the concept of mathematical modeling, and the evaluation proces
0 views • 10 slides
Indirect Proofs: Contradiction and Contraposition Examples
Indirect proofs offer a roundabout approach to proving statements, with argument by contradiction and argument by contraposition being the main techniques. Argument by contradiction involves supposing the statement is false and deriving a contradiction, while argument by contraposition relies on the
2 views • 18 slides
Mathematical Modeling and Error Analysis in Engineering
Mathematical modeling plays a crucial role in problem-solving in engineering by using numerical methods. This involves formulating problems for solutions through arithmetic operations. The study of numerical methods is essential as they are powerful problem-solving tools that enhance computer usage
5 views • 10 slides
Guide to Direct Proofs in Discrete Math
Dive into the world of direct proofs in discrete math with this comprehensive guide. Learn how to prove implications, create truth tables, and follow a step-by-step direct proof template. Test your understanding with engaging quizzes and practical examples. Master the art of logical reasoning and fo
0 views • 18 slides
Evolution of Proofs in Cryptography
Cryptography has evolved from classical proofs to interactive and probabilistically checkable proofs, enabling the development of applications like Non-Malleable and Chosen-Ciphertext Secure Encryption Schemes. Non-Malleability protects against active attacks like malleability and chosen-ciphertext
0 views • 29 slides
Mathematical Proof Techniques and Examples
Explore various proof techniques in mathematics including direct proofs, proofs by cases, proofs by contrapositive, and examples showing how to prove statements using algebra, definitions, and known results. Dive into proofs involving integers, even and odd numbers, and more to enhance your understa
2 views • 13 slides
Mathematical Proof Methods and Divisibility Rules
In this lesson, we explore various methods of proof in mathematics, including direct proof, contrapositive, proof by contradiction, and proof by cases. We delve into basic definitions of even and odd numbers and learn about proving implications. Additionally, the concept of divisibility, prime numbe
0 views • 30 slides
CS 345 Lecture 1: Introduction and Math Review
This content encompasses the introduction and mathematical review covered in CS 345 lecture 1, including topics such as sets, sequences, logarithms, logical equivalences, and proofs. It delves into sets theory, mathematical operations, deductive reasoning, and examples like the conjecture of even nu
0 views • 68 slides
Algebra and Geometry Reasoning: Concepts and Proofs
Explore key concepts in algebra and geometry reasoning, including properties of equality, distributive property, and proofs using deductive reasoning. Practice solving equations, identifying properties of congruence, and writing two-column proofs to justify mathematical statements.
0 views • 13 slides
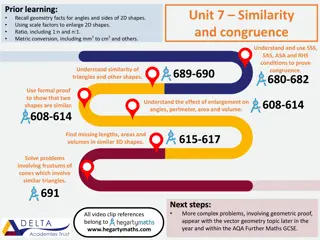
Comprehensive Mathematics Prior Learning Overview
This comprehensive overview delves into key mathematical concepts, including geometry, equations, quadratics, and circle theorems. It covers topics such as similarity, congruence, vectors, and algebraic manipulation, preparing students for more complex problem-solving and geometric proofs. The conte
0 views • 6 slides
Challenges in Constant-Round Public-Coin Zero-Knowledge Proofs
The paper discusses the implausibility of constant-round public-coin zero-knowledge proofs, exploring the limitations and complexities in achieving them. It delves into the fundamental problem of whether such proofs exist, the challenges in soundness error reduction, and the difficulties in parallel
0 views • 20 slides
Mathematical Division Theorems and Base Conversion Explained
The content covers topics such as the division algorithm, properties of divisibility, the division theorem, proofs, change of radix, and base conversion in mathematics. It delves into how integers can be divided, the relationship between divisors and multiples, and the process of converting numbers
0 views • 33 slides
Effective Learning Strategies for Mathematical Proof Comprehension
Explore self-explanation training techniques to enhance students' understanding of mathematical proofs. Dive into key concepts such as definitions, worked examples, theorems, and proofs, focusing on intuitive learning methods and practical applications.
0 views • 27 slides
Analysis: Insights and Challenges in Advanced Mathematics
Dive into the world of advanced calculus and real analysis with insights from Dr. Wai W. Lau’s course at SPU. Explore the challenges and rewards of mastering calculus, the importance of multiple exposures to the subject, and the skills needed to excel in mathematical proofs. Gain valuable perspect
0 views • 31 slides
Towards Establishing Scientifically Valid Proofs for Mythological Cosmology by Bamidele Oluwade
This presentation by Bamidele Oluwade explores the research on mythological cosmology, aiming to provide scientifically valid proofs for metaphysical phenomena through mathematical models and standard methods of proof in mathematics, supported by scientific/thought experiments and results from vario
0 views • 45 slides
Mathematical Foundations: Bounding Summations and Series
Explore the mathematical foundations of bounding summations, including the sum of first n natural numbers and geometric series. Learn about bounding each term of series, monotonically increasing and non-decreasing functions, and approximating summations by integrals. Dive into proofs, examples, and
0 views • 17 slides
Advancements in Interactive Proofs for Efficient Computation
Recent developments in interactive proofs focus on enhancing the efficiency of computations outsourced to untrusted servers, addressing concerns related to correctness and privacy. Solutions like doubly efficient interactive proofs offer a secure way to delegate computations while minimizing relianc
0 views • 25 slides
Exhaustive Proofs and Proof by Cases in Discrete Math
Exhaustive proofs and proofs by cases are essential methods in discrete mathematics for proving theorems. Exhaustive proofs involve checking all possibilities, while proof by cases focuses on considering different scenarios separately. The methods are illustrated through examples like proving (n+1)^
0 views • 8 slides
Checking Mathematical Proofs
Reading and understanding mathematical proofs involves careful analysis of logic and reasoning. Mathematicians and students use various strategies to ensure correctness, such as examining assumptions, following step-by-step logic, and verifying conclusions. This process is crucial for grasping the v
1 views • 79 slides
Evolution of Proofs in Computer Science
Explore the development of proofs in computer science, from classical mathematical proofs to interactive and zero-knowledge proofs pioneered by researchers like Goldwasser, Micali, Rackoff, and others. Discover how proof theory has evolved over time, making computation verification more efficient an
0 views • 28 slides
Constant Round Interactive Proofs for Delegating Computations
The research explores techniques for securely delegating computations to the cloud, addressing concerns of correctness and privacy through interactive proofs and efficient verification methods. It compares classical and doubly efficient interactive proofs, emphasizing the importance of computational
0 views • 43 slides
Metamath: A Computer Language for Mathematical Proofs
Metamath is a computer language designed for representing mathematical proofs. With several verifiers and proof assistants, it aims to formalize modern mathematics using a simple foundation. The Metamath-100 project is focused on proving a list of 100 theorems, with significant progress made in prov
0 views • 17 slides
Evolution of Proofs in Computer Science: Zero-Knowledge Proofs Overview
Explore the evolution of proofs in computer science focusing on succinct zero-knowledge proofs, their significance, and impact on Bitcoin protocol and public ledgers. Learn about classical proofs, zero-knowledge proofs by Goldwasser-Micali-Rackoff, and interactive proofs in the realm of computer sci
0 views • 40 slides
Mathematics Program Quality Improvement Report 2009-2010 at Department of Mathematical Sciences
This report outlines the student-learning outcomes of the Mathematics program at the Department of Mathematical Sciences. It covers areas such as knowledge of mathematical content, reasoning and proof, mathematical representation and problem-solving, mathematical communication, and knowledge of tech
0 views • 29 slides
Enhancing Critical Thinking Skills Through Mathematical Concepts in Mrs. Helenski's Classroom
Mrs. Helenski's classroom provides a safe environment where mathematical concepts are utilized to develop critical thinking skills for both mathematical knowledge and everyday life. With a focus on promoting metacognition in Geometry Honors, students are challenged to apply, prove, justify, and expl
0 views • 20 slides
Mathematical Literacy and Its Importance in Education
Recognizing the language of mathematics, understanding symbols, and being able to explain solutions are key components of mathematical literacy. It goes beyond merely answering questions correctly to encompass explaining reasoning and exploring concepts actively. The Standards for Mathematical Pract
0 views • 36 slides
Predicate Logic and Quantifiers for Symbolic Proofs
Dive into the realm of predicate logic and quantifiers, exploring the nuances of symbolic proofs and evaluating logical statements. Learn about bound variables, domain considerations, and strategies for constructing iron-clad proofs using quantifiers.
0 views • 36 slides
Quantum NIZK Proofs Explained with Dominique Unruh
Explore the concept of non-interactive quantum zero-knowledge proofs with Dominique Unruh at the University of Tartu. Discover how these proofs ensure verifier acceptance of true statements while learning nothing, and delve into the various implementations and implications of Quantum NIZK proofs wit
0 views • 19 slides
Zero-Knowledge Proofs in Cryptography
Exploring zero-knowledge proofs in cryptography, this content delves into interactive protocols, perfect zero-knowledge definitions, and the QR protocol's honest verifier and malicious verifier zero-knowledge theorems. It discusses how simulators work to maintain zero-knowledge properties and the si
0 views • 37 slides
Enhancing Mathematical Understanding through Drawing Activities
Exploring the cognitive benefits of drawing-to-learn activities in undergraduate mathematics, this content delves into visual proofs, historical mathematical concepts, and the importance of communication in mathematics. Visual thinking, creative representations, and the power of visualization are em
0 views • 36 slides
Interactive Proofs in Complexity Theory
Delve into the realm of interactive proofs in complexity theory, exploring concepts such as completeness, soundness, and efficiency. Discover how interactive proof systems can be utilized in scenarios like graph isomorphism and their implications on the complexity classes NP and coNP. Uncover the in
0 views • 40 slides
Quantifier Proofs, English Proofs
Learn how to apply the Direct Proof Rule in quantifier proofs with English proofs. Incorrect proofs are corrected by introducing assumptions and using inference rules such as Modus Ponens. Follow the steps outlined to successfully demonstrate the implications in the given statements.
0 views • 32 slides
Predicate Logic and Proofs in CSE 311
Explore the translation of statements into predicate logic, learn about inference proofs and nested quantifiers, and delve into the application of logical thinking in real-world scenarios. Discover a new way of constructing proofs and understand notation laws of inference. Engage in interactive proo
0 views • 37 slides
Innovative Approach in Mathematical Education for Maritime Students
Explore the innovative approach in mathematical education for maritime students as presented during the MareMathics Teachers Training and Meeting in Tallinn. The sessions covered topics such as mathematical applications in thermodynamics, including partial derivatives, derivations, and integrals wit
0 views • 11 slides
Mathematical Proofs and Concepts
Explore the world of mathematical proofs through chapters 4, 5, and 6. Delve into terminology, theorems, definitions, divisors, and accepted axioms used in mathematical reasoning. Discover the logic behind proofs and various methods employed in establishing the truth of mathematical statements.
2 views • 101 slides
Mastering Mathematical Induction
The power of mathematical induction with proofs, odd powers, divisibility by prime numbers, and the induction rule. Understand how to prove statements using induction techniques.
0 views • 26 slides
Tau vs. Pi: Embracing a New Constant in Math
The debate between Tau and Pi as fundamental mathematical constants, this content delves into the concept of Tau as the new circle constant. It discusses the relationship between Tau and Pi in various mathematical contexts, presenting key arguments for and against each constant. Through informative
0 views • 6 slides
Mathematical Operations Visualized
In this collection of visual representations of mathematical operations, calculations are brought to life with images and numbers. Explore addition, subtraction, multiplication, and more in a unique visual format that aids in understanding mathematical concepts. From simple equations to more complex
0 views • 43 slides