Bivariate Normal Data Analysis: LPGA 2008 Season Overview
Explore the analysis of bivariate normal data focusing on LPGA driving distance and fairway percent from the 2008 season. Learn how to compute confidence ellipses, estimated means, variance-covariance matrix, eigenvalues, eigenvectors, and plot insightful visualizations. Understand the method, set u
1 views • 8 slides
Understanding Three-Dimensional Systems of Linear Ordinary Differential Equations
Explore the concepts of three-dimensional linear systems of ordinary differential equations, including techniques for finding eigenvalues and the general solutions. Learn how to determine characteristic polynomials for 3x3 matrices and identify sink, source, and saddle points in 3D systems.
0 views • 20 slides
Understanding the Singular Value Decomposition
The Singular Value Decomposition (SVD) is a powerful factorization method for matrices, extending the concept of eigenvectors and eigenvalues to non-symmetric matrices. This decomposition allows any matrix to be expressed as the product of three matrices: two orthogonal matrices and a diagonal matri
0 views • 35 slides
Understanding Singular Value Decomposition (SVD) in Linear Algebra
Singular Value Decomposition (SVD) is a powerful technique in linear algebra that breaks down any matrix into orthogonal stretching followed by rotation. It reveals insights into transformations, basis vectors, eigenvalues, and eigenvectors, aiding in understanding linear transformations in a geomet
3 views • 18 slides
Understanding Eigenvalues and Characteristic Polynomials
Unravel the mystery of eigenvalues and characteristic polynomials through detailed lectures and examples by Hung-yi Lee. Learn how to find eigenvalues, eigenvectors, and eigenspaces, and explore the roots of characteristic polynomials to solve characteristic equations. Dive into examples to discover
2 views • 19 slides
Understanding Diagonalization in Mathematics
Diagonalization plays a crucial role in converting complex problems into simpler ones by allowing matrices to be represented in a diagonal form. The process involves finding eigenvalues and corresponding eigenvectors, ultimately leading to a diagonal matrix representation. However, careful considera
0 views • 36 slides
Understanding Eigenvalues and Eigenvectors in Linear Algebra
Explore the concepts of eigenvectors and eigenvalues in linear algebra, from defining orthonormal bases and the Gram-Schmidt process to finding eigenvalues of upper triangular matrices. Learn the theorems and examples that showcase the importance of these concepts in matrix operations and transforma
0 views • 24 slides
Understanding Diagonalization in Linear Algebra
Discover the concept of diagonalization in linear algebra through eigenvectors, eigenvalues, and diagonal matrices. Learn the conditions for a matrix to be diagonalizable, the importance of eigenvectors in forming an invertible matrix, and the step-by-step process to diagonalize a matrix by finding
0 views • 26 slides
Advanced Data Analysis Slides Showcase
Explore a series of visually engaging slides showcasing advanced data analysis concepts, including regression modeling, least squares solutions, eigenvalues, and more. The slides present information through images and equations, providing a comprehensive overview of key topics in data analysis.
0 views • 29 slides
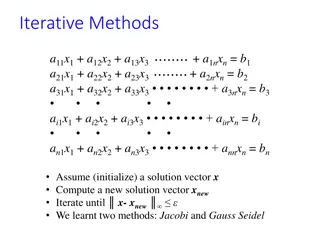
Understanding Iterative Methods in Linear Algebra
Explore the concepts of iterative methods such as Jacobi and Gauss-Seidel for solving systems of linear equations iteratively. Understand conditions for convergence, rate of convergence, and ways to improve convergence speed. Delve into iterative schemes in matrix forms, convergence criteria, eigenv
0 views • 39 slides
Understanding Lotka-Volterra Model in Mathematical Modelling
Explore the dynamics of predator-prey systems through the Lotka-Volterra model, including equilibrium points, behavior around equilibria, linearization, eigenvalue analysis, and classification of equilibria based on real and complex eigenvalues.
2 views • 14 slides
Understanding Low Threshold Rank Graphs and Their Structural Properties
Explore the intriguing world of low threshold rank graphs and their structural properties, including spectral graph theory, Cheeger's inequality, and generalizations to higher eigenvalues. Learn about the concept of threshold rank, partitioning of graphs, diameter limits, and eigenvectors approximat
0 views • 22 slides
Understanding Eigenvalues in Quantum Information
Explore the eigenvalues of sums of non-commuting random symmetric matrices in the context of quantum information. Delve into the complexities of eigenvalue distributions in various scenarios, including random diagonals, orthogonal matrices, and symmetric matrix sums. Gain insights into classical and
0 views • 24 slides
Exact Correlation Models in Biscalar Fishnet Theory
In the study of biscalar fishnet models, various operators and spectra were explored, leading to findings on exact correlation functions, strong coupling regimes, Regge limits, and more in arbitrary dimensions. The investigation delves into Lagrangian formulations, graph-building operators, conforma
0 views • 15 slides
Understanding Principal Component Analysis (PCA) in Data Analysis
Introduction to Principal Component Analysis (PCA) by J.-S. Roger Jang from MIR Lab, CSIE Dept., National Taiwan University. PCA is a method for reducing dataset dimensionality while preserving spatial characteristics. It has applications in line/plane fitting, face recognition, and machine learning
0 views • 23 slides
Understanding Eigenvectors in Linear Algebra
Explore the concept of eigenvectors in linear algebra, covering topics such as linear transforms, eigenvalues, symmetric matrices, and their practical applications. Learn how eigenvectors represent directions in which a transformation only stretches or compresses without changing direction, and unde
0 views • 25 slides
Dynamics of Orcinus orca Population: An Application of Eigentheory
This study explores the dynamics of the Orcinus orca population using eigentheory models. It examines various age groups within the population and predicts their growth over a span of 100 years. Eigenvalues play a crucial role in understanding the evolution of the population dynamics.
0 views • 23 slides
Understanding Multicollinearity in Regression Analysis
Multicollinearity is a crucial issue in regression analysis, affecting the accuracy of estimators and hypothesis testing. Detecting multicollinearity involves examining factors like high R-squared values, low t-statistics, and correlations among independent variables. Ways to identify multicollinear
0 views • 32 slides
Understanding Quantum Mechanics Postulates and Operators
Explore the foundational principles of quantum mechanics, including postulates describing quantum systems, wavefunctions, probabilistic nature, Hermitian operators, eigenvalues, and their significance in measuring physical observables.
0 views • 16 slides
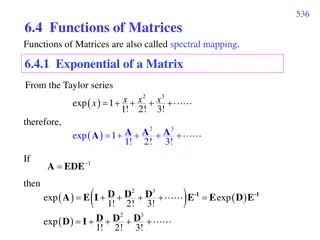
Matrix Functions and Taylor Series in Mathematics
A detailed exploration of functions of matrices, including exponential of a matrix, eigenvector sets, eigenvalues, Jordan-Canonical form, and applications of Taylor series to compute matrix functions like cosine. The content provides a deep dive into spectral mapping, eigenvalues, eigenvectors, and
0 views • 53 slides
Understanding Limit Cycles and Bifurcations in Dynamical Systems
Explore the stability of limit cycles and bifurcations in dynamical systems through examples like the Holling-Tanner model. Discover the significance of stable limit cycles and the role of Liapunov functions in forbidding closed orbits. Delve into the intriguing behavior of trajectories in bottlenec
0 views • 16 slides