Understanding Maternal and Perinatal Deaths: Key Concepts and Quiz Contest
Explore key terminologies related to maternal and perinatal deaths through an interactive quiz contest. Participants will define terms such as MMR, denominator, and multiplier. Join the session to enhance your knowledge on pregnancy-related deaths and improve awareness in maternal healthcare.
1 views • 41 slides
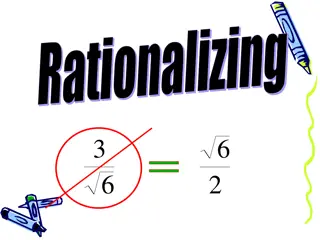
Understanding Rationalizing in Mathematics
Discover the essential concept of rationalizing in mathematics, focusing on not leaving radicals in the denominator of a fraction. Learn how to change the denominator of a fraction without altering its value by multiplying the numerator and denominator by the same number. Explore the process of rati
1 views • 18 slides
Understanding the Mechanism of Labour: Key Terms and Concepts
Mechanism of labour involves the series of passive movements a fetus undergoes during passage through the birth canal. Key terms include lie, attitude, presentation, position, denominator, presenting part, and engagement. Understanding these terms is crucial in obstetrics for assessing fetal positio
0 views • 9 slides
Understanding Points of Discontinuity in Rational Functions
Explore the concept of points of discontinuity in rational functions, where the denominator equals zero, leading to breaks in the graph. Learn about types of discontinuities such as vertical asymptotes and holes, and discover how to identify them in graphical representations through examples.
0 views • 14 slides
Understanding Partial Fraction Decomposition
The partial fraction decomposition method is a powerful technique used to simplify rational functions by breaking them into simpler fractions. It involves reducing the degree of either the numerator or the denominator. Learn about proper and improper fractions, simple and repeated factors, and how t
0 views • 17 slides
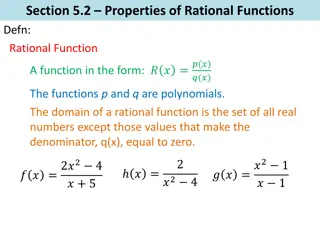
Understanding Properties of Rational Functions
Rational functions are expressed as the ratio of two polynomials. The domain of a rational function excludes values that make the denominator zero. Various examples illustrate how to determine the domain and identify asymptotes in rational functions. Vertical asymptotes exist where the denominator i
3 views • 20 slides
Understanding Decimals, Rounding, and Percentages
Decimal numbers represent values based on powers of 10, with each digit's position holding a specific place value. Rounding simplifies numbers while keeping them close in value. Additionally, percentages represent fractions of 100 and can easily be converted from fractions by writing them with a den
0 views • 14 slides
Solving Work Problems with Rational Equations
Learn how to solve work problems involving rational equations. Find the least common denominator, multiply by it, and solve for the variables. Practice examples like determining how long it takes workers to finish a task when working together. Also, solve distance equals rate times time problems to
0 views • 7 slides
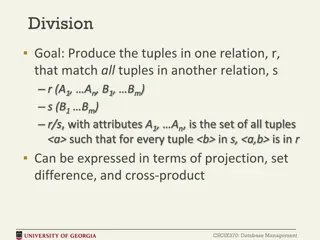
Understanding Division in Relational Algebra
Division in relational algebra is a fundamental operation used to produce tuples in one relation that match all tuples in another relation based on certain conditions. It involves expressing the desired result in terms of projection, set difference, and cross-product operations. The process ensures
0 views • 13 slides
Turning Negative Exponents into Positive Exponents Explained
Learn how to convert negative exponents into positive exponents by identifying the placement of negatives in the numerator or denominator, and moving the exponent accordingly. Follow these simple steps to master this fundamental math concept easily.
0 views • 4 slides
Understanding Vertical and Horizontal Asymptotes in Rational Functions
Vertical and horizontal asymptotes play a crucial role in understanding the behavior of rational functions. Vertical asymptotes occur when the denominator of a rational function equals zero, leading to excluded values. On the other hand, horizontal asymptotes are determined by comparing the degrees
0 views • 13 slides