Exploring Advanced Multiplication Concepts Through Arrays and Partitioning
Delve into the intricacies of multiplication through hands-on activities using arrays, partitioning, and understanding the commutative property. Discover how arrays can help visualize multiplication, learn to partition numbers for easier calculations, and grasp the concept of multiplying by powers of ten. Develop a deeper understanding of multiplication as an essential numeracy skill.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
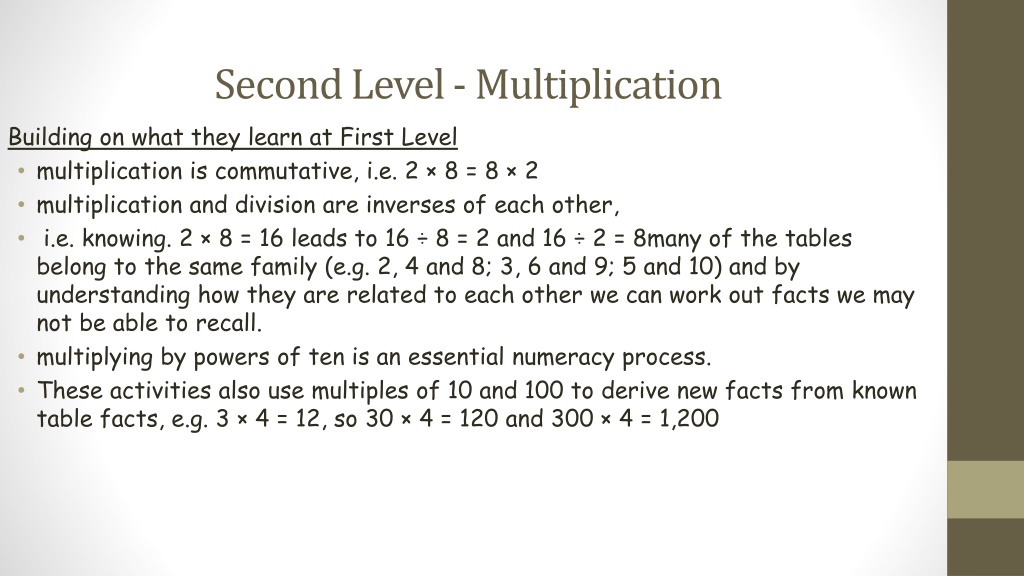
Second Level -Multiplication Building on what they learn at First Level multiplication is commutative, i.e. 2 8 = 8 2 multiplication and division are inverses of each other, i.e. knowing. 2 8 = 16 leads to 16 8 = 2 and 16 2 = 8many of the tables belong to the same family (e.g. 2, 4 and 8; 3, 6 and 9; 5 and 10) and by understanding how they are related to each other we can work out facts we may not be able to recall. multiplying by powers of ten is an essential numeracy process. These activities also use multiples of 10 and 100 to derive new facts from known table facts, e.g. 3 4 = 12, so 30 4 = 120 and 300 4 = 1,200
Arrays This array shows 7 rows of 8 dots which can be written as 7 8 = 56. Rotate it by a quarter turn and it shows 8 7 = 56, i.e. multiplication is commutative.
Arrays What does this array show?
Arrays X16 7 It shows 7 rows of 16. How does this help us figure out the answer? Partition it into manageable sums.
Arrays x10 x6 7 We can partition this into 7 x 10 + 7 x 6=?
Brackets This would be shown as (7x10) + (7x6)= 70 + 42 = 112 Brackets are used to show the thinking behind the partitioning and children should be becoming increasingly confident with their use, understanding that the calculation within has to be done before any other combining.
Brackets As multiplication is commutative, 2 7 5 is the same as 2 5 7. It is easier to swap the numbers round to make a calculation of (2 5) 7 because we know that 2 5 makes 10 We would look for a rounded number when we are using brackets and moving 3 or more multiplications around to help us. Similarly we do the same with our arrays.
Arrays We extend the use of arrays by dropping the dots within them and concentrating on the rectangles that can be partitioned into manageable sums. These look to use the example of the earlier arrays, and partitioning, to make multiplying a single digit by 2,3 and more digits X26 7
Arrays The rectangle is then split into two to partition the sum and make it easier to calculate x6 X20 7
We then use Brackets to complete the sum (7x20) + (7x6) = 140 + 42 = 182 It then leads on towards the formal method. 140 182 26 x7 (7x20) (7x6) 42
Division How we now approach long division calculations
What is division The action of separating something into parts. Introduced through the idea of sharing 18 sweets into 6 bags. What if the problem becomes 18 sweets to be shared amongst 6 bags? Repeated subtraction.
Long Division Our memories of long division? Why do most not have positive memories of the processes Only those who had confidence in all number would be successful in answering any long division questions. Endless pages of practice of the process to get the answer No need to understand the maths, just reliance on the written operations
Why change? We all carry a calculator in our pocket. Still require strong number skills to make sense of every day numbers Mobile phone sales people Politician statistics ..
So Chunking? Repeated subtraction Easier to link between multiplication and division More like the everyday mental process of division Does not rely as much on abilities to memorise all times tables and facts. Although sound tables awareness does make the whole process easier
Chunking 96 6 92 4 2 digit by 1 digit 153 9 154 7 3 digit by 1 digit 396 12 540 15 3 digits by 2 digits
Would you like a try? Text book examples ( pages 59-65) Number Investigations Presenting to each other Games, Fact files, information/guide books Word problems in different context requiring choice of operation (textboog page 74 on) Setting partner challenges (Similar to the ones set above)