Understanding Heterogeneous Catalytic Reactions: Key Steps Explained
In a heterogeneous catalytic reaction, various important steps occur, including diffusion of reactants, adsorption onto the catalyst surface, surface reactions, and desorption of products. Different mechanisms like single-site, dual-site, and Eley-Rideal mechanisms are involved in the surface reaction step. The process of adsorption and desorption plays a crucial role in the overall reaction. This review provides insights into the detailed mechanisms involved in these steps.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
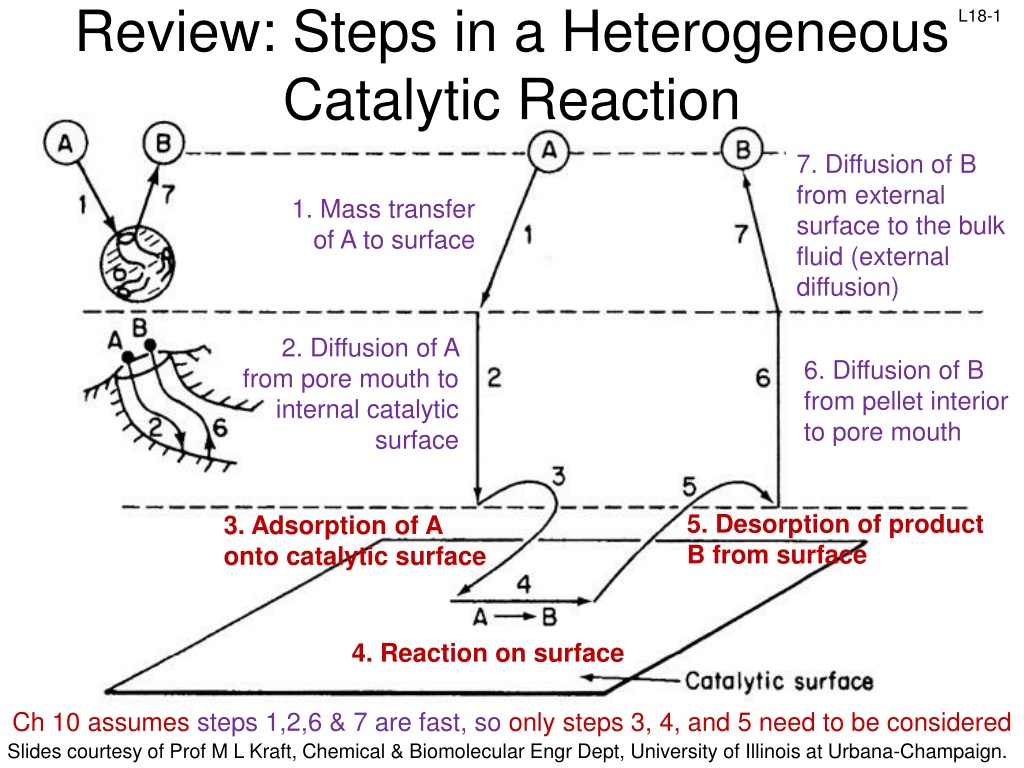
Review: Steps in a Heterogeneous Catalytic Reaction L18-1 7. Diffusion of B from external surface to the bulk fluid (external diffusion) 1. Mass transfer of A to surface 2. Diffusion of A from pore mouth to internal catalytic 6. Diffusion of B from pellet interior to pore mouth surface 5. Desorption of product B from surface 3. Adsorption of A onto catalytic surface 4. Reaction on surface Ch 10 assumes steps 1,2,6 & 7 are fast, so only steps 3, 4, and 5 need to be considered Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-2 Review: Adsorption Step The adsorption of A (gas phase) on an active site S is represented by: A A I A(g) + S A S -S-S-S- -S-S-S- S: open (vacant) surface site Rate of adsorption = rate of attachment rate of detachment r k P C = partial pressure of A k K k Review: Site Balance A S: A bound to a surface site Molar conc of vacant sites on surface = A K k C A S AD A A v A C Using adsorption equilibrium constant (KA) A S A Equation I AD r k P C = A A v A A Cv is not measurable, but the total # of sites, Ct can be measured Vacant active site B A Surface Assume the total # of active sites remains constant (no catalyst deactivation occurs): Ct = Cv + CA S + CB S Site balance: Use to express Cv in terms of measurable species Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-3 Review: Surface Reaction Step After the molecule is adsorbed onto the surface, it can react by a few different mechanisms 1. Singe site mechanism: Only the site to which the reactant is absorbed is involved in the reaction A I -S- -S- k k C B I S B S K = = where K S r k C A S B S S S A S S S Equation IIa 2. Dual site mechanism: Adsorbed reactant interacts with another vacant site to form the product A S + S S+ B S = 3. Eley-Rideal mechanism: reaction between adsorbed reactant and a molecule in the gas phase C I -S-S-S- -S-S-S A I B C C B S K v S r k C C I -S-S-S- Equation IIb S A S v S -S-S-S A S + B(g) C S = B A I C CS K S r k C P Equation IIc S A S B S Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-4 Review: Desorption Step Products are desorbed into the gas phase C S C + S = Equation III C C k k P C K D C v = where K D,C r k C I -S-S-S- -S-S-S- D,C D CS D D,C Note that the desorption of C is the reverse of the adsorption of C r = AD,C r D,C Also the desorption equilibrium constant KD,C is the reciprocal of the adsorption equilibrium constant KC K 1 = D,C K C Substituting 1/KC for KD,C in the rate equation for product desorption gives: = D,C r k C C C K P C D CS v Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
Review: Evaluating a Catalytic Reaction Mechanism Collect experimental data from test reactor Derive a rate law Select among types of adsorption, surface reaction, and desorption Write rate laws for each individual step, assuming all are reversible Postulate which step is rate limiting Surface reaction step is rate limiting ~70% of the time! Use non-rate-limiting steps to eliminate the surface concentration terms that cannot be measured Assume PSSH (rate of ads = rate of surface rxn = rate of desorp) No accumulation of species on the surface or near interface Each species adsorbed on surface is a reactive intermediate Net rate of formation of species i adsorbed on the surface is 0, ri S=0 See if rate law is consistent with data If not, then try other surface mechanism (i.e., dual-site adsorption or Eley- Rideal) or choose a different rate-limiting step (adsorption or desorption) L18-5 Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-6 L18: CVD & Catalyst Deactivation Chemical vapor deposition (CVD) Important process in the formation of microcircuits (electrically interconnected films ICs), microprocessors & solar cells Used to deposit thin films of material, such as Si, SiO2, & germanium (Ge) Mechanism of CVD is similar to those of heterogeneous catalysis except that site concentration (CV) is replaced w/ fraction of surface coverage (fV) H H H H Si silicon hydride adsorption Si Si Si Si Si Si Si Si Si Surface reaction H2 Si No desorption occurs, the product, Si, remains attached to the surface, forms a new surface Si Si Si Si Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-7 Growth of Silicon Film by CVD H H adsorption H H Surface reaction Si Si Si Si Si Si Si Si Si Si Si Si Si Si Si Write out elementary reactions and assume a rate-limiting step SiH 1. Adsorption Rate of adsorption = rate of attachment rate of detachment ( ) g + S SiH S 2 2 f SiH2 K = AD r k SiH P f k SiH f = AD r k SiH P f SiH v SiH SiH v 2 2 2 2 2 2 SiH2 fv & fSiH2:fraction of the surface covered by vacant sites or SiH2, respectively ( ) g 2. Surface reaction: + + SiH S Si S H 2 2 Si H C C K f v 2 = S r S SiH k f k Si H C P f = S r k f S v S SiH2 2 2 S Surface coverage is in terms of fraction of surface, not conc of active sites Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-8 Growth of Germanium Films by CVD Germanium films have applications in microelectronics & solar cell fabrication kdis kdis + Gas-phase dissociation GeCl GeCl Cl 4(g) 2(g) 2(g) kA kA + GeCl S GeCl S Adsorption (1) 2(g) 2 kH kH + 2(g) H 2S 2H S Adsorption (2) kS ( ) ( ) + + + GeCl S 2H S Ge s 2HCl g 2S Surface reaction Surface reaction is believed to be the rate-limiting step 2 What is the rate of Ge deposition if the surface reaction is rate limiting? a) r Dep=kdisPGeCl4-k-disPGeCl2PCl2 b) r"Dep=kAPGeCl2fv -k-AfGeCl2 c) r Dep=kHPH2fv2 -k-HfH2 d) r Dep=kSfGeCl2fH2 -k-S CGePHCl2fv2 e) r Dep=kSfGeCl2fH2 Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-9 Growth of Germanium Films by CVD Germanium films have applications in microelectronics & solar cell fabrication kdis kdis + Gas-phase dissociation GeCl GeCl Cl 4(g) 2(g) 2(g) + kA kA GeCl S GeCl S Adsorption (1) 2(g) 2 kH kH + 2(g) H 2S 2H S Adsorption (2) kS ( ) ( ) + + + GeCl S 2H S Ge s 2HCl g 2S Surface reaction 2 Surface reaction is believed to be the rate-limiting step: Rate of Ge deposition (nm/s): " 2 = Dep r S GeCl k f H f 2 ks: surface specific reaction rate (nm/s) fGeCl2:fraction of the surface covered by GeCl2 fH2:fraction on the surface occupied by H2 *Surface coverage is in terms of fraction of surface, not conc of active sites Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-10 Catalyst Deactivation Thus far, we assumed the total conc. of active sites on the surface was constant, which means the catalyst s activity is constant throughout its lifetime In reality, there is a gradual loss of catalytic activity (active sites on surface of the catalyst) as the reaction takes place Main types of catalyst deactivation Sintering (aging): loss of active surface due to high temperature Coking or fouling: carbonaceous material (coke) deposits on surface Poisoning: molecules irreversibly bind to the active site We will evaluate the kinetics of general catalyst deactivation and these specific types Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-11 Catalyst Deactivation Kinetics Adjustments for catalyst decay need to be made in the design of reactors Catalyst activity a(t) is used as a quantitative specification = At 0 ( ) 0 a t 1 Rate of consumption of reactant A on catalyst used for time t is: ' Reaction rate for catalyst used for time t r (t) r ( ) A ' a t Catalyst activity at time t: Reaction rate for fresh, unused catalyst = = a 1 For fresh, unused catalyst, t 0 = ( ) ( ) ( k T a t ) = r ' fn C C ,.. . .etc A A, B a(t): time-dependent catalyst activity k(T): T-dependent specific rate constant fn(CA, CB etc): function of gas-phase conc. of reactants, products & contaminants Functionality of rd on reacting species conc. h=1: no conc dependence; h=Cj: linearly dependent on concentration Function of activity da dt ( ) ( ) ( T h C ,C ,...,etc ) = = p a t r k Rate of catalyst decay: d d A B Temperature-dependent specific decay constant Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-12 Sintering (Aging) Loss of active surface area resulting from the prolonged exposure to high gas-phase temperatures Active surface area is lost by Crystal agglomeration and growth of metals deposited on support Narrowing or closing of pores inside the catalyst pellet Surface recrystallization Elimination of surface defects (active sites) Sintering is usually negligible at temperatures below 40% of the melting temperature of the solid Second-order decay of reaction rate with respect to present activity: = d r k a 2 d 1 ( ) = a t Catalyst activity at time t: 1 k t + d E R 1 1 T ( ) d = Sintering decay constant: k k T exp d d 0 T 0 Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-13 Coking (Fouling) Common to reactions involving hydrocarbons A carbonaceous (coke) material is deposited on surface of catalyst n Concentration of carbon on surface (g/m2): A & n are fouling parameters = C At C 1 ( ) = Catalyst activity at time t: a t m 1 k't + (one of many different expressions for a(t)) m is a fouling parameter Coking can be reduced by running at high pressure & hydrogen-rich feeds Catalyst deactivated by coking is often regenerated by burning off the carbon Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-14 Poisoning Poisoning molecule is irreversibly chemisorbed to active sites Reduces number of active sites available for reaction Catalyst can be poisoned by reactants, products, and impurities For the overall reaction: + + A S B S 'kd + A S poisoning by reactant: A S 'kd + posioning by product: B S B S 'kd + poisoning by impurity: P S P S da dt ( ) = = r a t k' C d d P a(t): time-dependent catalyst activity kd: specific decay constant CP: concentration of the poison Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-15 Moving-Bed Reactor When catalyst decay occurs at a significant rate, they require frequent regeneration or replacement of the catalyst Moving-bed reactor enables continuous regeneration of spent catalyst Operates in the steady state, like a PBR Reactant & catalyst enter at top of reactor Reactant & catalyst flow down the length of the reactor together as a plug Product and spent catalyst (black) flow out of reactor outlet Spent catalyst is regenerated by passing it through a separate regeneration unit, and newly regenerated catalyst is fed back into the top of the reactor Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-16 Moving-Bed Reactor Design fresh catalyst US (g/s) Catalyst activity vs position in PBR Catalyst flow US << reactant flow 0 As far as the reactants are concerned, reactor acts like a PBR: Assume the decay rate Reactants 0 (dm3/s) dX dW da d A = F r' A0 A W t U = S n = la w i : s k a d t Decay rate must be in terms of W (da/dW) not t (da/dt) US relates W to t use US to convert da/dt to da/dW W t U Multiply dt/dW by -da/dt to get da/dW: = d dt W Z W W + W Z + Z dt dW 1 dW U = = = dt Relate t to US: U S S S k U da dW da dt 1 n d = n a k a d U S S Products & coked catalyst Integrate to get a(W) ) ( ) ( k T fn C ( ) a W ) ( W If the rate of consumption of A for catalyst used for time t: = A0 dW F dW = r ' a W A j ( ) ( ) k F X a W T fnC d X r ' F k dX nC dX A j A A A0 A A = = d W 0 0 ( ) T f A0 j Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.
L18-17 A B Given constant T, pure A in feed, and 1st order kinetics for reaction & catalyst deactivation, find XA(t) in a fluidized batch reactor of constant volume Rate of deactivation: Rate of rxn: A A r C ak = da t = = r k a d d d Solve the batch reactor design eq for XA. The batch reactor design eq must be combined with the rate eq. The rate eq contains a , so we need to use the rate of deactivation to find how a varies w/ time. We will determine how a varies with time by integrating the rate of deactivation eq & solving for a : da r k a dt a 1 0 a = = A A kC e r Insert a into the rate eq: dX N dt X t A k t A0 A d A A0 0 0 1 X N ( ) A d A0 k N C A0 k N d A0 A X 1 e = a t da e k t d = a = lna k t = = = k dt d d d d k t k t d ( ) d dX = r k C 1 X e A A0 A k td ( ) A A = = r' W N k C 1 X e W Batch reactor design eq: A0 A A 0 A 0 A dt t C W k k C W dX k t d Integrate & solve for XA ( ) A0 N ( )( = ln 1 X e = ke dt A ( ) A0 d 0 ) ( ) C k N d A0 W C W A0 k td k t d A0 = k 1 e ln 1 X k 1 e = 1 X ( )( e A ) W k td k 1 e Slides courtesy of Prof M L Kraft, Chemical & Biomolecular Engr Dept, University of Illinois at Urbana-Champaign.