Understanding Location in a Distribution of Data
In this lesson on describing location in a distribution, you will learn how to find and interpret percentiles in quantitative data distributions, estimate values using cumulative relative frequency graphs, and understand standardized scores (z-scores). The concept is illustrated through examples like analyzing test scores in a statistics class and determining percentiles for salads at McDonald's based on calorie content. Mastering these skills will enhance your statistical analysis abilities and data interpretation techniques.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Analyzing One-Variable Data Lesson 1.9 Describing Location in a Distribution Statistics and Probability with Applications, 3rdEdition Starnes, Tabor Bedford Freeman Worth Publishers
Describing Location in a Distribution Learning Targets After this lesson, you should be able to: Find and interpret a percentile in a distribution of quantitative data. Estimate percentiles and individual values using a cumulative relative frequency graph. Find and interpret a standardized score (z-score) in a distribution of quantitative data. Statistics and Probability with Applications, 3rd Edition 2 2
Describing Location in a Distribution Here are the scores of all 25 students in Mr. Pryor s statistics class on their first test: 79 81 80 77 73 83 74 93 78 80 75 67 73 77 83 86 90 79 85 83 89 84 82 77 72 The bold score is Jenny s 86. How did she perform on this test relative to her classmates? Statistics and Probability with Applications, 3rd Edition 3 3
Describing Location in a Distribution One way to describe Jenny s location in the distribution of test scores is to calculate her percentile. Percentile An individual s percentile is the percent of values in a distribution that are less than the individual s data value. Because 21 of the 25 observations (84%) are below her score, Jenny is at the 84thpercentile in the class s test score distribution. Be careful with your language when describing percentiles. Percentiles are specific locations in a distribution, so an observation isn t in the 84thpercentile. Rather, it is at the 84th percentile. Statistics and Probability with Applications, 3rd Edition 4 4
Which salads are at McDonalds? Which salads are at McDonald s? Finding and interpreting percentiles Finding and interpreting percentiles PROBLEM:The dotplot below shows the number of calories in McDonald s salads in a recent year. (a) Find the percentile for the Premium Bacon Ranch Salad with Grilled Chicken, which contains 230 calories. 6 of the 11 salads have fewer than 230 calories. Because 6/11 0.55, or 55%, the Premium Bacon Ranch Salad with Grilled Chicken is at the 55th percentile of this distribution. Statistics and Probability with Applications, 3rd Edition 5 5
Which salads are at McDonalds? Which salads are at McDonald s? Finding and interpreting percentiles Finding and interpreting percentiles PROBLEM:The dotplot below shows the number of calories in McDonald s salads in a recent year. (b) The Premium Bacon Ranch Salad (without chicken) is at the 18th percentile of the distribution. Interpret this value in context. How many calories does the Premium Bacon Ranch Salad contain? About 18 percent of the salads have fewer calories than the Premium Bacon Ranch Salad (without chicken). Because 18% of 11 is (0.18)(11) = 1.98 (or 2 due to rounding error), the Premium Bacon Ranch Salad (without chicken) had more calories than 2 of the 11 salads on the menu. Counting up from the minimum on the dotplot, we see that the Premium Bacon Ranch Salad (without chicken) has 140 calories. Note that one other salad on the menu has 140 calories. This salad is also at the 18th percentile because 2 of the 11 salads have fewer calories than it does. Statistics and Probability with Applications, 3rd Edition 6 6
Describing Location in a Distribution There are some interesting graphs that can be made with percentiles. One of the most common starts with a frequency table for a quantitative variable and expands it to include cumulative frequency and cumulative relative frequency. Cumulative Relative Frequency Graph A cumulative relative frequency graph plots a point corresponding to the cumulative relative frequency in each interval at the smallest value of the next interval, starting with a point at a height of 0% at the smallest value of the first interval. Consecutive points are then connected with a line segment to form the graph. Statistics and Probability with Applications, 3rd Edition 7 7
Describing Location in a Distribution A cumulative relative frequency graph can be used to describe the position of an individual within a distribution or to locate a specified percentile of the distribution. Statistics and Probability with Applications, 3rd Edition 8 8
How fast can you run? How fast can you run? Interpreting cumulative relative frequency graphs Interpreting cumulative relative frequency graphs PROBLEM: As part of a student project, high school students were asked to sprint 50 yards. Their times were recorded. The cumulative relative frequency graph of the sprint times is shown. (a) One student ran the 50 yards in 8 seconds. Is a sprint time of 8 seconds unusually slow? To find this student s location in the distribution, draw a vertical line up from his sprint time (8) on the horizontal axis until it meets the graph. Then draw a horizontal line from this point to the vertical axis. See the figure below. This student s sprint time places him at about the 74th percentile. About 74% of all students who participated had lower times than this student. So this student s time is somewhat slow. Statistics and Probability with Applications, 3rd Edition 9 9
How fast can you run? How fast can you run? Interpreting cumulative relative frequency graphs Interpreting cumulative relative frequency graphs PROBLEM: As part of a student project, high school students were asked to sprint 50 yards. Their times were recorded. The cumulative relative frequency graph of the sprint times is shown. (b) Estimate and interpret the 25th percentile of the distribution. The 25th percentile of the distribution is the sprint time with cumulative relative frequency 25%. To find this value, draw a horizontal line across from the vertical axis at a height of 25% until it meets the graph. Then draw a vertical line from this point down to the horizontal axis. See the figure below. The 25th percentile is about 6.8 seconds. About 25% of the students who participated ran the sprint in a time less than 6.8 seconds. Statistics and Probability with Applications, 3rd Edition 10 10
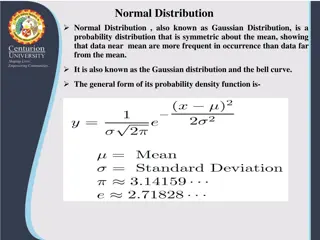
Describing Location in a Distribution A percentile is one way to describe the location of an individual in a distribution of quantitative data. Another way is to give the standardized score (z-score) for the observed value. Standardized Score (z-score) The standardized score (z-score) for an individual value in a distribution tells us how many standard deviations from the mean the value falls, and in what direction. To find the standardized score (z- score), compute value - mean standard deviation z= Statistics and Probability with Applications, 3rd Edition 11 11
Describing Location in a Distribution Values larger than the mean have positive z-scores. Values smaller than the mean have negative z-scores. Let s return to the data from Mr. Pryor s first statistics test. Where does Jenny s 86 fall within the distribution? Her standardized score (z-score) is That is, Jenny s test score is 0.99 standard deviations above the mean score of the class. Statistics and Probability with Applications, 3rd Edition 12 12
Are Caimans affected by pesticides? Are Caimans affected by pesticides? Finding and interpreting z Finding and interpreting z- -scores PROBLEM: The spectacled caiman is a crocodilian reptile that lives in Central and South America. Researchers recorded the mass (in kilograms) of fourteen caimans. The data are shown below, along with a dotplot and summary statistics. Find the standardized score (z-score) for the caiman that has a mass of 15 kg. Interpret this value in context. scores Mass 7.2 Variable n Mean StDev Minimum Q1 Median Q3 Maximum Mass 14 9.81 3.93 6.00 7.20 8.15 11.00 17.50 10 6 8.1 17.5 8.2 6.2 8 15 7.9 6 The caiman s standardized score is 11 17 9.2 ? =?? ?.?? ?.?? ?.?? This caiman s mass is 1.32 standard deviations greater than the sample mean caiman mass of 9.81 kg. Statistics and Probability with Applications, 3rd Edition 13 13
LESSON APP 1.9 Which states are rich? The following cumulative relative frequency graph and the numerical summaries describe the distribution of median household incomes in the 50 states in a recent year. 1. At what percentile is North Dakota, with a median household income of $55,766? 2. Estimate and interpret the first quartile Q1 of the distribution. 3. Find and interpret the standardized score (z-score) for New Jersey, with a median household income of $66,692. Statistics and Probability with Applications, 3rd Edition 14 14
LESSON APP 1.9 Which states are rich? Statistics and Probability with Applications, 3rd Edition 15 15
Describing Location in a Distribution Learning Targets After this lesson, you should be able to: Find and interpret a percentile in a distribution of quantitative data. Estimate percentiles and individual values using a cumulative relative frequency graph. Find and interpret a standardized score (z-score) in a distribution of quantitative data. Statistics and Probability with Applications, 3rd Edition 16 16