Economic Applications of Single-Variable Calculus Derivatives in Economics

Slide Note

In economics, derivatives play a crucial role in analyzing various economic phenomena such as marginal amounts, maximization, minimization, graphing, elasticity, and growth. This involves understanding derivatives of single-variable functions, slopes, instantaneous slopes, and the applications of derivatives in economic contexts. Derivatives help in determining the impact of small changes in variables, which is essential for decision-making in economics.

jamee Follow

Uploaded on Sep 15, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

E N D

Presentation Transcript

2. Economic Applications of Single- Variable Calculus Derivative Origins Single Variable Derivatives Economic uses of Derivatives 1

2. Economic Applications of Single- Variable Calculus 2.1 Derivatives of Single- Variable Functions 2.2 Applications using Derivatives 2

2. Economic Applications of Single-Variable Calculus In economics, derivatives are used in various ways: Marginal amounts (slope) Maximization Minimization Graphing Elasticity and Growth 3

2.1 Derivatives of Single-Variable Functions Slope: -The impact on the y variable when the x variable increases by 1 -consider the following graph -what is the impact of changing how much time you spend on assignment 1? 4

What is the impact of one more hour? Slope = rise/run Slope AB (2->3 hours) =(y1-y0)/(x1-x0) =(100-35)/(1-3) = y/ x = (y1-y0)/(x1-x0) =-32.5 mistakes Assignment 1 Mistakes Slope BC (3->4 hours) =(y1-y0)/(x1-x0) =(20-35)/(5-3) =-7.5 mistakes 120.00 100.00 80.00 Mistakes 60.00 40.00 But what is the impact of a SMALL time change at 3 hours? 20.00 0.00 1 2 3 4 5 6 7 8 9 10 Hours Spent on Assignment 1 5

2.1 Derivatives of Single-Variable Functions --in order to find the slope AT B (the impact of a small time change), one must find an INSTANTANEOUS SLOPE -slope of a tangent line at B -derivative at B 6

2.1 Tangents Slopes 120.00 100.00 80.00 Quantity 60.00 Series1 40.00 20.00 0.00 1 2 3 4 5 6 7 8 9 10 Price The green tangent line represents the instantaneous slope 7

2.1 Instantaneous Slope To calculate an instantaneous slope/find a derivative (using calculus), you need: 1) A function 2) A continuous function 3) A smooth continuous function 8

2.1.1 A Function Definitions: -A function is any rule that assigns a maximum and minimum of one value to a range of another value -ie y=f(x) assigns one value (y) to each x -note that the same y can apply to many x s, but each x has only one y -ie: y=x1/2 is not a function x = argument of the function (domain of function) f(x) or y = range of function 9

Function: y=0+2sin(2pi*x/14)+2cos(2pi*x/14) Each X 4 3 2 Has 1 Y 1 0 x y -1 1 3 5 7 9 11 13 15 17 19 -2 -3 -4 x 10

Not a Function: Here each x Corresponds to 2 y values Often called the straight line Often called the straight line test test 11

2.1.1 Continuous -if a function f(x) draws close to one finite number L for all values of x as x draws closer to but does not equal a, we say: lim f(x) = L x-> a A function is continuous iff (if and only iff) i) f(x) exists at x=a ii) Lim f(x) exists x->a iii) Lim f(x) = f(a) x->a 12

2.1.1 Limits and Continuity In other words: i) The point must exist ii) Points before and after must exist iii)These points must all be joined Or simply: The graph can be drawn without lifting one s pencil. 13

2.1.2 Smooth -in order for a derivative to exist, a function must be continuous and smooth (have only one tangent) Slopes 40.00 35.00 30.00 25.00 Quantity 20.00 Series1 15.00 10.00 5.00 0.00 1 2 3 4 5 6 7 8 9 10 Price 14

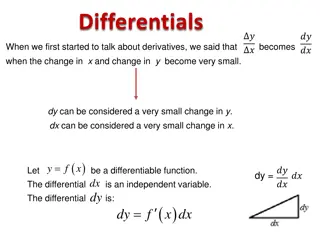
2.1.2 Derivatives -if a derivative exists, it can be expressed in many different forms: a)dy/dx b)df(x)/dx c)f (x) d)Fx(x) e)y 15

2.1.2 Derivatives and Limits -a derivative (instantaneous slope) is derived using limits: ) ( ' x f + ( ) ( ) f x h f x = lim h ( ) h 0 This method is known as differentiation by first principles, and determines the slope between A and B as AB collapses to a point (A) x=0+2sin(2pi*t/14)+2cos(2pi*t/14) 4 3 2 1 0 x x -1 1 3 5 7 9 11 13 15 17 19 -2 -3 -4 t 16

2.1.2 Rules of Derivatives -although first principles always work, the following rules are more economical: 1) Constant Rule If f(x)=k (k is a constant), f (x) = 0 2) General Rule If f(x) = ax+b (a and b are constants) f (x) = a 17

2.1.2 Examples of Derivatives 1) Constant Rule If f(x)=27 f (x) = 0 2) General Rule If f(x) = 3x+12 f (x) = 3 18

2.1.2 Rules of Derivatives 3) Power Rule If f(x) = kxn, f (x) = nkxn-1 4) Addition Rule If f(x) = g(x) + h(x), f (x) = g (x) + h (x) 19

2.1.2 Examples of Derivatives 3) Power Rule If f(x) = -9x7, f (x) = 7(-9)x7-1 =-63x6 4) Addition Rule If f(x) = 32x -9x2 f (x) = 32-18x 20

2.1.2 Rules of Derivatives 5) Product Rule If f(x) =g(x)h(x), f (x) = g (x)h(x) + h (x)g(x) -order doesn t matter 6) Quotient Rule If f(x) =g(x)/h(x), f (x) = {g (x)h(x)-h (x)g(x)}/{h(x)2} -order matters -derived from product rule (implicit derivative) 21

2.1.2 Rules of Derivatives 5) Product Rule If f(x) =(12x+6)x3 f (x) = 12x3 + (12x+6)3x2 = 48x3 + 18x2 6) Quotient Rule If f(x) =(12x+1)/x2 f (x) = {12x2 (12x+1)2x}/x4 = [-12x2-2x]/x4 = [-12x-2]/x3 22

2.1.2 Rules of Derivatives 7) Power Function Rule If f(x) = [g(x)]n, f (x) = n[g(x)]n-1g (x) -work from the outside in -special case of the chain rule 8) Chain Rule If f(x) = f(g(x)), let y=f(u) and u=g(x), then dy/dx = dy/du X du/dx 23

2.1.2 Rules of Derivatives 7) Power Function Rule If f(x) = [3x+12]4, f (x) = 4[3x+12]33 = 12[3x+12]3 8) Chain Rule If f(x) = (6x2+2x)3 , let y=u3 and u=6x2+2x, dy/dx = dy/du X du/dx = 3u2(12x+2) = 3(6x2+2x)2(12x+2) 24

2.1.2 More Exciting Derivatives 1) Inverses If f(x) = 1/x= x-1, f (x) = -x-2=-1/x2 1b) Inverses and the Chain Rule If f(x) = 1/g(x)= g(x)-1, f (x) = -g(x)-2g (x)=-1/g(x)2g (x) 25

2.1.2 More Exciting Derivatives 2) Natural Logs If y=ln(x), y = 1/x -chain rule may apply If y=ln(x2) y = (1/x2)2x = 2/x 26

2.1.2 More Exciting Derivatives 3) Trig. Functions If y = sin (x), y = cos(x) If y = cos(x) y = -sin(x) -We see this relationship graphically: 27

2.1.2 More Derivatives Reminder: derivatives reflect slope: Sine(blue) and Cosine(red) 1.5 1 sin(x),cos(x) 0.5 0 1 3 5 7 9 11 13 15 17 19 21 23 25 27 -0.5 -1 -1.5 x 28

2.1.2 More Derivatives 3b) Trig. Functions Chain Rule If y = sin2 (3x+2), y = 2sin(3x+2)cos(3x+2)3 Exercises: y=ln(2sin(x) -2cos2(x-1/x)) y=sin3(3x+2)ln(4x-7/x3)5 y=ln([3x+4]sin(x)) / cos(12xln(x)) 29

2.1.2 More Derivatives 4) Exponents If y = bx y = bxln(b) Therefore If y = ex y = ex 30

2.1.2 More Derivatives 4b) Exponents and chain rule If y = bkx y = bkxln(b)k Or more generally: If y = bg(x) y = bg(x)ln(b) X dg(x)/dx 31

2.1.2 More Derivatives 4b) Exponents and chain rule If y = 52x y = 52xln(5)2 Or more complicated: If y = 5sin(x) y = 5sin(x)ln(5) * cos(x) 32

2.1.2.1 Higher Order Derivatives -First order derivates (y ), show us the slope of a graph -Second order derivatives measure the instantaneous change in y , or the slope of the slope -or the change in the slope: -(Higher-order derivates are also possible) 33

2.1.2.1 Second Derivatives Here the slope increases as t increases, transitioning from a negative slope to a positive slope. x=15-10t+t*t 10 5 0 x x 1 2 3 4 5 6 7 8 -5 -10 -15 t A second derivative would be positive, and confirm a minimum point on the graph. 34

2.1.2.1 Second Derivatives Here, the slope moves from positive to negative, decreasing over time. x=15+10t-t*t 50 40 30 x x 20 10 0 1 2 3 4 5 6 7 8 t A second derivative would be negative and indicate a maximum point on the graph. 35

2.1.2.1 Second Order Derivatives To take a second order derivative: 1) Apply derivative rules to a function 2) Simplify if possible 3) Apply derivative rules to the answer to (1) Second order differentiation can be shown a variety of ways: a)d2y/dx2 c)f (x) e) y b) d2f(x)/dx2 d) fxx(x) 36

2.1.2.1 Second Derivative Examples y=12x3+2x+11 y =36x2+2 y =72x y=sin(x2) y =cos(x2)2x y =-sin(x2)2x(2x)+cos(x2)2 y =-cos(x2)2x(4x2)-sin(x2)8x-sin(x2)2x(2) =-cos(x2)8x3-sin(x2)12x 37

2.1.2.2 Implicit Differentiation So far we ve examined cases where our function is expressed: y=f(x) ie: y=7x+9x2-14 Yet often equations are expressed: 14=7x+9x2-y Which requires implicit differentiation. -In this case, y can be isolated. Often, this is not the case 38

2.1.2.2 Implicit Differentiation Rules 1) Take the derivative of EACH term on both sides. 2) Differentiate y as you would x, except that every time you differentiate y, you obtain dy/dx (or y ) Ie: 14=7x+9x2-y d(14)/dx=d(7x)/dx+d(9x2)/dx-dy/dx 0 = 7 + 18x y y =7+18x 39

2.1.2.2 Implicit Differentiation Examples Sometimes isolating y requires algebra: xy=15+x y+xy =0+1 xy =1-y y =(1-y)/x 40

2.1.2.2 Implicit Differentiation Examples x2-2xy+y2=1 d(x2)/dx+d(2xy)/dx+d(y2)/dx=d1/dx 2x-2y-2xy +2yy =0 y (2y-2x)=2y-2x y =(2y-2x) / (2y-2x) y =1 41

2.1.2.2 Implicit Differentiation Examples Using the implicit form has advantages: 3x+7y8=18 3+56y7y =0 56y7y =-3 y =-3/56y7 vrs. y=[(18-3x)/7)1/8 y =1/8 * [(18-3x)/7)-7/8 * 1/7 * (-3) Which simplifies to the above. 42

2.2.1 Derivative Applications - Graphs Derivatives can be used to sketch functions: First Derivative: -First derivative indicates slope -if y >0, function slopes upwards -if y <0, function slopes downwards -if y =0, function is horizontal -slope may change over time -doesn t give shape of graph 43

2.2.1 Positive Slope Graphs Linear, Quadratic, and Lin-Log Graphs 120 100 80 60 40 20 0 1 2 3 4 5 6 7 8 9 10 44

2.2.1 Derivative Applications - Graphs Next, shape/concavity must be determined Second Derivative: -Second derivative indicates concavity -if y >0, slope is increasing (convex) -if y <0, slope is decreasing (concave, like a hill or a cave) -if y =0, slope is constant (or an inflection point occurs, see later) 45

2.2.1 Sample Graphs x = 2, slope is increasing; graph is convex x=15-10t+t*t 10 5 0 x x 1 2 3 4 5 6 7 8 -5 -10 -15 t 46

2.2.1 Sample Graphs x =-2, slope is decreasing; graph is concave x=15+10t-t*t 50 40 30 x x 20 10 0 1 2 3 4 5 6 7 8 t 47

2.2.1 Derivative Applications - Graphs Maxima/minima can aid in drawing graphs Maximum Point: If 1) f(a) =0, and 2) f(a) <0, -graph has a maximum point (peak) at x=a Minimum Point: If 1) f(a) =0, and 2) f(a) >0, -graph has a minimum point (valley) at x=a 48