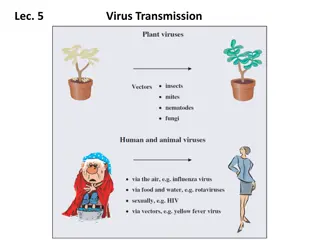
Vectors and Velocity
Vectors in space specified by three numbers, position vectors, adding vectors algebraically, notation, velocity concepts, 2-D coordinates, 1D velocity, graphing velocity, position to velocity ratio. Explore how direction and displacement relate to velocity over time.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
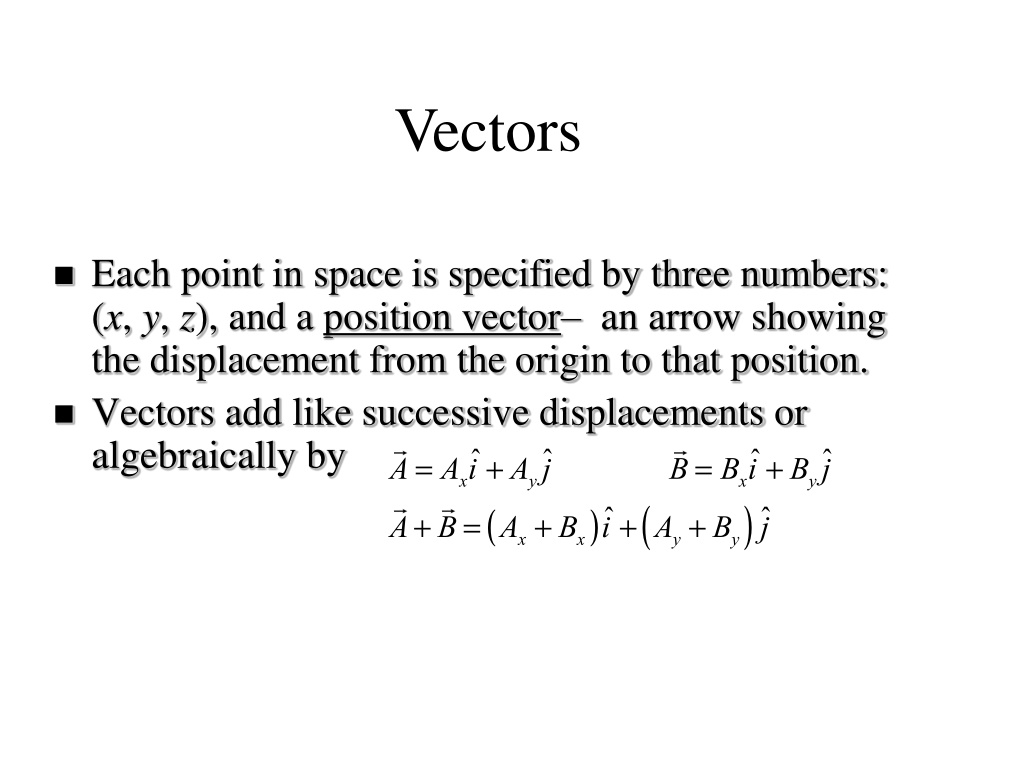
Vectors Each point in space is specified by three numbers: (x, y, z), and a position vector an arrow showing the displacement from the origin to that position. Vectors add like successive displacements or algebraically by A = Ax i + Ay j A+ B = Ax+ Bx B = Bx i + By j ) i + Ay+ By ( ) j (
Notation We specify the directions we are talking about by drawing two little arrows of unit length in two perpendicular directions. x and y are called the coordinates and can be positive or negative. Note that if x is negative, it means is a vector pointing in the direction opposite to r = x i + y j j i x i i
Velocity Average velocity is defined by v =Dr time it took to do it Note: an average velocity goes with a time interval. Dt=vector displacement Instantaneous velocity is what we get when we consider a very small time interval (compared to times we care about) r d v = Note: an instantaneous velocity goes with a specific time. dt
Vectors (2-D coordinates) We have 2 directions to specify. We must Choose a reference point (origin) Pick 2 perpendicular axes (x and y) Choose a scale We specify our x and y directions by drawing little arrows of unit length in their positive direction. A position vector (displacement from the origin) is written i , j i , x and y have units, do not. j
1D Velocity Velocity is the rate of change of position Average velocity = (how far did you go?)/(how long did it take you?) v =Dx Dt Instantaneous velocity = same (but for short t) v =dx dt
Graphing velocity: Figuring it out from the position Slope = <v> You can figure out the velocity graph from the position graph using v =Dx Dt x Dx = v Dt t Position as a function of time Velocity as a function of time 10 2 x (m) 8 1.5 6 x (m) 1 4 0.5 2 0 0 -5 0 5 10 15 -5 0 5 10 15 t (sec) t (sec) 9/9/15 6
Position to velocity x (m) v (m) dx dt v t (sec) t (sec) Ratio of change in position that takes place to the (small) time interval dx dt x t ( = v t ( ) + - D - D D ) x t ( ) t t Difference of two positions at two (close) times 2 2 = v t ( ) t 9/9/15
Recall: Vectors (2-D coordinates) We have 2 directions to specify. We must Choose a reference point (origin) Pick 2 perpendicular axes (x and y) Choose a scale We specify our x and y directions by drawing little arrows of unit length in their positive direction. A position vector (displacement from the origin) is written i , j i , x and y have units, do not. j
Vector velocity and speed A displacement a change in position has a direction. This means velocity = displacement/time interval has one too. dt vx i +vy j =d v =dr ( )= i + j dx dt dy dt x i + y j dt We define speed as the magnitude of velocity. (No vector on this. Why?) v = vx 2+vy 2
Velocity to position x (m) v (m) dx v t (sec) t (sec) dt change in position that takes place in a small time interval dt = ( ) dx v t dt sum ( ) in the changes in position over many small time intervals = = ( ) x dx v t dt 9/9/15
What have we learned? Representations and consistency Visualizing where an object is a position graph at different times Visualizing how fast an object is moving a velocity graph at different times Position graph velocity graph v =Dx v =dx slopes Dt dt areas Dx = v Dt Dx = vdt Velocity graph position graph
Vectors If a position vector (displacement from the origin) lies in each of the four indicated quadrants, what can you say about the vector representation of that displacement, 1. x and y are both positive 2. x and y are both negative 3. x is positive and y is negative 4. x is negative and y is positive y D A x C B