Unraveling the Fibonacci Sequence: Lessons in Mathematics & Nature

Join us on a journey through the enigmatic Fibonacci sequence with engaging lessons on its construction, exploration of mathematical patterns, observations of the golden spiral in nature, and creative activities linking to global goals. Discover the legacy of Fibonacci, the Italian mathematician, and delve into the intriguing world of number patterns and sequences.

• Fibonacci Sequence
• Mathematics
• Nature
• Golden Spiral
• Education

liel Follow

Uploaded on May 11, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

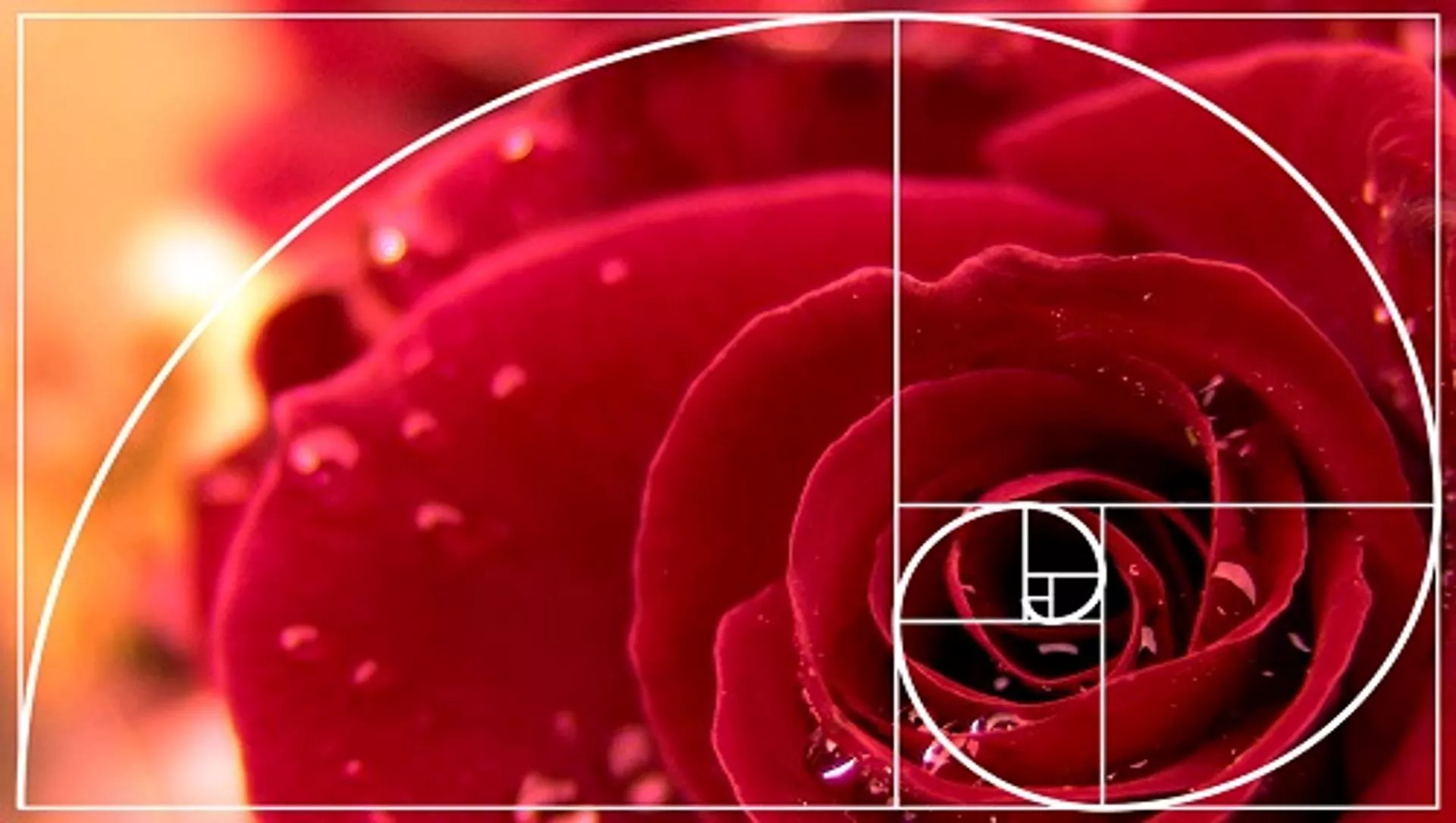
1. Fibonacci Whole Class Sequence Lesson One Introduction to Fibonacci, try to understand how the sequence is built, use addition strategies to build the sequence, visualise with counters/macaroni Lesson Two Consider the Fibonacci pyramid, look for the patterns, see what happens when you change the exterior numbers to 2s and 3s can you make links back to the F sequence? Lesson 3 Observe the golden spiral in nature, understand how the spiral is created, mark out small spiral on paper then take chalk and metre sticks outside Lesson 4 Attempt to create sketches that link to the global goals using the golden spiral template

2. Why this guy here is an absolute legend Fibonacci

3. Fibonacci was an Italian mathematician born in Pisa

4. He was born nearly 1000 years ago (long before calculators) Yet, he discovered a secret number pattern (I m telling you, this guy is a rockstar!)

5. Heres the sequence: 0 1 1 2 3 Can you figure out how it is created?

6. Can you continue the pattern? How many even numbers are there? How many odd numbers? Can you show it with macaroni?

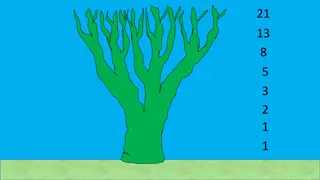
7. How many ways can you make 15 2 0 1 1 3 5 8 13 21

8. 1 This is a Fibonacci Pyramid. 1 1 1 2 1 1 3 3 1 1 4 6 4 1 Can you work out how it is created? What if you changed the 1s to 2s? What about 3s?

9. 1 1 1 2 1 1 1 2 1 1 3 3 1 1 4 6 4 1 3 5

15. Lets take it outside!

17. Using the golden ratio, can you create images that to the Global Goals? No: No: No: