Understanding Wave-Particle Duality in Physics
Explore the complex relationship between particles and waves in physics, as particles exhibit wave-like behavior described by wave packets rather than single waves. Delve into the concept of group velocity and phase velocity, and how a particle's behavior is represented by a wave function. Uncover the intricacies of wave-particle duality through insightful information presented by Dr. J.P. Singh, Associate Professor in Physics.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Wave packet Dr. J P Singh Associate Professor in Physics Post Graduate Govt. College Sector-11 Chandigarh
2 c / v De-broglie waves moves with velocity Which is much faster than c, therefore the wave associated with particle moves faster than particle itself leaving particle much behind, so it is clear that the particle can not be equivalent to a single wave train.
Motion of a particle is equivalent to a wave packet rather than a single wave. Wave packet is consists of group of waves, which have slightly different velocity and wave length. Phase and amplitude of these waves is so chosen that they interfere constructively over only a small region where particle is located. Outside of which they produce destructive interference so that amplitude become zero.
Particle behaviour should be described by the wave function (r,t), whose amplitude is max is where the probability of finding the particle is large and other region its magnitude is minimum.
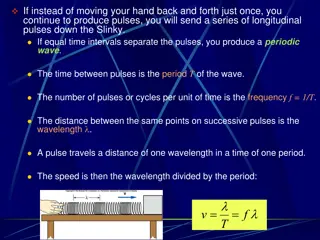
Group velocity With which a wave packet moves or the velocity with which the slowly varying envlope formed by group of waves moves in a medium. Phase velocity the velocity of individual de-broglie wave is called phase velocity and is more than the velocity of light.
= cos( ) y a t k x 1 1 1 = cos( ) y a t k x 2 2 2 = + y y y 1 2 = + cos( ) cos( ) y a t k x a t k x 1 1 2 2 + + = cos cos 2 cos cos 2 2 + 2 + 2 2 2 ( ) ( ) ( ) ( ) k k k k = 1 2 1 2 1 2 1 2 2 cos . cos y a t x t x
+ 2 k + 2 2 1 2 2 1 2 k 1 2 k = d 1 2 = k k dk 1 2 d dk = 2 cos( ). cos( ) y a t kx t x 2 2
This equation consists of two parts one is a wave of frequency and propagation vector k v Phase velocity or wave velocity = = v p 2 2 . = = 2 p 2 k Therefore this is the velocity of first part. The second part is another wave of frequency d /2 and propagation vector dk/2 and the group velocity / 2 d d = = vg / 2 dk dk
2 E 2 = . = 2 mc = 2 . h h 1 2 2 2 2 m c 2 m c v 2 = 0 = 0 1 2 h h c 2 v 1 2 c differentiating 3 2 2 . . 1 2 v v 2 2 2 = 1 d m c dv 0 2 2 h c c 2 m v = 0 . dv (1) d 3 h 2 v 2 1 2 c
2 2 2 2 m v = = = = 0 . k mv 1 / h p h h 2 v 2 1 2 c diff. 2 m dv = 0 . (2) dk 3 h 2 v 2 1 2 c dividing (1) by (2) d = = = v v v g p dk
2 2 p = = k h 2 dp h = = dp . dk 2 h dk 1 = + 2 2 2 0 4 E p c m c 2 = + 2 2 2 2 0 4 E p c m c . diff 2 = + 2 2 c . 0 EdE pdp = 2 EdE pc dp
Dividing by dk dE dp h 2 = = 2 2 . E pc pc dk dk 2 2 2 dE pc pc p mv = = = = = . v 2 h dk E mc m m 2 dE = = v v g h dk