Understanding Universal Instantiation in Deductive Reasoning
Universal instantiation is a crucial tool in deductive reasoning, allowing us to derive specific conclusions from general statements. By combining universal instantiation with modus ponens and modus tollens, we can construct valid arguments such as universal modus ponens and universal modus tollens. Examples illustrate the application of these rules, highlighting how they aid in recognizing valid forms of arguments. Diagrams are also used to test for validity, showcasing the distinction between valid and invalid reasoning based on the logical structure of statements.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
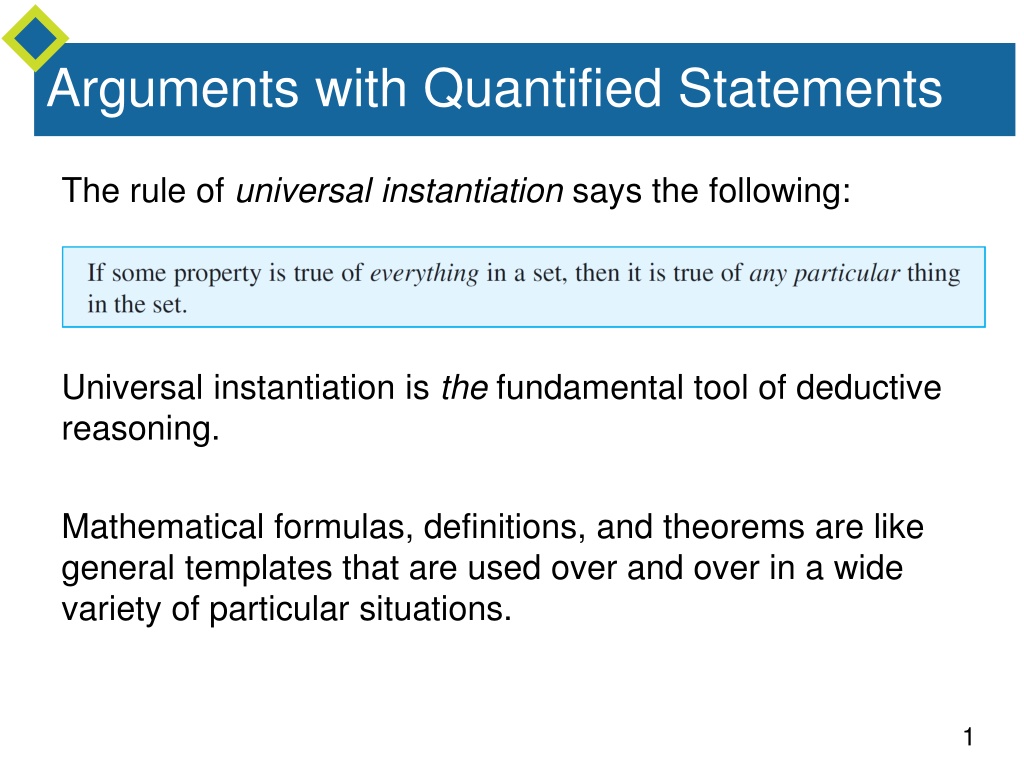
Arguments with Quantified Statements The rule of universal instantiation says the following: Universal instantiation is the fundamental tool of deductive reasoning. Mathematical formulas, definitions, and theorems are like general templates that are used over and over in a wide variety of particular situations. 1
Universal Modus Ponens The rule of universal instantiation can be combined with modus ponens to obtain the valid form of argument called universal modus ponens. 2
Example Recognizing the Form of Universal Modus Ponens Jim is mortal. All human beings are mortal. Jim is a human being. 3
Universal Modus Tollens Another crucially important rule of inference is universal modus tollens.Its validity results from combining universal instantiation with modus tollens. Universal modus tollens is the heart of proof of contradiction, which is one of the most important methods of mathematical argument. 4
Example Recognizing the Form of Universal Modus Tollens Zeus is not human. All human beings are mortal. Zeus is not mortal. 5
Example 6 Using Diagrams to Show Validity Use a diagram to show the invalidity of the following argument: All human beings are mortal. Jim is a human being. Jim is mortal. Use a diagram to show the invalidity of the following argument: All human beings are mortal. Felix is mortal. Felix is a human being. 6
Example 6 Solution cont d The conclusion Felix is a human being is true in the first case but not in the second (Felix might, for example, be a cat). Because the conclusion does not necessarily follow from the premises, the argument is invalid. 7
Using Diagrams to Test for Validity This argument would be valid if the major premise were replaced by its converse. But since a universal conditional statement is not logically equivalent to its converse, such a replacement cannot, in general, be made. We say that this argument exhibits the converse error. 8
Using Diagrams to Test for Validity The following form of argument would be valid if a conditional statement were logically equivalent to its inverse. But it is not, and the argument form is invalid. We say that it exhibits the inverse error. 9
Example 7 An Argument with No Use diagrams to test the following argument for validity: Jim is not a UNC student. No UNC student works for Duke. Jim works for Duke. 10
Using Diagrams to Test for Validity An alternative approach to this example is to transform the statement No UNC student works for Duke. into the equivalent form x, if x is a UNC student, then x does not work for Duke. 11
Using Diagrams to Test for Validity If this is done, the argument can be seen to have the form where P(x)is x is a UNC student and Q(x)is x does not work for Duke. Use Jim substitute a , ~Q(a): Jim works for Duke. This is valid by universal modus tollens. 12
Creating Additional Forms of Argument Consider the following argument: This argument form can be combined with universal instantiation to obtain the following valid argument form. 13