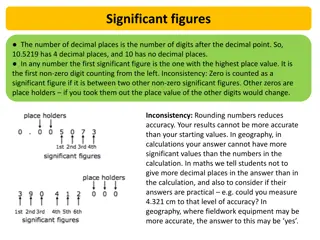
Understanding Significant Figures in Precision Measurements

Significant figures, often referred to as Sig Figs, play a vital role in conveying the precision of measurements. They help indicate the certainty of a measurement and guide in understanding the level of accuracy. Following rules like non-zero digits being significant, trapped zeros, insignificant leading zeros, and significant trailing zeros are essential in determining the correct number of significant figures in a measurement.

• Precision
• Measurement
• Significant Figures
• Sig Figs
• Accuracy

braden Follow

Uploaded on Jul 12, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. SIGNIFICANT SIGNIFICANT FIGURES FIGURES AMOLE AMOLE 2015 2015

2. WHAT & WHY? WHAT & WHY? Refer to them as Sig Figs for short Refer to them as Sig Figs for short Used to communicate the degree of Used to communicate the degree of precision measured precision measured Example Example - - Scientists records: 50 mL Scientists records: 50 mL Does that mean 50 mL exact? Could he only measure to the ones place? Did he round up from 49.8? Is it really 50.12 mL?

3. RULE #1: NON ZEROS RULE #1: NON ZEROS Every Every nonzero nonzero digit is significant. digit is significant. If it s not a zero, it will count If it s not a zero, it will count Examples: Examples: 24 = 2 sig figs 24 = 2 sig figs 3.56 = 3 sig figs 3.56 = 3 sig figs 7 = 1 sig figs 7 = 1 sig figs

4. RULE #2: CAPTURED ZEROS RULE #2: CAPTURED ZEROS Also called trapped or sandwiched Also called trapped or sandwiched zeros zeros Zeros Zeros between between non non- -zeros are significant Examples: Examples: 7003 = 4 sig figs 7003 = 4 sig figs 40.9 = 3 sig figs 40.9 = 3 sig figs 60.09 = ? 60.09 = ? zeros are significant

5. RULE #3: LEADING ZEROS RULE #3: LEADING ZEROS Zeros appearing in Zeros appearing in front are are not not significant significant Act as placeholders Act as placeholders Can t be dropped, show magnitude Can t be dropped, show magnitude Examples: Examples: 0.00024 = 2 sig figs 0.00024 = 2 sig figs 0.453 = 3 sig figs 0.453 = 3 sig figs 0.003 = ? 0.003 = ? front of non of non- -zero digits zero digits

6. RULE #4: TRAILING ZEROS RULE #4: TRAILING ZEROS Zeros at the Zeros at the end decimal decimal point are significant. point are significant. At the end and At the end and to the right of a decimal point to the right of a decimal point Examples: Examples: 43.00 = 4 sig figs 43.00 = 4 sig figs 1.010 = 4 sig figs 1.010 = 4 sig figs 1.50 = ? 1.50 = ? end of a number of a number with a with a

7. RULE #5: TRAILING ZEROS RULE #5: TRAILING ZEROS Zeros at the Zeros at the end decimal decimal point are point are not At the end and At the end and to the right of a decimal point to the right of a decimal point Examples: Examples: 300 = 1 sig figs 300 = 1 sig figs 27,300 = 3 sig figs 27,300 = 3 sig figs 120 = ? 120 = ? end of a number of a number without a not significant. significant. without a

8. All non All non- -zero digits DO count. zero digits DO count. Leading zeros DON T count. Leading zeros DON T count. (zeros in front of numbers) Captive Zeros DO count. Captive Zeros DO count. (zeros between non-zero numbers) Trailing Zeros DO count IF the number Trailing Zeros DO count IF the number contains a DECIMAL. contains a DECIMAL. (zeros at the end of numbers)

9. TRY THESE! = 4 sig. figs. = 3 sig. figs. = 6 sig. figs. = 6 sig. figs. = 1 sig. figs. = 4 sig. figs. = 5 sig. figs. = 4 sig. figs. 4.012 4.012 87,900 87,900 91.0005 91.0005 500,001 500,001 0.005 0.005 0.6010 0.6010 7,040, 100 7,040, 100 2.100 2.100

10. ADDING & SUBTRACTING ADDING & SUBTRACTING The answer cannot be more precise than the values in The answer cannot be more precise than the values in the calculation the calculation The answer is rounded off so it contains the same The answer is rounded off so it contains the same decimal places as the number in the problem with the decimal places as the number in the problem with the fewest . fewest . Example: 12.11 + 18.0 = Example: 12.11 + 18.0 = 30.11 30.11 12.11 = 2 decimal places 12.11 = 2 decimal places 18.0 = 1 decimal place 18.0 = 1 decimal place 12.11 + 18.0 = 30.1 12.11 + 18.0 = 30.1

11. YOU TRY: 2.140 + 0.023 = ? 2.140 + 0.023 = ? 2.140 = 3 decimal places 2.140 = 3 decimal places 0.023 = 3 decimal places 0.023 = 3 decimal places Answer unrounded: 2.163 Answer unrounded: 2.163 Answer with appropriate sig figs: 2.163 Answer with appropriate sig figs: 2.163

12. MULTIPLYING & DIVIDING MULTIPLYING & DIVIDING The answer cannot be more precise than the values in The answer cannot be more precise than the values in the calculation the calculation Answer should contain the same number of sig figs as Answer should contain the same number of sig figs as the number with the least sig figs in the problem: the number with the least sig figs in the problem: Example: 4.56 x 1.4 = Example: 4.56 x 1.4 = 6.38 4.56 = 3 4.56 = 3 1.4 = 2 1.4 = 2 4.56 x 1.4 = 6.4 4.56 x 1.4 = 6.4 6.38

13. YOU TRY: 1.20 x 0.51 = ? 1.20 x 0.51 = ? 1.20 = 3 1.20 = 3 0.51 = 2 0.51 = 2 Answer unrounded: 0.612 Answer unrounded: 0.612 Answer with appropriate sig figs: 0.61 Answer with appropriate sig figs: 0.61

14. TRY THESE! = 4.04 4.01 + 0.03 4.01 + 0.03 = 4.04 = 2.857 = 2.9 87.957 87.957 85.1 85.1 = 4.956 = 5.0 4.13 x 1.2 4.13 x 1.2 = 90.90909 = 90 500 / 5.5 500 / 5.5

15. PUTTING IT ALL TOGETHER (1.2 x 10 (1.2 x 103 3) x (3 x 10 ) x (3 x 104 4) = ? ) = ? (3.6 x 10 (3.6 x 107 7) / (4.0 x 10 ) / (4.0 x 105 5) = ? ) = ? (1.2 x 10 (1.2 x 10- -3 3) x (3 x 10 ) x (3 x 104 4) = ? ) = ? (3.6 x 10 (3.6 x 107 7) / (4.0 x 10 ) / (4.0 x 10- -5 5) = ? ) = ?