Understanding Optics: Refraction and Optical Fibres in Science
Explore the concepts of refraction and optical fibres in this comprehensive science lesson. Learn about refractive index, Snell's law, total internal reflection, and the role of cladding in optic fibres. Enhance your understanding of pulse broadening, absorption, and critical angles in optics through practical applications and assessments.
Uploaded on Sep 28, 2024 | 0 Views
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
5 Waves 3.3.2 Optics Animated Science 2015
Chapter Map Animated Science 2015
3.3.2.3 Refraction at a plane surface (5 hours) I can.... (tick off) 1. Define refractive index in terms wave speed in different media. 2. Recall that the refractive index of air is approximately 1. 3. Use Snell s law to calculate angles when light crosses a boundary between two media, 4. Describe total internal reflection and distinguish this from partial reflection. 5. Calculate critical angles using refractive indices. 6. Describe the step index optic fibre. 7. Understand the principles and consequences of pulse broadening and absorption. Assessment Practice.... PHYA1 May 2014 Q5 PHYA1 May 2013 Q5 PHYA1 Jan 2013 Q5 PHYA1 May 2012 Q4 PHYA1 Jan 2012 Q6 PHYA1 May 2011 Q5 Learning Objectives... Defining refractive index in terms of wave speed in different media. Snell s law of refraction at a boundary: ICT Links... http://www.learnerstv.com/animation/ani mation.php?ani=102&cat=physics Total internal reflection Step index optic fibres including the function of the cladding. http://hyperphysics.phy- astr.gsu.edu/hbase/geoopt/refr.html Material and modal dispersion and the consequences of pulse broadening and absorption. https://www.youtube.com/watch?v=0Mw MkBET_5I Stretch and Challenge... Complete to nm precision! Skills: AO1: Demonstration of knowledge and understanding of refractive index and its relationship to wave speed. AO1: Demonstration of knowledge and understanding of Snell s law. AO2: Apply knowledge and understanding of Snell s law in calculations. MS 0.6, 4.5 Use of sine. MS 4.1 Use of angles (incidence and refraction). MS 2.4 Solve algebraic equations to determine angles of refraction. MS3.2 and 3.4 Plot graph to determine refractive index. AO1: Demonstration of knowledge and understanding of total internal reflection and critical angle. AO2: Apply knowledge and understanding in calculations involving the critical angle. MS 2.4 Solve algebraic equations to determine critical angles. AO1: Demonstration of knowledge and understanding of optic fibres and the importance of cladding. AO1: Demonstration of knowledge and understanding of material and modal dispersions PS1.2 Apply scientific knowledge to explain the consequences of pulse broadening and absorption. I need further help with.... Animated Science 2015
Mr D Powell 2018 Animated Science 2018 5.3 TIR - Explaining how light moves through a medium when it reflects internally Appreciate that the higher the refractive index or optical density of a substance the more light will refract for the same entry angle. C When the angle of refraction is 90 or /2 then Snells law simplifies from n1sin 1 = n2sin 2 n1sin 1 = n2 (out of a prism) B Gain understanding of these key terms and be able to apply to an optical fibres exam question: Core, Cladding, coherence, Spectral Dispersion, Multipath Dispersion, Critical Angle (TIR), Mono-mode A Extension challenge question/task: Research in detail multimode fibres and step modes. Animated Science 2018
5.3 TIR When the angle of refraction is 90 or /2 then Snells law simplifies from n1sin 1 = n2sin 2 n1sin 1 = n2 (out of a prism) (AO1a) Appreciate that the higher the refractive index or optical density of a substance the more light will refract for the same entry angle. (AO2b) Gain understanding of these key terms and be able to apply to an optical fibres exam question : Core, Cladding, coherence, Spectral Dispersion, Multipath Dispersion, Critical Angle (TIR), Mono- mode (AO2b) AO3a Take precise practical readings to support the theories during the lesson. http://en.wikipedia.org/wiki/Optical_fiber Animated Science 2015
Starter. n1sin 1= n2sin 2 You have used this formula from previous lessons and should understand how it works. Can you demonstrate your knowledge of what it is used for? (5 marks) 2 = Sin40 * (1/1.33) = 0.48 2 = 28.9 1 = 40.0 Hint Try drawing the rays n1 = 1.00 Try an example calculation. n2 = 1.33 Extension: what happens when refracted ray is at 90 to normal. Animated Science 2015
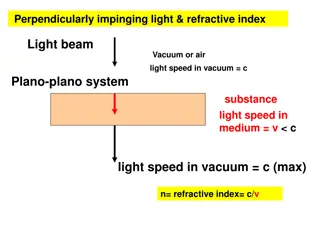
TIR weak reflected ray As the angle of incidence increases towards the critical angle ( glass = 420 ) the refracted ray gets weaker and the reflected ray gets stronger. NB: When light travels from an optically denser medium to a less dense medium, rays are bent away from the normal. The incident substance has a larger refractive index than the other substance Animated Science 2015
Critical Angle? Critical angle depends upon the refractive indices of the media ??????sin??????=????sin???? ??????sin??????=????sin90 ???? nair ???? ??????= C sin?????? ?????? nprism 1 ??????= sin?????? 1 sin??????= ?????? Animated Science 2015
Critical Angle Example? For Water 1 sin? = 1.33 2 AIR WATER C 1 C = 48.80 1 sin? = C = 41.80 For Crown Glass 1.50 Animated Science 2015
Diamonds Sparkle the most! 1 ?1 sin? = Diamond has a refractive index of 2.417. This means that colours are spread out more. TIR occurs many times inside the diamond before emerging. 24.44 Can you work out the critical angle? Animated Science 2015
Answers Refractive index n1 Refractive index n2 2 refracted 1 incident Material Water (tank) 1.33 1 90 49 Your Prism 1.39 1 (air) 90 46 Crown Glass 1.5 1 90 42 Diamond 2.417 1 90 24 Un cladded fibre optic 1.62 (core) 1 (air) 90 38 Cladded fibre optic 1.62 (core) 1.52 (clad) 90 70 1 sin? = ?????? ??????sin??????=????sin???? Animated Science 2015
Critical Angle? ?????? Critical angle depends upon the refractive indices of the media sin???? sin?????? ?????? sin90 sin?????? ?????? 1 sin?????? 1 sin? = C nprism = = 1 ??????= sin? 1 ?????? Filter Colour Critical Angle Refractive Index ??????= sin? = None (white) 1 46 1.39 ? = sin 1 ?????? Red 46.5 1.378 Green 46 1.39 Blue 45.5 1.40 Animated Science 2015
Basics of the Fibre Light can enter in a range of values which can lead to signals travelling a longer path length to the end of the fibre (multipath dispersion). This can cause merging of the signal from pulse to pulse. The typical refractive value for the cladding of an optical fibre is 1.52. The core value is typically 1.62. The larger the index of refraction, the slower light travels in that medium. The idea of the core is to produce TIR at a larger angle so rays entering at a larger angle will be passed down the fibre. Use of white light can also cause problems (spectral dispersion) for the same reasons. However, in this case the light turns into a spectrum at the other end. The material causes different wavelengths to spread out. n= 1.62 n= 1.52 A solution to the dispersion is to make the fibre very thin 5 m in diameter to reduce the effect. (Monomode) Animated Science 2015
Cladding Calculation. An optical fibre of refractive index n =1.62 is surrounded initially in air n = 1. Using the critical angle formulae it can be shown that ??sin??=????sin???? ??sin??=???? 1 ??= sin 1???? c = 38.1 However, if n increases from 1 to 1.52 by adding cladding to the fibre ?? = sin 1????? ?? ?? c = 69.76 So the cladding increases the critical angle between the two materials. n= 1.52 n= 1.62 Animated Science 2015
Cladding Calculation. So if the cladding increases the critical angle between the two materials. The benefits of this are: only those rays that are close to the axis of the fibre pass through the inner fibre is protected from damage the rays all travel roughly the same distance and so information fed in at one end arrives at the other only very slightly spread out in time there are fewer reflections and the distance travelled is smaller than the multiple reflection case and so there is less energy loss and the time of transmission is shorter n= 1.52 n= 1.62 Animated Science 2015
Extras.. https://www.cyberphysics.co.uk/graphics/diagrams/light/cable_block.gif Animated Science https://www.cyberphysics.co.uk/topics/light/FiberOptics/FibreOptics.htm 2015
Cladding and multipath dispersion (More Detail) The fibres are coated with a glass of slightly lower refractive index. This is known as cladding. The cladding increases the critical angle within the core fibre and also prevents adjacent fibres from touching each other. At every point of contact light would escape into another fibre. The fewer the reflections the less energy loss, and the shorter the time of transfer of information down the fibre since the light travels a shorter distance. Initially it would seem that the addition of the cladding would allow light to escape into the surroundings. This is indeed the case but the cladding has another purpose. It means that only the light that makes a small angle with the axis of the fibre is transmitted over large distances. The difference in the time of travel between the individual light rays is therefore smaller and so the spread of information (known as multipath dispersion) is also reduced. n= 1.62 n= 1.52 Animated Science 2015
Applications of Fibre Optics A non-coherent fibre bundle, as you would expect, does not have this precise matrix alignment since they need only transmit light for illumination purposes. They are cheaper to produce. Christmas Tree. A coherent bundle of optical fibres in which the relative spatial coordinates of each fibre are the same at the two ends of the bundle. Such a bundle are used for the transmission of images. Endoscope (stick of rock!) https://www.youtube.com/watch?v=0ButqZV-xJI Animated Science 2015
More Uses of Optical Fibres. 1. Illuminating models or road signs using only one bulb 2. Endoscopy - seeing down inside a patient s body 3. Communications sending information along a light beam. Useful for telephone, television, radio, computer networks, stereo links, control in aircraft 4. Security fencing very difficult to bypass 5. Fibre optic lamp Advantages of fibre optics over copper wire 1. 2. 3. Cheap glass is made from silica, the basic constituent of sand Light in weight useful in aircraft Light beam can carry a huge amount of information Such fibres can be made to carry information such as TV channels or telephone conversations. Other applications of fibre optics include its use in medicine to see inside the human body and in road signs where one light bulb and a set of fibres is used to illuminate different parts of the sign thus saving electrical energy. A further recent application is in security fences. The metal strands of the fence contain a piece of fibre optic material down which a beam of light passes. If the strand is cut the light beam is interrupted and an alarm sounds. It is thought that this type of system is impossible to bypass. Animated Science 2015
Key Points. Fibre optic cables can carry signals with much less energy loss than copper cable as copper wires lose signal energy as heat (P=I2R) due to their resistance. They are much lighter than copper cables with the same band width, so much less space is required in underground cabling ducts and costs for transportation and handling are therefore less. They are immune to electromagnetic interference from radio signals, lightning etc They can be routed safely through explosive or flammable atmospheres where are the risk of sparks from electrical cables would be to great for them to be used without a lot of precautions taken. The raw materials to make them are plentiful (silicon from sand!) whereas copper supplies are dwindling. Optical fibres are still more expensive per metre than copper because they are manufactured to a high standard and are made to carry multiple signals. They cannot be joined together as easily as copper cable Animated Science 2015
Extension Animated Science 2015
Feature Purpose / Related Physics This is the angle at which then a light ray incident on a boundary becomes great enough to it will reflect off the internal surface of the material. Total Internal Reflection Critical Angle (TIR) Narrow flexible fibre of glass designed to make light TIR at the inside surface. It has a high refractive index to make sure the maximum amount of light is transmitted. Core The cladding has a lower refractive index that the core. It is there to ensure that if adjacent fibres touch there is no transmission of light. Also as a change of index to increase the angle at which TIR takes place and keep the light in at greater angles. The cables in the endoscope specifically must form a pattern or image of the patient. Hence we need to make sure that the same pattern is show at the top and bottom of the bundle Cladding Endoscopes coherence Multipath (Modal) Dispersion Reduction of the size of the core means that there is a limit to the pathways that light can travel leading to a single mode or mono-mode of travel. Light can enter in a range of values which can lead to signals travelling a longer path length to the end of the fibre (multipath or material dispersion). This can cause merging of the signal from pulse to pulse. Use of one prevents this being a problem. Mono mode fibres are made very thin to 5 m in diameter to reduce the effect of spectral and multipath dispersion (broadening). Spectral (Material) Dispersion Mono-mode Animated Science 2015
Acceptance Angle in Fibre Optics Extension (Not required) The acceptance angle of an optical fibre is defined based on a purely geometrical consideration (ray optics). It is the maximum angle of a ray (against the fibre axis) hitting the fibre core which allows the incident light to be guided by the core. Where . is the angle the ray makes with the fiber axis, before entering the fiber The sine of that acceptable angle is called the numerical aperture, and it is essentially determined by the refractive index contrast between core and cladding of the fibre, assuming that the incident beam comes from air or vacuum: nois the refractive index along the central axis (core) of the fiber ncis the refractive index of the cladding. Animated Science 2015
Animated Science 2015
Animated Science 2015
NB: so the higher n of the glass gives a smaller critical angle Animated Science 2015
Animated Science 2015
Animated Science 2015
sin??=?1 The critical angle formulae only works for when n1 > n2 ?2 Hence this only occurs for glass film and not other way around Animated Science 2015
Animated Science 2015
Animated Science 2015
Animated Science 2015
Refraction Summary / Revision Main idea is... That the angle of incidence increases the angle of refraction will also increase. i The theory of this is that light is slowed down by a prism due to an increase in optical density in comparison with air. c = f so the theory is that if reducesthen so will c as the frequency or colour is a constant through the prism. Light enters a prism It slows down Turns towards the normal This process is called refraction We can measure the incident angle (i) We can measure the refracted angle (r) We find the ratio of the sine function of each gives us a consistent value for a material. This is the refractive index of a substance NB: When light travels from an optically denser medium to a less dense medium, rays are bent away from the normal. If the incident substance has a larger refractive index than the other substance i.e. Air -> prism. This reverses on the way out and bends towards the normal. sin( ) i = n sin( ) r Light enters a prism at the critical angle. c The angle of refraction becomes 90 Ratio sin formula simplifies as sin 90 = 1 The refractive index n can be worked out from 1 angle instead of 2. If the light has a different wavelength then it refracts or slows down differently in the prism. Blue refracts the most so the critical angle is lower in the same prism. Example Material n Vacuum 1 Air 1.00029 Filter Colour None (white) Red Green Blue Critical Angle Refractive Index Wavelength / x 10-6m Carbon dioxide 1.0005 Diamond 2.419 1 46 1.39 - = nprism Cubic Zirconia 2.15 46.5 46 45.5 1.378 1.39 1.40 0.62 0.53 0.41 sin C Water ice 1.31 Prism ? cornea (human) 1.3375 Animated Science 2018