Understanding Nuclear Reaction Rates and Cross Sections

Nuclear reaction rates and cross sections play a crucial role in determining the probability of interactions between particle beams and target nuclei. Cross section is the effective area of a target nucleon to the incident beam, with the interaction probability calculated based on the number density of particles in the beam and target. The total area of interaction and resulting reaction rate depend on the flux density and characteristics of the beam-target system. Attenuation and mean free path further contribute to understanding the behavior of beams traversing through materials.

• Nuclear reactions
• Cross sections
• Interaction probability
• Attenuation
• Mean free path

berkley Follow

Uploaded on Aug 03, 2024 | 1 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

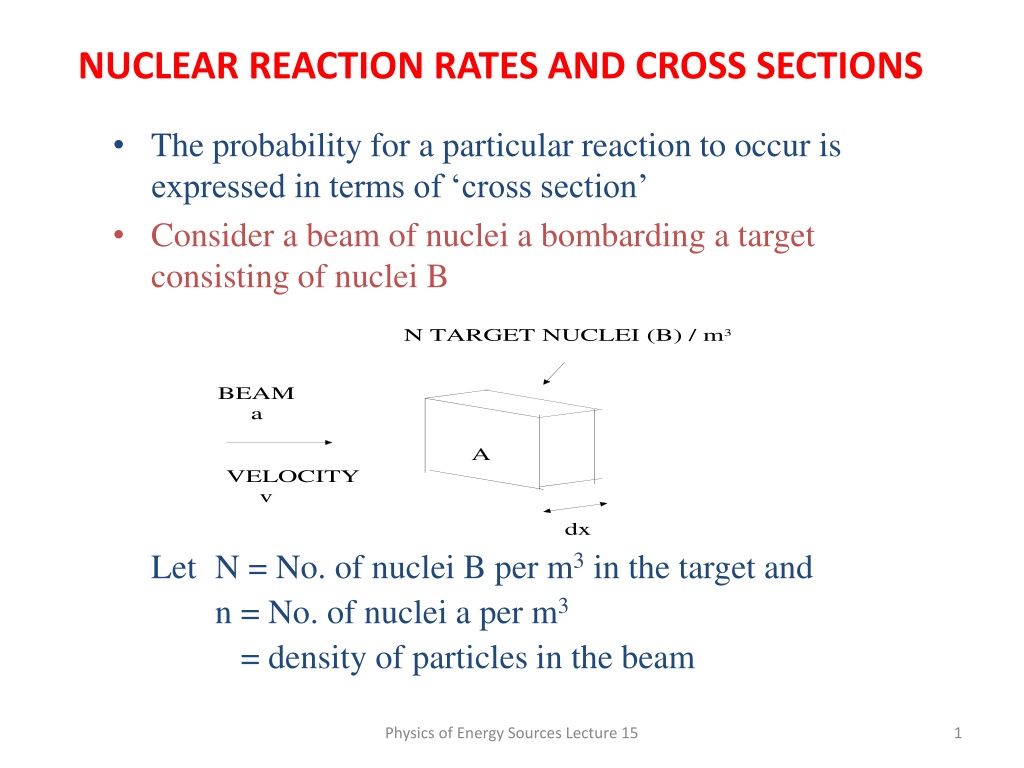
1. NUCLEAR REACTION RATES AND CROSS SECTIONS The probability for a particular reaction to occur is expressed in terms of cross section Consider a beam of nuclei a bombarding a target consisting of nuclei B N TARGET NUCLEI (B) / m3 BEAM a A VELOCITY v dx Let N = No. of nuclei B per m3in the target and n = No. of nuclei a per m3 = density of particles in the beam Physics of Energy Sources Lecture 15 1

2. The CROSS SECTION is the effective area of a target nucleon to the incident beam E.g. For billiard ball scattering of a point particle by spherical targets of radius r = r2 Other scattering processes are more complex but the idea is the same In an area A of target material of thickness dx there will be N A dx nucleons Total area offered to one beam particle to generate an event = N A dx Interaction ProbabilityP = N A dx / A = N dx(15.1) The beam flux density is the number of particles crossing a unit area per second = = v n Total number of interactions per second = reaction rate R (15.2) Physics of Energy Sources Lecture 15 2

3. R = (Number of beam particles / second) x (interaction probability) = ( A) x P R = A N dx = N dV or R = N per unit volume In this formula A is the area of interaction between beam and target. It may be defined by either target dimensions or beam dimensions Note that the assumption is that the same flux traverses the whole target, i.e. very few beam particles interact This is the thin target approximation. Cross sections are measured in BARNS 1 b = 10-28 m2 For nuclear power typical values :- 10-2 b < <10+3 b (15.3) Physics of Energy Sources Lecture 15 3

4. EXAMPLE: The total cross section for a 2 MeV neutron in 235U is 7.7 b. What is the probability of an interaction if the target is 1 mm thick ? [235U has a density = 19000 kg m-3] Number density for 235U is N = 19000/(235 x 1.66 10-27) = 4.84 1028 m-3 P = N dx = 4.84 1028 x 7.7 10-28 x 10-3 = 0.037 i.e. There is about 4 % chance of an interaction. However the cross section for thermal neutrons is ~ 690 b ! So what happens then?? Physics of Energy Sources Lecture 15 4

5. ATTENUATION Consider the loss of flux in a beam traversing a distance x of material Flux x dx The loss of flux d in a beam traversing a thickness dx is given by the interaction rate d = d x A = - R = - A N dx so d / = - N dx & integrating = exp( - N x) where is the flux density at x = 0 (15.4) MEAN FREE PATH The Mean Free Path is the average distance between interactions Probability of a beam particle interacting between x and x + dx is P(x) = (Probability of traversing x) x (Probability of interacting in dx) Physics of Energy Sources Lecture 15 5

6. P(x) =exp( - N x) . N dx 0 0 ( ) exp( ) xP x dx xN N x dx = = 0 0 ( ) exp( ) P x dx N N x dx 0 0 [ exp( )] exp( ) x N x N x dx = 0 [ exp( )] 1 N x 1 = = N = 1 / N = 1 / (15.5) where = = N is the MACROSCOPIC CROSS-SECTION We can rewrite the flux attenuation as = exp( - x / ) or = exp( - x ) or = exp( - x ) where is the ABSORPTION COEFFICIENT For a mixture = = N1+ N2+ N3+... (15.6) Physics of Energy Sources Lecture 15 6

7. The intensity of a beam of thermal neutrons is attenuated by a factor 10-6 by means of the (n, ) reaction in a plate of natural boron of thickness 1.4mm. Calculate the macroscopic cross section for natural boron. The composition by mass of natural boron is 20% of 10B and 80% of 11B. The interaction of thermal neutrons with 11B is negligibly small. Calculate the microscopic (n, ) cross section for thermal neutrons incident on 10B. (The density of natural boron is 2.3 x 103 kg m-3) I = I0 exp(- x) so 10-6 = exp(-1.4 10-3 x ) Then = 9868 m-1 Average mass No. of Boron = 0.2*10+0.8*11 = 10.8 No. atoms per cubic metre is N = 2.3 103 / (10.8 x 1.66 10-27) = 1.28 1029 m-3 (natural boron) = 0.2 x (10B) + 0.8 x (11B) = 0.2 x (10B) = 9868 = 1.28 x1029 x 0.2 x (10B) Hence (10B) = 3.855 x 10-25 m2 = 3855 b Physics of Energy Sources Lecture 15 7

8. RADIOACTIVE DECAY Basic assumption is that nuclear decay is a RANDOM process The probability for ONE nucleus to decay per unit time, , is a constant i.e. p1(t) = (1/N) dN/dt = - = constant so dN/dt = - N (15.7) (-ve sign because N decreases due to decay) N = N(t) is the number of radioactive nuclei at time t N0 = N(0) = initial number at t=0 RADIOACTIVE DECAY N t dN 0 = dt N 1.5 N N(T) / N(0) 0 1 = (15.8) log = log N N t Series1 0 e e 0.5 exp( ) N N t o 0 For N nuclei the probability of decay P(t) = N p1(t) = - N 0 1 2 3 (15.9) TIME IN MEAN-LIVES Physics of Energy Sources Lecture 15 8

9. MEAN LIFETIME Define the mean lifetime in terms of the probability function P(t) as follows:- 0 0 ( ) tP t dt tN dt = = 0 0 ( ) P t dt N dt 0 t N te dt 0 = 0 t N e dt 0 t 1 (15.10) = = N N e 0 Define HALF LIFE T1/2 as time taken for radioactive nuclei to decay N.B. ACTIVITY = dN/dt = - N =-N/ is easier to measure T N 1 / 2 = e N 0 (15.11) = = ln( 2 ) . 0 693 T 1 / 2 (15.12) Physics of Energy Sources Lecture 15 9

10. FORMS OF DECAY -DECAY Occurs in heaviest nuclei with Z > 83 e.g. 238U 234Th + 216Rn 212Po + T1/2 ~ 4x109 yrs T1/2 ~ 4.5 s -DECAY AND eK CAPTURE T1/2 ~ 11 min Mg + + + 14 n p e.g. + 27 12 27 13 * Al T1/2 ~ 9 min 15 N.B. The * indicates an excited state and the bar indicates an antiparticle (anti-neutrino) and +e.g 13 14 Si K-capture is when an electron is captured from the K-shell of an atom. Similar to positron emission, Z Z-1, N N+1 and energetically more favourable e.g. + 3 4 Be eK Physics of Energy Sources Lecture 15 T1/2 ~ 4 sec + + + 27 27 13 * 14 Al + 7 7 3 * 4 Li T1/2 ~ 53 days 10

11. -DECAY Nuclei left in excited states e.g. 27Al*, decay to their ground states by emitting -rays with < 10-12 s A* E=h A FISSION Some nuclei heavier than uranium can fission spontaneously e.g. + + 242 96 148 58 92 38 2 Cm Ce Sr n 146 90 54 T1/2 ~ 53 days Many other channels Physics of Energy Sources Lecture 15 11

12. NUCLEAR STABILITY CURVE Z=N 120 decay + or eK Pb 80 Region of stable nuclei Z 40 - decay 50 100 150 N Heavy nuclei contain more neutrons than protons Nuclear forces are the same Coulomb forces reduce the binding energy of protons Radioactive nuclei lie outside the region of stability. A parent decays to a daughter Multiple decays may occur to reach the stable region Physics of Energy Sources Lecture 15 12

13. Example of the decay chain for 238U 234U 210Po 206Pb 214Bi 206Tl210Pb214Bi218Po 206Hg 222Rn 214Pb 230Th 234Pa 238U 226Ra 214Po 234Th This sequence concludes with the stable isotope 206Pb Fission produces neutron rich products e.g. 238U146 / 2 119Pd73 (Asymmetry likely) The only stable nucleus with A=119 is 119Sn69 So several - decays are needed to reach stability Physics of Energy Sources Lecture 15 13