Understanding Normal Distribution in Statistical Problems
Explore various scenarios involving normal distributions, such as calculating probabilities, determining population percentages, estimating standard deviations, and analyzing ranges of values. Practical examples include math test scores, rattlesnake lengths, car speeds, jelly bean quantities, and more.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
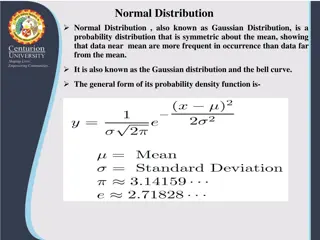
The normal The normal distribution distribution
1 Scores in a maths test are normally distributed with a mean of 64% and a standard deviation of 10%. Find the probability that a randomly chosen student scores more than the mean. 0.5 0.64 0.36 0.5
2 A population has a normal distribution with a mean ? and a standard deviation ?. The percent of the population that lies between ? 2? and ? + ? is: 47.5 95.4 68.3 81.5
3 The lengths of rattlesnakes are normally distributed with a mean of 100cm and a standard deviation of 10cm. The number of rattlesnakes whose length is less than 95cm is equal to the number of rattlesnakes whose length is: > 105 < 100 > 95 < 105
4 On a stretch of highway a speed camera has recorded the speeds of all the passing cars. The speeds are found to be normally distributed with a mean of 108km/h and a standard deviation of 15km/h. A car is chosen at random, find the probability that the speed of the car is within 1 standard deviation of the mean. 0.333 0.667 0.682 0.318
5 The continuous random variable ? ?(100,?2). It is given that ?(92 < ? < 108) 0.95. Estimate the standard deviation of ?. 4 16 8 64
6 Given ? ?(200,64). Find ?(? < 212). ?.???
7 Given ? ?(200,64). Find ?(? 194). ?.???
8 The number of jelly beans in a bag is normally distributed with a mean of 35 and a standard deviation of 4. If a bag of jelly beans is selected at random, the probability to the nearest hundredth, that there are between 37 and 45 jelly beans in the packet is: 0.50 0.30 0.48 0.70
9 The diagram represents ?~?(32,102). The shaded region is ? 32 < ? < 40 = ?. Calculate the value of ?. 8 0.0319 0.0716 0.288
10 On a stretch of highway a speed camera has recorded the speeds of all the passing cars. The speeds are found to be normally distributed with a mean of 108km/h and a standard deviation of 15km/h. A car is chosen at random, find the probability that the speed of the car has a speed of less than 100km/h. 0.340 0.297 0.660 0.703
11 The lifetime of a brand of kettles are normally distributed with a mean of 38 months and a standard deviation of 8 months. If a chain of stores sells 2800 kettles and gives an 18 month warranty, how many of them would be expected to fail before the warranty expires? 8 83 17 170
12Given ? ?(8,1.5). Find ?(? 0). ?
13Given ? ?(8,1.5). Find ?(6 < ? < 10). ?.???
14 On a stretch of highway a speed camera has recorded the speeds of all the passing cars. The speeds are found to be normally distributed with a mean of 108km/h and a standard deviation of 15km/h. 30% of the cars have a speed that exceeds ? km/h. Find the value of ? correct to 3sf. 115 116 71 100
15 The weight, ? grams, of a particular variety of orange is normally distributed with mean 205 and standard deviation 25. A wholesaler decides to grade such oranges by weight. He decides that the smallest 30 percent should be graded at small. Determine, to 1dp, the maximum weight of an orange graded as small. 218.1 218.0 191.8 191.9
16 An airline operates a service between Manchester and Paris. The flight time may be modelled by a normal distribution with mean 85 minutes and standard deviation 8 minutes. In order to gain publicity for the service, the airline decides to refund fares when a flight time exceeds ? minutes. Find the value of ? such that the probability of fares being refunded on a particular flight is 0.001. 61 105 110 55
17 The random variable G has a mean of 20.0 and a standard deviation of ?. It is given that ?(? > 15.0) = 0.6. Assume that G is normally distributed. Find the value of ?. 0.253 388 19.7 5
18 Batteries are produced at a factory. The batteries are normally distributed with a mean of 28 hours and a standard deviation of ?. Given that 5% of the batteries fail, and that only batteries with a life less than 24.052 hours fail, find the value of ?. 3.6 2.4 5 5.76
19 Convert ? ?(50,0.45) into a normal distribution. ?~?(??.?,??.???)
20 Convert ? ?(70,0.53) into a normal distribution. ?~?(??.?,??.???)
21 If ? is a discrete variable, and ? is its continuous equivalent, how would you represent ? ? 7 for ?? ?(? ?.?)
22 If ? is a discrete variable, and ? is its continuous equivalent, how would you represent ? ? 4 for ?? ?(? ?.?)
23 If ? is a discrete variable, and ? is its continuous equivalent, how would you represent ? ? = 5 for ?? ?(?.? < ? < ?.?) OR ?(?.? ? ?.?)
24 If ? is a discrete variable, and ? is its continuous equivalent, how would you represent ? ? < 10 for ?? ?(? ?.?)
25 If ? is a discrete variable, and ? is its continuous equivalent, how would you represent ? ? > 14 for ?? ?(? ??.?)
26 The distances, ? metres, that Michael can hit a golf ball are normally distributed with mean 200 and standard deviation 15. Michael wants to test, at the 5% significance level, whether a new golf club has increased the distance that he can hit a golf ball. Michael uses the new golf club 50 times and records the distances that he hits the ball. State the hypotheses of this hypothesis test. ?0: ? = 200,?1: ? > 200 ?0:? = 200,?1:? 0 ?0: ? = 200,?1: ? 200 ?0:? = 200,?1:? > 0
27 The distances, ? metres, that Michael can hit a golf ball are normally distributed with mean 200 and standard deviation 15. Michael wants to test, at the 5% significance level, whether a new golf club has increased the distance that he can hit a golf ball. Michael uses the new golf club 50 times and records the distances that he hits the ball. What is the test statistic of this test? The total of the 50 distances in the sample The mean of the 50 distances in the sample The difference between the largest distance in the sample vs 200 The difference between the mean of the 50 distances in the sample vs 200
28 The distances, ? metres, that Michael can hit a golf ball are normally distributed with mean 200 and standard deviation 15. Michael wants to test, at the 5% significance level, whether a new golf club has increased the distance that he can hit a golf ball. Michael uses the new golf club 50 times and records the distances that he hits the ball. What is the rejection region of this test? ? > 224.7 ? > 200.5 ? > 203.5 ? > 252.3