Understanding Electronic Structure and Quantum Theory in Atoms
Electronic structure and quantum theory elucidate the behavior and arrangement of electrons in atoms, with a focus on electromagnetic radiation, wavelength, frequency, and the speed of light. The inverse relationship between frequency and wavelength is explored through practical examples involving radiation sources like sodium vapor lamps and laser surgery. Furthermore, the concept of quantization of energy in hot objects, as discovered by Max Planck, sheds light on the emission and absorption of energy in discreet units.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Chapter 6: Electronic Structure quantum theory- explains the behavior of electrons in atoms electronic structure- arrangement of electrons in atoms electromagnetic radiation (EM) (radiant energy)- a form of energy that has wave characteristics and travels at a speed of 3.00 x 108 m/s. ex- gamma rays, X-rays, UV, visible light, infrared, microwaves, radio *Page 209
wavelength- the distance between two adjacent peaks of a wave ( - lambda) frequency- # of complete wave cycles that pass a given point each second ( - nu) *frequency and wavelength are inversely proportional amplitude- distance from origin to peak/trough
Formulas c = c = speed of light (3.00 x 108m/s or 3.00 x 1010cm/s) = frequency (1/s, s-1, Hz) = wavelength (m, cm, nm(10-9)) = c/ = c/
Problem: The yellow light given off by a sodium vapor lamp used for public lighting has a wavelength of 589nm. What is the frequency of this radiation? c= 3.00 x 108m/s = 589nm 5.89 x 10-7m = ? = 3.00 x 108m/s 5.89 x 10-7m = 5.09 x 1014Hz
Practice: 1) A laser used in eye surgery to fuse detached retinas produces radiation with a wavelength of 640.0nm. Calculate the frequency of this radiation. 2) An FM radio station broadcasts electromagnetic radiation at a frequency of 103.4 MHz. Calculate the wavelength of this radiation. 1) 4.69 x 1014Hz 2) 2.90m
Hot Objects and the Quantization of Energy -when solids are heated they emit radiation -wavelength distribution depends on temperature -red-hot objects are cooler than yellowish or white-hot ones Max Planck -found that energy of a body changes in small discrete units quantum- smallest amount of energy that can be emitted or absorbed as EM radiation
E = h E = energy in joules (J) h = Planck s constant = 6.626 x 10 -34J s = frequency (1/s, s-1, Hz) v = E/h -according to Planck, matter can emit and absorb energy only in whole # multiples of h (h , 2 h , 3 h ) ex- 3h = 3 quanta of energy being emitted
Practice: Calculate the energy of one quantum of EM radiation whose frequency is 5 x 10-3Hz. E = (5 x 10-31/s)(6.626 x 10 -34J s) = 3 x 10-36J Can this radiation produce a burst of energy equal to 5 x 10-36J? Why or why not? -No, because it is not a whole # multiple of E
Photoelectric Effect -discovered by Einstein -metals emit electrons when light shines on them *especially the alkali metals -used in solar calculators photons- particles of light *Also uses E = h
Practice: 1) Calculate the energy of one photon of yellow light that has a wavelength of 589nm. 2) A laser emits light that has a frequency of 4.69 x 1014Hz. What is the energy of one photon of this radiation? 3) If the laser emits a pulse containing 5.0 x 1017 photons of this radiation, what is the total energy of the pulse? 4) If the laser emits 1.3 x 10-2J of energy during a pulse, how many photons are emitted?
1) 3.37 x 10-19 J 2) 3.11 x 10-19 J 3) 0.16J 4) 4.2 x 1016 photons
Line Spectra -radiation made up of a single is monochromatic -most radiation contains more than one and is polychromatic spectrum- produced when radiation is separated into component wavelengths continuous spectrum- contains all colors of visible light (ROYGBIV) -not all radiation produces a continuous spectrum line spectrum- contains only radiation of specific wavelengths (page 213 fig 6.11)
Bohrs Model (used H atom) 1) only orbits of certain radii, corresponding to specific energies are permitted for the e- in a H atom 2) an e- in a permitted orbit is in an allowed energy state and does not radiate energy *so it does not spiral into the nucleus 3) energy is emitted or absorbed as a photon by the e- as the e- changes energy levels
ground state- lowest energy state excited state- when an e- moves to a higher energy state *transfer from higher to lower releases energy *transfer from lower to higher absorbs energy
Problems: 1) Using Figure 6.12, predict which of these electronic transitions produces the spectral lines having the longest wavelength: n = 2 to n = 1, n = 3 to n = 2, or n = 4 to n = 3 -energy and wavelength are inversely prop. *n = 4 to n = 3 produces longest wavelength b/c lowest energy
2) Indicate whether each of the following electronic transitions emits energy or absorbs energy. n = 3 to n = 1 emits n = 2 to n = 4 absorbs
-Bohr model began to prove untrue -but did introduce two important ideas *e- exist only in certain discrete energy levels which are described by quantum numbers *energy is involved in the transition of an e- from one level to another
Louis de Broglie -stated that a single electron traveling through space has a wave nature -its wavelength is related to its kinetic energy = h/mv v = h/m = wavelength h = Planck s constant (6.626x10-34J s) m = mass of electron (9.11x10-31kg) v = velocity (m/s) mv = momentum **1J = 1kg m2/s2
Problems: 1) What is the wavelength of an e- moving with a speed of 5.97 x 106m/s? 1.22 x 10-10m 2) Calculate the velocity of a neutron whose de Broglie wavelength is 500pm. The mass of a neutron is 1.67 x 10-27kg. 794m/s
Heisenbergs Uncertainty Principle -states that it is impossible to know both the momentum and position of a particle at the same time
electron configuration- how e- are arranged around the nucleus Rules for e- configs: 1) Aufbau Principle -electrons enter orbitals of lower energy first s p d f -atomic orbitals are represented as boxes s = 1 box d = 5 boxes p = 3 boxes f = 7 boxes
2) Pauli-Exclusion Principle -an atomic orbital can hold at most 2 e- -e- are represented as arrows -spins are opposite -first e- is -second e- is -number of e- must equal number of arrows
3) Hunds Rule -one e- enters each orbital of equal energy (degenerate) until orbitals contain one e-, then they can hold two e- -it is more stable to have partially filled orbitals than empty orbitals
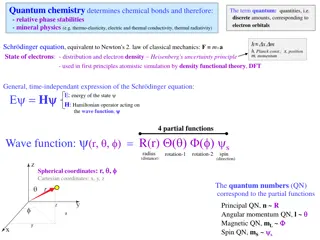
Quantum Mechanics and the Atom Principal Quantum Number (n) -the energy level -determines the overall size and energy of an orbital (where e- are held) -n can be equal to 1, 2, 3, 4, 5, 6, 7 -distance e- is from the nucleus increases as n increases
Angular Momentum Quantum Number (l) -determines the shape of the orbital -shapes are s, p, d, or f -l can be any integer including zero up to n - 1 ex: n = 1 l = 0 ex: n = 2 l = 0, 1
Value of l l = 0 l = 1 l = 2 l = 3 Back to examples: #1: n = 1 l = 0 is in first energy level with s orbitals #2: n = 2 l = 0, 1 is in the second energy level with s and p orbitals Shape of orbital s p d f
Atomic Orbital Shapes s-orbital -spherically shaped -one shape/orbital -lowest energy orbital p-orbital -peanut/dumb bell shaped -three shapes/orbitals d-orbital -clover shaped -five shapes/orbitals
f-orbitals -flower shaped -7 shapes/orbitals energy level n = 1 n = 2 n = 3 n = 4 # of sublevels Type of sublevel 1 2 3 4 1s 2s,2p 3s, 3p, 3d 4s, 4p, 4d, 4f
ex: how many orbitals in 3d? 5 in 4s? 1 in 2p? 3 in 2s? 1 in the third energy level? 9 1 from s, 3 from p, 5 from d
Magnetic Quantum Number (ml) -specifies the orientation of the orbital -equal to integer values, including zero ranging from -l to +l ex: l = 0 ml = 0 ex: l = 1 ml = -1, 0, +1
ex: What are the quantum numbers and names of the orbitals in the n = 4 principal level? How many orbitals exist? n = 4 l ml values 0 0 1 -1, 0, +1 2 -2, -1, 0, +1, +2 3 -3, -2, -1, 0, +1, +2, +3 orbitals 4s 4p 4d 4f **16 total orbitals
Spin Quantum Number (ms) -electron spin is represented by the direction of the arrow -all e- have the same amount of spin -electrons can only spin in one of two directions- up or down ms= +1/2 (spin up) ms= -1/2 (spin down) Question: What are the four quantum numbers for each of the two e- in a 4s orbital? n = 4 l = 0 ml = 0 n = 4 l = 0 ml = 0 ms= +1/2 ms= -1/2