Understanding Dice Patterns in Cubes
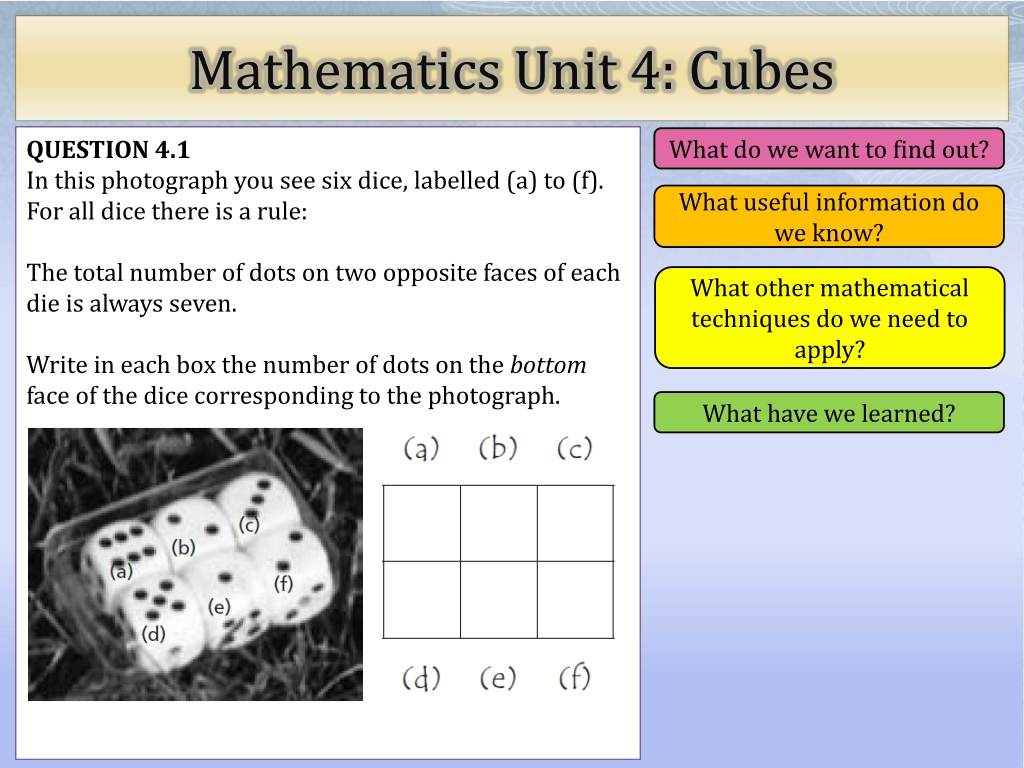
In this unit on cubes, we explore a photograph of six labeled dice (a-f) and a specific rule indicating that the total number of dots on two opposite faces of each die always adds up to seven. By applying mathematical techniques, we aim to determine the number of dots on the bottom face of each die based on the given information. Through this exercise, we enhance our understanding of cube patterns and numerical relationships.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Mathematics Unit 4: Cubes What do we want to find out? What do we want to find out? QUESTION 4.1 In this photograph you see six dice, labelled (a) to (f). For all dice there is a rule: What useful information do we know? What useful information do we know? The total number of dots on two opposite faces of each die is always seven. What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Write in each box the number of dots on the bottom face of the dice corresponding to the photograph. What have we learned? What have we learned?
Mathematics Unit 4: Cubes What do we want to find out? QUESTION 4.1 In this photograph you see six dice, labelled (a) to (f). For all dice there is a rule: What useful information do we know? What useful information do we know? The total number of dots on two opposite faces of each die is always seven. What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Write in each box the number of dots on the bottom face of the dice corresponding to the photograph. What have we learned? What have we learned? Back to start Back to start
Mathematics Unit 4: Cubes What do we want to find out? What do we want to find out? QUESTION 4.1 In this photograph you see six dice, labelled (a) to (f). For all dice there is a rule: What useful information do we know? The total number of dots on two opposite faces of each die is always seven. What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Write in each box the number of dots on the bottom face of the dice corresponding to the photograph. What have we learned? What have we learned? Back to start Back to start
Mathematics Unit 4: Cubes What do we want to find out? What do we want to find out? QUESTION 4.1 In this photograph you see six dice, labelled (a) to (f). For all dice there is a rule: What useful information do we know? What useful information do we know? The total number of dots on two opposite faces of each die is always seven. What other mathematical techniques do we need to apply? Write in each box the number of dots on the bottom face of the dice corresponding to the photograph. What have we learned? What have we learned? Back to start Back to start
Mathematics Unit 4: Cubes What do we want to find out? What do we want to find out? QUESTION 4.1 In this photograph you see six dice, labelled (a) to (f). For all dice there is a rule: What useful information do we know? What useful information do we know? The total number of dots on two opposite faces of each die is always seven. What other mathematical techniques do we need to apply? What other mathematical techniques do we need to apply? Write in each box the number of dots on the bottom face of the dice corresponding to the photograph. What have we learned? Back to start Back to start