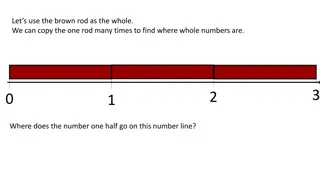
Types of Numbers: Natural, Whole, Integers, Fractions
In this presentation, you'll learn about different sets of numbers including natural numbers, whole numbers, integers, and fractions. Natural numbers are the counting numbers, whole numbers include positive numbers and zero, integers consist of whole numbers and their opposites, and fractions can be proper, improper, or mixed. Each type of number has unique characteristics and uses in mathematics.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Click Slide Show. Then click From Beginning. Sets of Numbers Click the screen when you are ready for the next page. Ms. Andrea Davis Morgan
Natural Numbers Natural Numbers are the counting numbers: 1, 2, 3, 4, 5, . . . Click when ready for the next object.
Natural Numbers Click when ready for the next object.
Whole Numbers Whole Numbers include the counting numbers, Whole Numbers include the counting numbers, The Whole Numbers are positive numbers, except the number zero, which is neither positive nor negative. written with or without a positive sign. written with or without a positive sign. Zero has no sign. The positive numbers can be Zero has no sign. The positive numbers can be 1, 2, 3, 4, 5, . . . 0, 1, 2, 3, 4, 5, . . . 0, 1, 2, 3, 4, 5, . . . 0, 1, 2, 3, 4, 5, . . . 0, 1, 2, 3, 4, 5, . . . or 0, +1, +2, +3, +4, +5, . . . but, also include the number zero. Click when ready for the next object.
Integers Zero, which is neither positive nor negative, Again, zero has no sign, and the positive numbers can be Integers consist of the Whole Numbers. Integers consist of the Whole Numbers Each Integer, except zero, has an opposite which is the same number, but with the opposite sign. is its own opposite. Opposites always have a sum of zero. written with or without a positive sign. -3 + 3 = 0 . . . -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, . . . . . . -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, . . . . . . -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, . . . . . . -5, -4, -3, -2, -1, 0, 1, 2, 3, 4, 5, . . . or 0, 1, 2, 3, 4, 5, . . . -3 + 3 = 0 or -3 + +3 = 0 and the opposites of those Whole Numbers (the negatives). Negative three and positive three are opposites of each other. . . . -5, -4, -3, -2, -1, 0, +1, +2, +3, +4, +5, . . . Click when ready for the next object.
There are three types of numbers involving fractions. Proper fractions Improper fractions Mixed numbers Click when ready for the next object.
In proper fractions, the numerator (top number) is smaller than the denominator (bottom number). 2 7 Click when ready for next object.
In improper fractions, the numerator is larger than the denominator.. 9 7
In mixed numbers, there is a whole number in front of a proper fraction. 2 7 4
There are three types of decimal numbers. Terminating decimal numbers Repeating decimal numbers Non-terminating, Non-repeating decimal numbers Click when ready for the next object.
Terminating decimal numbers have a definite end, a last digit. In the number 5.38, the last digit, the 8, is in the hundredths place. In the number 62.0972, the last digit, the 2, is in the ten thousandths place. Click when ready for next object.
In Repeating decimal numbers, there is no last number because the numbers behind the decimal keep going and going and never stop. There is a number or numbers that repeat in a pattern over and over. In the number 7.88888888 . . . , the number 8 keeps repeating on and on infinitely (forever). The 3 dots indicate that there are more 8 s following the others. Another way to write this number is with a repeating bar over the number that keeps going. 7.88888888 . . . = 7.8 Click when ready for the next object.
In the number 12.6743434343 . . . , the numbers 4 and 3 repeat in a pattern over and over without end. When you write this number with a repeating bar, you only put the bar over the digits that repeat. The bar does not go over the 6 and 7, only the 4 and 3. 12.6743434343 . . . = 12.6743 The repeating bar never goes over the number in front of the decimal. 6.666666666 . . . = 6.6 Click when ready for the next object.
In Non-terminating, Non-repeating decimal numbers, the numbers behind the decimal keep going and never stop, but but there is no pattern to the numbers. Examples: 0.70710678118 . . . 2.64575131106 . . . Click when ready for the next object.
Rational Numbers The Rational Numbers are numbers that can be written as a ratio of two Integers. That means, they can be written as a proper or improper fraction where the numerator and denominator are Integers. -4 5 Example: In this Rational Number, the numerator, -4, is an integer, and the denominator, 5, is an integer. Click when ready for the next object.
Rational Numbers Numbers are Rational Numbers if they can be written in fraction form where a and b are Integers. Since the denominator of a fraction cannot be zero, the denominator b cannot be zero (b 0) -7 2 a b Example: In this Rational Number, the numerator a is -7 and the denominator b is 2. Click when ready for the next object.
Rational Numbers a b Proper and Improper fractions are already in the form and Mixed numbers can be put into that form by converting the Mixed number to an improper fraction. -34 -19 5 Example: = 5 Therefore, all positive and negative fractions and mixed numbers are Rational Numbers. Click when ready for the next object.
Rational Numbers All Natural Numbers, Whole Numbers, and Integers can be written as a ratio of two integers simply by putting the number over one, so all Natural Numbers, Whole Numbers, and Integers are included in the Rational Numbers. -15 -5 3 1 6 2 3 = = = All of these fractions are the ratios of two Integers and are equivalent to the number 3. The number 3 is a Rational Number because it can be written as a ratio of two Integers. Click when ready for the next object.
Rational Numbers a b All Terminating decimal numbers can be written as a fraction . 45 100 9 -69 20 -3 -3 Example: = = = -3.45 (Reduce and change to an improper fraction.) 20 a b All Repeating decimal numbers can be written as a fraction . 271 110 (We will go over how to convert these in a different lesson.) Example: 2.463 = So Terminating decimal numbers and Repeating decimal numbers are Rational numbers. Click when ready for the next object.
Rational Numbers All of the following are Rational Numbers: Proper fractions Improper fractions Mixed numbers Whole numbers Terminating decimals Repeating decimals Click when ready for the next object.
Irrational Numbers That leaves the Non-terminating, Non-repeating decimal numbers. Those are the only decimal numbers that cannot be written in fraction form. Therefore, they are in their own separate set called the Irrational Numbers Example: 2.64575131106 . . . (The prefix ir- means not , so irrational means not rational ). Click when ready for the next object.
Irrational Numbers There are some symbols that we use to represent specific irrational numbers, such as ?, ?, and e. ? = 3.14159265 . . . ? = 1.61803398 . . . e = 2.71828182 . . . Click when ready for the next object.
Irrational Numbers A square root is a number that will multiply times itself to equal the number under the square root sign. For example = 3 because 3 x 3 = 9. 9 is called a perfect square because its square root is a rational number. When you take the square root of a number that is not a perfect square, the result is an irrational number. =1.41421356 . . . Click when ready for the next object.
Real Numbers The Natural Numbers are the counting numbers. Rational Numbers The Natural Numbers are included in the Whole Numbers. The Irrational Numbers are a separate set of numbers. 2 7 8.65 Integers . . . - 7 15 . . . Whole Numbers The Whole Numbers are included in the Integers. Irrational Numbers ? -0.756 -8 3 . . . . . . The Integers are included in the Rational Numbers. 2.645751 . . . Natural Numbers . . . . . . -4, -3, -2, -1, . . . -4, -3, -2, -1 0, 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 0 0 . . . -7.3 4 5 13.2 -2 . . . Click when ready for the next object.
Real Numbers Real Numbers Rational Numbers . . . 2 7 8.65 Integers . . . 2 7 8.65 - 7 15 - 7 15 . . . Whole Numbers -0.756 . . . -8 3 -0.756 . . . Irrational Numbers ? . . . -8 3 . . . . . . . . . Natural Numbers . . . ? . . . -4, -3, -2, -1, . . . -4, -3, -2, -1 0, 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 1, 2, 3, 4, 5, 6 . . . 2.645751 . . . 0 0 . . . 1, 2, 3, 4, 5, 6 . . . . . . 0 . . . -4, -3, -2, -1 2.645751 . . . -7.3 4 5 13.2 -7.3 -2 -24 13.2 . . . . . . . . . 5 Click when ready for the next object.