Topological Phase Transitions Seminar
This seminar on topological phase transitions, presented by Melda Akyazi, covers topics like XY model, spin wave approximation, vortices, experimental realization, and more. It discusses N-Vector models, Ising model, XY model, Heisenberg model, and the characterisation of phase transitions based on energy minimisation and entropy maximisation. The competition between minimising energy and maximising entropy is explored in the context of ordered and disordered states, phase transitions like ferromagnetic to paramagnetic phases, order parameters, and spin-spin correlations. The seminar provides an in-depth understanding of phase transitions in ferromagnetic systems on lattices.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Topological Phase Transitions May 08, 2020 Presented by Melda Akyazi 1 HEIDELBERG UNIVERSITY - SEMINAR ON STATISTICAL PHYSICS - LECTURER: PROF. DR. GEORG WOLSCHIN - SUMMER SEMESTER 2020
Content Prerequisites Motivation XY Model & Spin Wave Approximation Proliferation of Vortices Experimental Realisation Summary & Outlook References 2
N-Vector Models Ising model n = 1 XY model n = 2 Heisenberg model n = 3 system of interacting n-dimensional spins of unit length on a d-dimensional lattice ? in the following we consider only nearest neighbour interactions and for ?,? we denote nearest neighbour pairs by ?,? we are always considering ferromagnetic systems, i.e., the coupling constant J of the spins is positive 4 PREREQUISITES
Characterisation of Phase Transitions Competition between two fundamental concepts: minimisation of energy vs. maximisation of entropy 5 PREREQUISITES
Characterisation of Phase Transitions Competition between two fundamental concepts: minimisation of energy vs. maximisation of entropy ordered state disordered state Phase Transition e.g. ferromagnetic phase paramagnetic phase 6 PREREQUISITES
Characterisation of Phase Transitions Competition between two fundamental concepts: minimisation of energy vs. maximisation of entropy ordered state disordered state Phase Transition e.g. ferromagnetic phase paramagnetic phase order parameter: spin-spin correlation: 7 PREREQUISITES
Characterisation of Phase Transitions Competition between two fundamental concepts: minimisation of energy vs. maximisation of entropy ordered state disordered state Phase Transition e.g. ferromagnetic phase paramagnetic phase order parameter: spin-spin correlation: Long range order (LRO) no LRO break of symmetry 8 PREREQUISITES
Hohenberg-Mermin-Wagner-Theorem Intuitive Approach for d = 1 [1] 10 MOTIVATION
Hohenberg-Mermin-Wagner-Theorem Intuitive Approach for d = 1 [1] Rigorous Results For the n-vector models with continuous symmetries, i.e. for the XY and Heisenberg model, with finite-range exchange interaction there can be no LRO at any nonzero temperature in one and two dimensions. There can be no superconductivity and superfluidity at any nonzero temperature in one and two dimensions. 11 MOTIVATION
Arising Problem Series expansions and numerical results show that there is a phase transition for d = 2 contradiction to the Hohenberg-Mermin-Wagner theorem? 12 MOTIVATION
Arising Problem Series expansions and numerical results show that there is a phase transition for d = 2 contradiction to the Hohenberg-Mermin-Wagner theorem? Hint by Mermin and Wagner: "Our inequality rules out only spontaneous magnetization or sublattice magnetization. It does not exclude the possibility of other kinds of phase transitions." ([J], p. 1135) 13 MOTIVATION
Timeline 1966 1971 1972 Berezinskii's Solution Hohenberg- Mermin- Wagner Theorem Kosterlitz Thouless Theory 14 MOTIVATION
Timeline 3 publications mentioning KT-theory 1977 1966 1971 1972 Berezinskii's Solution Hohenberg- Mermin- Wagner Theorem Kosterlitz Thouless Theory 15 MOTIVATION
Timeline nearly 2200 publications mentioning KT-theory 3 publications mentioning KT-theory 2013 2016 1977 1966 1971 1972 Nobelprize in Physics for Kosterlitz & Thouless (and Haldane) Berezinskii's Solution Hohenberg- Mermin- Wagner Theorem Kosterlitz Thouless Theory 16 MOTIVATION
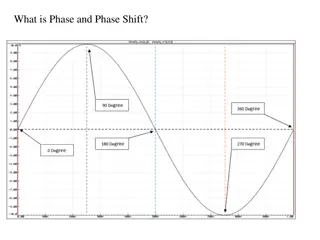
Two Dimensional XY Model [2] The ground state ?0is given by if N denotes the number of nearest neighbours Question: What happens at low tempreatures causing a phase transition? 18 XY MODEL & SPIN WAVE APPROXIMATION
Spin Wave Approximation Assumption: is small, i.e., there are only slowly varying configurations of spins 1st step: 2nd step: extend the range of ??to ( , ) rather than [0,2 ] 19 XY MODEL & SPIN WAVE APPROXIMATION
Spin-Spin Correlations 20 XY MODEL & SPIN WAVE APPROXIMATION
Spin-Spin Correlations 21 XY MODEL & SPIN WAVE APPROXIMATION
Spin-Spin Correlations The decay of correlations is algebraic rather than exponential implying the possibility of a phase transition 22 XY MODEL & SPIN WAVE APPROXIMATION
Characterisation of Phase Transitions ordered state disordered state Phase Transition 23 XY MODEL & SPIN WAVE APPROXIMATION
Characterisation of Phase Transitions ordered state disordered state Phase Transition quasi ordered state New kind of phase transition without symmetry breaking! 24 XY MODEL & SPIN WAVE APPROXIMATION
Vortices Topological charge: with C being a path around a vortex in anti-clockwise direction, M mapping an angle ? to ?,? and ?,? being nearest neighbours. [3] vortex anti-vortex 26 PROLIFERATION OF VORTICES
Vortices Topological charge: with C being a path around a vortex in anti-clockwise direction, M mapping an angle ? to ?,? and ?,? being nearest neighbours. [3] Continuum limit: vortex anti-vortex for r being a radial coordinate 27 PROLIFERATION OF VORTICES
Energy Calculation for Single Vortices Question: Is it favourable to make vortex excitations? Calculate energy of a single vortex: 28 PROLIFERATION OF VORTICES
Energy Calculation for Single Vortices Question: Is it favourable to make vortex excitations? Calculate energy of a single vortex: The configurational entropy is given by , hence as an upper bound 29 PROLIFERATION OF VORTICES
Energy Calculation for Single Vortices Question: Is it favourable to make vortex excitations? Calculate energy of a single vortex: whereas for pairs of vortices and antivortices at positions ??and ??: The configurational entropy is given by , hence as an upper bound 30 PROLIFERATION OF VORTICES
Vortex-Antivortex Pairs [5] 31 PROLIFERATION OF VORTICES
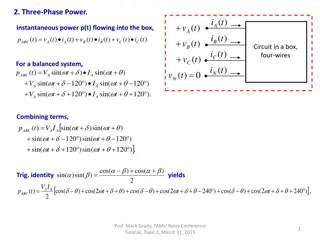
Experimental Realisation ?????where ??is the superfluid density Superfluids can be expressed as ? = 33 EXPERIMENTAL REALISATION
Experimental Realisation ?????where ??is the superfluid density Superfluids can be expressed as ? = by Z. Hadzibabic, P. Kr ger, M. Cheneau, B. Battelier, J. Dalibard [6] [7] 34 EXPERIMENTAL REALISATION
Something to think about "My almost total ignorance of the conventional wisdom of condensed matter physics seemed to be a real advantage in this situation where genuinely new physics was involved." "Almost always the men who achieve these fundamental inventions of a new paradigm have been either young or very new to the field whose paradigm they change. And perhaps that point need not have been made explicit, for obviously these are the men who, being little committed by prior practice to the traditional rules of normal science, are particularly likely to see that those rules no longer define a playable game and to conceive another set that can replace them." "As I was operating from a position of extreme ignorance, these [concepts like topological defects] did not seem any stranger to me than other ideas about phase transitions." - Thomas Kuhn The Structure of Scientific Revolutions. 50th Anniversary Edition. The University of Chicago Press 2012, p.90 -John Michael Kosterlitz [E] p.1, [F] p.2 36 SUMMARY & OUTLOOK
Something to think about "My almost total ignorance of the conventional wisdom of condensed matter physics seemed to be a real advantage in this situation where genuinely new physics was involved." "Almost always the men who achieve these fundamental inventions of a new paradigm have been either young or very new to the field whose paradigm they change. And perhaps that point need not have been made explicit, for obviously these are the men who, being little committed by prior practice to the traditional rules of normal science, are particularly likely to see that those rules no longer define a playable game and to conceive another set that can replace them." "As I was operating from a position of extreme ignorance, these [concepts like topological defects] did not seem any stranger to me than other ideas about phase transitions." - Thomas Kuhn The Structure of Scientific Revolutions. 50th Anniversary Edition. The University of Chicago Press 2012, p.90 -John Michael Kosterlitz [E] p.1, [F] p.2 Thank you for your attention! 37 SUMMARY & OUTLOOK
References for Images [1] & [2] Kadanoff, L. P. (2000). Statistical physics: statics, dynamics and renormalization. World Scientific Publishing Company. p. 380 & p.378 [3] Kardar, M. (2007). Statistical physics of fields. Cambridge University Press. p. 165 [4] Simon Willerton. URL: https://johncarlosbaez.wordpress.com/2016/10/07/kosterlitz-thouless-transition/ (access on 2nd May 2020) [5] Brian Skinner. URL: https://johncarlosbaez.wordpress.com/2016/10/07/kosterlitz-thouless-transition/ (access on 2nd May 2020) [6] & [7] Hadzibabic, Z., Kr ger, P., Cheneau, M., Battelier, B., & Dalibard, J. (2006). Berezinskii-Kosterlitz-Thouless crossover in a trapped atomic gas. Nature, 441(7097), 1118 1121. p. 1 & p.4 38 REFERENCES
References [A] Chaikin, P. M., Lubensky, T. C., Witten, T. A. (1995). Principles of condensed matter physics (Vol. 10). Cambridge University Press. [B] Hadzibabic, Z., Kr ger, P., Cheneau, M., Battelier, B., Dalibard, J. (2006). Berezinskii-Kosterlitz-Thouless crossover in a trapped atomic gas. Nature, 441(7097), 1118 1121. [C] Kadanoff, L. P. (2000). Statistical physics: statics, dynamics and renormalization. World Scientific Publishing Company. [D] Kardar, M. (2007). Statistical physics of fields. Cambridge University Press. [E] Kosterlitz, J. M. (2016). Commentary on Ordering, metastability and phase transitions in two-dimensional systems J M Kosterlitz and D J Thouless (1973 J. Phys. C: Solid State Phys. 6 1181-203) - The early basis of the successful Kosterlitz-Thouless theory. Journal of Physics Condensed Matter, 28(48), 481001. [F] Kosterlitz, J. M. (2016). Kosterlitz-Thouless physics: A review of key issues. Reports on Progress in Physics, 79(2), 26001. [G] Kosterlitz, J. M., Thouless, D. J. (1973). Ordering, metastability and phase transitions in two-dimensional systems. Journal of Physics C: Solid State Physics, 6(7), 1181 1203. 39 REFERENCES
References [H] Kosterlitz, J. M., Thouless, D. J. (2016). Early Work on Defect Driven Phase Transitions. International Journal of Modern Physics B, 30(30), 1 67. [I] Kosterlitz, J. M. (1974). The critical properties of the two-dimensional xy model. Journal of Physics C: Solid State Physics, 7(6), 1046 1060. [J] Mermin, N. D., Wagner, H. (1966). Absence of ferromagnetism or antiferromagnetism in one- or two-dimensional isotropic Heisenberg models. Physical Review Letters, 17(22), 1133 1136. [K] Plischke, M., Bergersen, B. (1994). Equilibrium statistical physics. World Scientific Publishing Company. 40 REFERENCES

 undefined
undefined