Projectile Motion
Learn about projectile motion, trajectory, and velocity vectors in this detailed guide by Kelly Rick. Explore how to solve problems involving projectile motion, understand the horizontal and vertical components, and analyze motion separately in the x and y directions. Discover the key steps to successfully approach and solve projectile motion problems using diagrams and coordinate systems. Master the principles behind horizontally launched objects and angles of departure to enhance your understanding of this essential physics concept.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
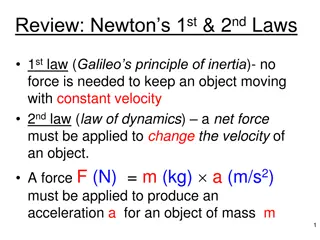
Projectile Motion Unit 6
Created by Kelly Rick Projectile Motion
There are only two kinds of problems and we will approach each separately then mix for the review: Horizontally launched Departure angle of 0 Launched at an angle 3 Created by Kelly Rick
Projectile Motion and the Velocity Vector Any object that is moving through the air affected only by gravity is called a projectile. The path a projectile follows is called its trajectory. 4 Created by Kelly Rick
Projectile Motion and the Velocity Vector The trajectory of a thrown basketball follows a special type of arch-shaped curve called a parabola. The distance a projectile travels horizontally is called its range. Range 5 Created by Kelly Rick
Projectile Motion It can be understood by analyzing the horizontal (x) and vertical (y) motions separately. 6 Created by Kelly Rick
Projectile Motion The speed in the x- direction is constant; in the y-direction the object moves with constant acceleration g. This photograph shows two balls that start to fall at the same time. The one on the right has an initial speed in the x-direction. It can be seen that vertical positions of the two balls are identical at identical times, while the horizontal position of the yellow ball increases linearly. 7 Created by Kelly Rick
Solving Problems Involving Projectile Motion 1. Read the problem carefully, and choose the object(s) you are going to analyze. 2. Draw a diagram. 3. Choose an origin and a coordinate system. 4. Decide on the time interval; this is the same in both directions, and includes only the time the object is moving with constant acceleration g. 5. Examine the x and y motions separately. 8 Created by Kelly Rick
Solving Problems Involving Projectile Motion 6. List known and unknown quantities. Remember that vx never changes, and that vy = 0 at the highest point. 7. Plan how you will proceed. Use the appropriate equations; you may have to combine some of them. 9 Created by Kelly Rick
The famous T chart quantity Horizontal X motion Vertical Y motion distance acceleration Initial Velocity Final Velocity time 0 m/s2 -10 m/s2 0 m/s Same Same 10 Created by Kelly Rick
Horizontally Launched Projectiles 11 Created by Kelly Rick
Horizontal Speed The ball s horizontal velocity remains constant while it falls because gravity does not exert any horizontal force. Since there is no force, the horizontal acceleration is zero (ax = 0). The ball will keep moving to the right at 5 m/sec. 12 Created by Kelly Rick
Horizontal Speed & distance The horizontal distance a projectile moves can be calculated according to the formula: 13 Created by Kelly Rick
Vertical Speed The vertical speed (vy) of the ball will increase by 9.8 m/sec after each second. After one second has passed, vyof the ball will be 9.8 m/sec. After the 2nd second has passed, vywill be 19.6 m/sec and so on. 14 Created by Kelly Rick
Solving Problems Involving Projectile Motion Example : Driving off a cliff. A movie stunt driver on a motorcycle speeds horizontally off a 50.0-m-high cliff. How fast must the motorcycle leave the cliff top to land on level ground below, 90.0 m from the base of the cliff where the cameras are? Ignore air resistance. 15 Created by Kelly Rick
Created by Kelly Rick Vector Review
Vectors and Direction Key Question: How do we accurately communicate length and distance? 17 Created by Kelly Rick
Vectors and Direction A scalar is a quantity that can be completely described by one value: the magnitude. You can think of magnitude as size or amount, including units. 18 Created by Kelly Rick
Vectors and Direction A vector is a quantity that includes both magnitude and direction. Vectors require more than one number. The information 1 kilometer, 40 degrees east of north is an example of a vector. 19 Created by Kelly Rick
20 Created by Kelly Rick
21 Created by Kelly Rick
Calculate a resultant vector An ant walks 2 meters West, 3 meters North, and 6 meters East. What is the displacement of the ant? 22 Created by Kelly Rick
Finding Vector Components Graphically Draw a displacement vector as an arrow of appropriate length at the specified angle. Mark the angle and use a ruler to draw the arrow. 23 Created by Kelly Rick
24 Created by Kelly Rick
Finding the Magnitude of a Vector When you know the x- and y- components of a vector, and the vectors form a right triangle, you can find the magnitude using the Pythagorean theorem. 25 Created by Kelly Rick
Projectiles Launched at an Created by Kelly Rick Angle 26
Projectile Motion If an object is launched at an initial angle of 0 with the horizontal, the analysis is similar except that the initial velocity has a vertical component. Vx=Constant velocity ax=0 Horizontal: velocity is constant and acceleration is zero 27 Created by Kelly Rick
Projectile Motion If an object is launched at an initial angle of 0 with the horizontal, the analysis is similar except that the initial velocity has a vertical component. Vy=changing velocity ay=-10 Vyi not 0m/s Vertical: acceleration is -10m/s/s 28 Created by Kelly Rick
Components and vectors Represents the velocity of an object in 2D space 29 Created by Kelly Rick
Components and vectors Now we resolve the vector into its x and y components why? Because the combination of the action in the x and y combined produce the 2D motion represented below 30 Created by Kelly Rick
Components and vectors Y-Component of motion x-Component of motion 31 Created by Kelly Rick
Components of the motion Y-Component of motion x-Component of motion 32 Created by Kelly Rick
Practicing component form Draw the x and y components for the following vector 37 33 Created by Kelly Rick
Practicing component form Draw the x and y components for the following vector Y-component of 18m/s 37 x-component of 18m/s 34 Created by Kelly Rick
Practicing component form Draw the x and y components for the following vector Y-component of 18 SOH CAH TOA Sine 37=Y-COMPONENT 18 Y- component= 18 (sin 37) Y-component =10.8 Y-component of 18 37 x-component of 18m/s COS 37=X-COMPONENT 18 X- component= 18 (cos 37) X-component =14.4 35 Created by Kelly Rick
Practicing component form2 Draw the and R components for the following vector 8m SOH CAH TOA 6m 36 Created by Kelly Rick
Practicing component form2 Draw the and R components for the following vector 8m R= x2+y2=10m SOH CAH TOA 6m Tan =opp/adj Tan = 8m/6m =Tan-1 (8/6) =53 37 Created by Kelly Rick
Screen Shot 2013-02-02 at 11.37.08 PM.png Note Taking Guide 1 Note Taking Guide 2 Using Math to Find Resultants Using Math to Find Components Vector Math Practice Sheet Review Review 38 Created by Kelly Rick
3-8 Solving Problems Involving Projectile Motion Example 3-7: A kicked football. A football is kicked at an angle 0 = 37.0 velocity of 20.0 m/s, as shown. Calculate (a) the maximum height, (b) the time of travel before the football hits the ground, (c) how far away it hits the ground, (d) the velocity vector at the maximum height, and (e) the acceleration vector at maximum height. Assume the ball leaves the foot at ground level, and ignore air resistance and rotation of the ball. with a 39 Created by Kelly Rick
Solving Problems Involving Projectile Motion Conceptual Example : Where does the apple land? A child sits upright in a wagon which is moving to the right at constant speed as shown. The child extends her hand and throws an apple straight upward (from her own point of view), while the wagon continues to travel forward at constant speed. If air resistance is neglected, will the apple land (a) behind the wagon, (b) in the wagon, or (c) in front of the wagon? 40 Created by Kelly Rick
41 Created by Kelly Rick
Solving Problems Involving Projectile Motion Conceptual Example : The wrong strategy. A boy on a small hill aims his water-balloon slingshot horizontally, straight at a second boy hanging from a tree branch a distance d away. At the instant the water balloon is released, the second boy lets go and falls from the tree, hoping to avoid being hit. Show that he made the wrong move. (He hadn t studied physics yet.) Ignore air resistance. 42 Created by Kelly Rick
Note Taking Guide I Note Taking Guide II Projectile Problems Review 43 Created by Kelly Rick