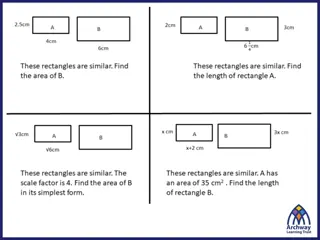
Problem-Solving Scenarios in Work and Distance Calculations
In these scenarios, you are faced with various work and distance calculation problems involving bricklaying, surveying, and boat speed determination. The solutions are presented with detailed equations and charts to help you understand the concepts and apply them effectively. These practical examples enhance your problem-solving skills in real-life situations.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
Work problems #22: An experienced bricklayer constructs a small wall in 3 hours. The apprentice (you) completes the job in 6 hours. Find how long it takes if they work together. The answer is not 4.5 hours (no averaging!) The answer is not 9 hours (Makes no sense!!)
An experienced bricklayer constructs a small wall in 3 hours. The apprentice (you) completes the job in 6 hours. Find how long it takes if they work together. Make a chart! Hours to complete total job Part of job completed in 1 hr Experienced worker 3 1/3 You 6 1/6 Team effort x 1/x Equation set-up: 1/3 + 1/6 = 1/x Solution: x = 2 hrs
#21 Section 5.6 Smith Engineering found that an experienced surveyor surveys a roadbed in 4 hours. An apprentice surveyor needs 5 hours to do the job. If the two work together, find how long it takes them to complete the job? How long to finish the job Portion of the job completed in 1 hr. Co-worker 4 hrs Apprentice 5 hrs 1/5 Team or working together X 1/x Set-up: 1/4 + 1/5 = 1/x LCD = 20x Solution x = 20/9 hours
#26: Distance = Rate X Time A boat can travel 9 miles upstream in the same amount of time it takes to travel 11 miles downstream. If the current of the river is 3 mi/hr, complete the chart below and use it to find the speed of the boat in still water.
A boat can travel 9 miles upstream in the same amount of time it takes to travel 11 miles downstream. If the current of the river is 3 mi/hr, complete the chart below and use it to find the speed of the boat in still water (let boat speed = x). Dist = rate x time time = Dist/rate Distance Rate (boat & river) Time Upstream (against river current slows you down) 9 mi X - 3 (Same time) Downstream (with river current speeds you up) 11 mi X + 3 (Same time) Set-up: 9 x 11 x + = The speed of the boat in still water is 30 mi/hr 9(x+3) = 11 (x-3) 9x+27 = 11x-33 27+33 = 11x 9x 60 = 2x 30 = x 3 3
