Power Analysis and Efficiency Calculations for Three-Phase Induction Motors
The provided content discusses the power relations and efficiency calculations for two examples of three-phase induction motors. It covers topics such as air gap power, power converted, output power, stator copper losses, rotor copper losses, core losses, stray losses, and efficiency calculations. Detailed solutions and relevant formulas are provided for a better understanding.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
THREE-PHASE INDUCTION MOTORS An Approximate Equivalent Circuit
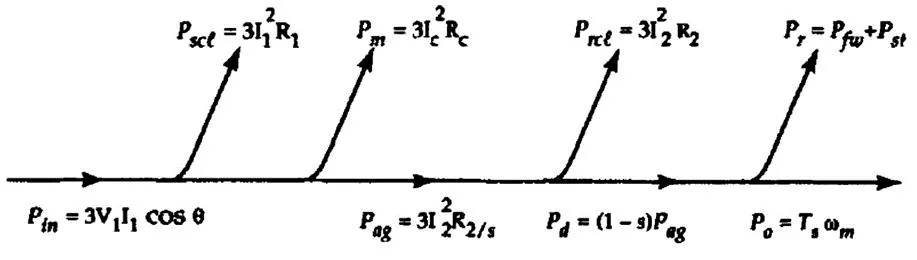
Power Relations For 3-Phase IM EXAMPLE 1-2 A 480V, 60Hz, 50hp, Y-connected, 3 phase induction motor is drawing 60A at 0.85 PF lagging. The stator copper losses are 2kW, and the rotor copper losses are 700W. The friction and windage losses are 600W, the core losses are 1800W, and the stray losses are negligible. Using the power flow diagram in Figure 1-6a, find: a) The air gap power Pag. b) The power converted Pd. c) The output power Po. d) The efficiency of the motor. SOLUTION a) The phase voltage V1 = VL/ 3 = 480/ 3 = 277V Pin Pag Pscl = Pin Pm=42400 2000 1800=38600W = 3V1I1cos = 3 277 60 0.85 = 42400W or 42.4kW or 38.6kW Pd = Pag Prcl = 38600 700 = 37900W P0 = Pd Pr= Pd Pfw Pst= 37900 600 0 =37300W % =Po 100 =37300 100 =88% or 37.9kW b) c) or 37.3kW d) 42400 Pin 2
Power Relations For 3-Phase IM EXAMPLE 1-3 A 6-pole, 230-V, 60-Hz, Y-connected, three-phase induction motor has the following parameters on a per-phase basis: R1 = 0.5 , R2 = 0.25 , X1 = 0.75 , X2 = 0.5 , Xm = 100 , and Rc = 500 . The friction and windage loss is 150 W. Determine the efficiency of the motor at its rated slip of 2.5%. SOLUTION The synchronous speed of the motor is N =120 f=120 60 = 1200 rpm or s 6 P s= 125.66 rad / s The per-phase applied voltage is V =VL=230 = 132.8V 1 3 3 The effective rotor impedance as referred to the stator is =0.25 0.025 =R2 + jX + j 0.5 = 10 + j 0.5 Z 2 2 s 3
Power Relations For 3-Phase IM SOLUTION (1-3) cont. Since Rc, jXm, and are in parallel, we can compute the equivalent impedance as: 1=1 Z 2 1 1 + + Z e Rc 1 500 j Xm 1 1 Z e= 9.619 + j 1.417 and = + + = 0.102 j 0.015 S 10 + j0.5 j100 The stator winding impedance is Z = R + jX = 0.5 + j 0.75 1 1 1 Hence, the total input impedance is Z = Z + Z = 10.119 + j 2.167 1 in e The stator current: 132.8 00 V Zin I1 = 1=10.119 + j2.167 =12.832 12.1 A 0 The power factor: pf = cos( ) = cos( ) = cos(12.1 ) = 0.978 lagging 0 v i Power input: Pin= 3V1 I1 cos = 3 132.8 12.832 0.978 = 4998.54 W 4
Power Relations For 3-Phase IM SOLUTION (1-3) cont. Stator copper loss: P = 3I 2 R = 3 12.8322 0.5 =246.99 W scl 1 1 The induced emf in the stator winding is E = V I Z =132.8 (12.832 12.10)(0.5+ j0.75)=124.763 3.710V 1 1 1 1 Hence, the Core loss is Pm = 3E2/ R = 3(124.76)2 / 500 = 93.75W The Air-gappower: Pag Pscl 1 c = Pin Pm = 4657.8 W The Rotor copper loss: Prcl= 3 I2R P = sP rcl = 0.25 4657.8 = 116.46W or 2 2 ag Power developed: Prcl Pd = Pag = 4541.34 W Power output: P0 = Pd Pr= 4541.34 150 = 4391.34 W Efficiency: % =P0 100 =87.9% Pin 5
An Approximate Equivalent Circuit A well-designed three-phase induction motor usually meets most ofthe following guidelines: (refer to the per-phase equivalent circuit shown in Figure 1-4) 1.The stator winding resistance is kept small in order to reduce thestator copper loss. 2.The stator winding leakage reactance is minimized by reducing the mean- turn length of each coil. 3.Thin laminations of low-loss steel are used to cut down the core loss.Thus, the equivalent core-loss resistance is usually high. 4.The permeability of steel selected for laminations is high, and the operating flux density in the motor is kept below the knee of the magnetization curve. Thus, the magnetization reactance is usually high. Figure1-4 21
An Approximate Equivalent Circuit An induction motor conforming to the above stipulations can be represented by an approximate equivalent circuit, as shown in Figure 1-7, where wehave placed the parallel branch (the excitation circuit) across the powersource. We admit that the analysis of an induction motor using the approximate equivalent circuit is somewhat inaccurate, but the inaccuracy is negligible for a well-designed motor. On the other hand, the approximate equivalent circuit not only simplifies the analysis but also aids in comprehending various characteristics of the motor. For instance, we use the approximate equivalent circuit to determinethe speed at which (a) the torque developed is maximum, (b) the power developed is maximum, and (c) the motor efficiency ismaximum. Figure 1-7 An approximate equivalent circuit on a per-phase basis of a balanced three-phase induction motor. 7
An Approximate Equivalent Circuit EXAMPLE 1-4 A 6-pole, 230-V, 60-Hz, Y-connected, three-phase induction motor has the following parameters on a per-phase basis: R1 = 0.5 , R2 = 0.25 , X1 = 0.75 , X2 = 0.5 , Xm = 100 , and Rc = 500 . The friction and windage loss is 150 W. Determine the efficiency of the motor at its rated slip of 2.5%. Using the approximate equivalent circuit shown in Figure 1-6 SOLUTION The synchronous speed of the motor is N =120 f=120 60 = 1200 rpm or s 6 P s= 125.66 rad / s The per-phase applied voltage is V =VL=230 = 132.8V Figure1-7 1 3 3 The effective rotor impedance as referred to the stator is ) s R + jX =(0.5 + j0.75) + 0.25 + j0.5 = 10.5 + j1.25 Z = (R + jX + 2 2 0.025 1 1 e 8
An Approximate Equivalent Circuit SOLUTION (1-4) cont. Hence, the rotor current: 132.8 =V1 = =12.558 6.790A I 2 10.5 +j1.25 Z e Core-loss current: Figure1-7 =V1 =132.8=0.266 A 500 I c R Magnetization current is c V1 jX =132.8 = j1.328 A j100 = I m The stator current: m I1 =I2 + Ic + Im =12.558 6.79 +0.266 j1.328 =13.043 12.45 0 A 0 The power factor: pf = cos( ) = cos( ) = cos(12.45 ) = 0.977lagging 0 v i Power input: Pin= 3V1 I1 cos = 3 132.8 13.043 0.977 = 5074 W Compare to 4998.57W 9
An Approximate Equivalent Circuit SOLUTION (1-4) cont. Stator copper loss: P = 3I2R = 3 12.5582 0.5= 236.6 W scl 2 1 Hence, the Core loss is P = 3I2R = 3 0.2662 500 = 106.1 W Figure1-7 m c c The Air-gappower: = Pin Pm = 4731.3 W Pscl Pag Compare to 4657.8W The Rotor copper loss: = 3I2R = 3 12.5582 0.25 =118.3 W P rcl 2 2 Power developed: Prcl=4731.3 118.3 =4613 W Pd = Pag Power output: P0 = Pd Pr= 4613 150 = 4463 W Efficiency: % =P0 100 =4463 100 =87.96% Compare to 87.9% 5074 Pin 10
An Approximate Equivalent Circuit EXAMPLE 1-5 A 460V, 25hp, 60Hz, 4 pole, Y-connected induction motor has the following impedances in ohms per phase referred to the stator circuit: R1 = 0.641 , R2 = 0.332 , X1 = 1.106 , X2 = 0.464 , and Xm = 26.3 . The total rotational losses are 1100W and are assumed to be constant. The core loss is lumped in with the rotational losses. For a rotor slip of 2.2% at the rated voltage and rated frequency, using the approximate equivalent circuit in Figure 1-8, find the motor s a) stator current, b) power factor, c) Pd and Po, d) the efficiency, and e) Td andTo. SOLUTION The synchronous speed of the motor is N =120 f=120 60 = 1800 rpm or P 4 s= 188.5 rad / s s a) The per-phase applied voltage is V =VL=460 = 265.6V Figure 1-8 A modified approximate equivalent circuit. 1 3 3 The effective rotor impedance as referred to the stator is ) s R + jX =(0.641 + j1.106) + 0.332 + j0.464 = 15.732 + j1.57 Z = (R + jX + 2 2 0.022 1 1 e 11
An Approximate Equivalent Circuit SOLUTION (1-5) cont. Hence, the rotor current: 265.6 =V1 =16.8 5.70A = I 2 15.732 + j1.57 Z e Magnetization current is Figure1-8 V1 jX =265.6 = j10.1=10.1 900A j26.3 = I m m The stator current: Im =16.8 5.7 j10.1 = 20.44 35.14 0 A I1 = I2+ 0 b) The power factor: pf = cos( ) = cos( ) = cos(35.140 ) = 0.8177 lagging 1 v1 i1 c) Power input: Pin= 3V1I1cos 1= 3 265.6 20.44 cos(35.14) =13318 W or Pin= 3V1I2cos( v1 i2)= 3V1I2cos 2= 3 265.6 16.8 cos(5.7)=13320 W 12
An Approximate Equivalent Circuit SOLUTION (1-5) cont. The Air-gappower: P = 3 I 2 R2 = 3 16.82 0.332 = 12777.8 W s 2 ag 0.022 Power developed: Pd= (1 s)Pag=(1 0.022) 12777.8 =12496.7 W d) Power output: P0 = Pd Pr= 12496.7 1100 = 11396.7 W Figure1-6(b) Efficiency: % =P0 100 =11396.7 100 = 85.57% 13318 Pin e) Developed torque: m =(1 s) s = (1 .022) 188.5=184.35 rad / s Pag s 12777.8 =Pd =12496.7 = 67.8 N.m m 184.35 T = d = = 67.8 N.m T or d 188.50 Shaft (load) torque: T =Po=11396.7 = 61.8 N.m o m 184.35 13
Three-Phase Induction Motors Problems Power Relations: 7. A 10-hp, 4-pole, 440-V, 60-Hz, Y-connected, three-phase induction motor runs at 1725 rpm on full load. The stator copper loss is 212 W, and the rotational loss is 340 W. Determine (a) the power developed, (b) the airgap power, (c) the rotor copper loss, (d) the total power input, and (e) the efficiency of the motor. What is the shaft torque? 8. A 2-hp, 120-V, 60-Hz, 4-pole, Y-connected, three-phase induction motor operates at 1650 rpm on full load. The rotor impedance at standstill is 0.02 + j0.06 /phase. Determine the rotor current if the rotational loss is 160 W. What is the magnitude of the induced emf in the rotor? 9. A three-phase induction motor operates at a slip of 3% and has a rotor copper loss of 300 W. The rotational loss is 1500 W. Determine (a) the air gap power and (b) the power output. If the rotor impedance is 0.2 + j0.8 /phase, what is the magnitude of the induced emf per phase in the rotor? 10.A 10-hp, 6-pole, 440-V, 60-Hz, -connected, three-phase induction motor is designed to operate at 3% slip on full load. The rotational loss is 4% of the power output. When the motor operates at full load, determine (a) the rotor copper loss, (b) the air-gap power, (c) the power developed, and (d) the shaft torque. 14
Three-Phase Induction Motors Problems Development of an Equivalent Circuit: 11.A 208-V, 50-Hz, 12-pole, Y-connected, three-phase induction motor has a stator impedance of 0.1 + j0.3 /phase and a rotor impedance of 0.06 + j0.8 /phase at standstill. The core-loss resistance is 150 /phase, and the magnetization reactance is 750 /phase. The friction and windage loss is 2 kW. When the motor operates at its full-load slip of 5%, determine (a) the power input, (b) the stator copper loss, (c) the rotor copper loss, (d) the air-gap power, (e) the power developed, (f) the power output, (g) the efficiency, (h) the shaft torque, and (i) the horsepower rating of the motor. 12.The per-phase equivalent circuit parameters of a 208-V, 4-pole, 50-Hz, three-phase, Y-connected, induction motor are R1 = 0.4 , X1 = 0.8 , R2 = 0.3 , X2 = 0.9 , and Xm = 40 . The core loss is 45 W, and the friction and windage loss is 160 W. When the motor operates at a slip of 5%, determine (a) the input current, (b) the power input, (c) the air-gap power, (d) the power developed, (e) the power output, ( f ) the shaft torque, and (9) the efficiency of the motor. Draw its power-flow diagram. 13.Calculate the starting torque developed by the motor of Problem 12. 15
Three-Phase Induction Motors Problems A. A 4-pole, 230-V, 60-Hz, Y-connected, three-phase induction motor has the following parameters on a per-phase basis: R1 = 10.12 , X1 = 38.61 , R2 = 21.97 , X2= 11.56 , and Xm = 432.48 . The core loss is 10.72 W, and the friction and windage loss is 5.9 W. When the motor operates at its rated speed of 1550 rpm, determine (a)the stator current, (b) the magnetization current, (c) the rotor current, (d) the power input, (e) the stator copper loss, ( f ) the rotor copper loss, (g) the power output, (h) the shaft torque, and (i) the efficiency. An Approximate Equivalent Circuit: 15.Redo Problem 12 using the approximate equivalent circuit. Also, solve Problem 13. 16.The equivalent circuit parameters of a 208-V, 60-Hz, 6-pole, Y-connected, three- phase induction motor in ohms/phase are R1 = 0.21, R2 = 0.33, X1 = 0.6, X2 = 0.6, Rc = 210, and Xm = 450. When the motor runs at a slip of 5% on full load, determine the torque developed by the motor using the approximate equivalent circuit. What is the starting torque developed by the motor? 16