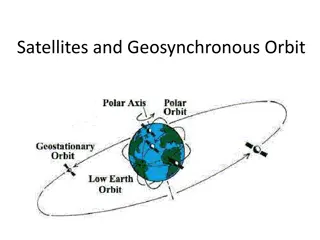
Planet Nine: Orbits, Mass, and Kepler's Law
Some helpful numbers and formulae
Group 1
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 2
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 3
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 4
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 5
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 6
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 1
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 2
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 3
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 4
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 5
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Group 6
T
h
e
r
e
i
s
s
o
m
e
e
v
i
d
e
n
c
e
f
r
o
m
d
i
s
t
a
n
t
S
o
l
a
r
S
y
s
t
e
m
o
b
j
e
c
t
s
o
f
a
l
a
r
g
e
m
a
s
s
i
v
e
b
o
d
y
v
e
r
y
f
a
r
f
r
o
m
t
h
e
S
u
n
,
o
f
t
e
n
r
e
f
e
r
r
e
d
t
o
a
s
“
P
l
a
n
e
t
N
i
n
e
.
”
A
s
t
r
o
n
o
m
e
r
s
p
r
e
d
i
c
t
t
h
a
t
,
i
f
t
h
i
s
b
o
d
y
w
e
r
e
t
o
e
x
i
s
t
,
P
l
a
n
e
t
N
i
n
e
w
o
u
l
d
b
e
o
n
a
m
o
d
e
r
a
t
e
l
y
e
l
l
i
p
t
i
c
a
l
o
r
b
i
t
;
a
d
d
i
t
i
o
n
a
l
l
y
,
i
t
w
o
u
l
d
b
e
4
t
i
m
e
s
m
o
r
e
m
a
s
s
i
v
e
t
h
a
n
t
h
e
E
a
r
t
h
.
A
s
t
r
o
n
o
m
e
r
s
a
l
s
o
p
r
e
d
i
c
t
a
s
e
m
i
-
m
a
j
o
r
a
x
i
s
o
f
6
0
0
A
U
a
n
d
a
s
e
m
i
-
m
i
n
o
r
a
x
i
s
o
f
5
0
0
A
U
.
(a)
What would the period of Planet Nine’s orbit be?
(b)
Let’s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the
orbit of Planet Nine?
(c)
Now imagine that Planet Nine were 4 times more massive
than the Sun
. Using Newton’s version of Kepler’s
Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Pluto and its moon,
Charon, taken by New
Horizons in 2015
Astronomers speculate about Planet Nine, a massive body in the distant Solar System. Calculations are made about its orbit period, potential interactions with a black hole, and changes in period if it were more massive than the Sun. Utilizing Newton's version of Kepler's Law, astronomers delve into the dynamics of this hypothetical celestial body.
Download Presentation
Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Group 1 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Group 2 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Group 3 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Group 4 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Group 5 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
Group 6 Pluto and its moon, Charon, taken by New Horizons in 2015 There is some evidence from distant Solar System objects of a large massive body very far from the Sun, often referred to as Planet Nine. Astronomers predict that, if this body were to exist, Planet Nine would be on a moderately elliptical orbit; additionally, it would be 4 times more massive than the Earth. Astronomers also predict a semi-major axis of 600 AU and a semi-minor axis of 500 AU. (a) What would the period of Planet Nine s orbit be? (b) Let s imagine replacing the Sun with a black hole of the same mass as the sun. How does this change the orbit of Planet Nine? (c) Now imagine that Planet Nine were 4 times more massive than the Sun. Using Newton s version of Kepler s Law, calculate the new period of Planet Nine assuming the semi-major and semi-minor axes.
