Physics 2053 Final Review - Concepts and Practice Problems
Covering topics such as vectors, kinematics, forces, Newton's laws, circular motion, work and energy, impulse, momentum, rotational dynamics, harmonic motion, and more. Practice problems included to test understanding and application of these concepts.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
OVERVIEW oVectors oKinematics oForces and Newton s Laws oCircular Motion oWork and Energy oImpulse oMomentum oRotational Kinematics oRotational Dynamics (torque) oHarmonic Motion oQuestions and further clarifications
VECTORS Vector a quantity that has both magnitude and direction Scalar has no direction -speed -velocity -mass -displacement -distance -acceleration -force
KEEP IN MIND oTrig functions oSin, cos, tan ???????? ????????? ???????? ????????? ???????? ???????? osin? = ocos? = otan? = o Addition is tip-to-tail oWhen in doubt, use components oAll you need is 1 angle + 1 side or 2 sides to find anything else
PRACTICE PROBLEM 1 On a safari, a team of naturalists sets out toward a research station located 4.8 km away in a direction 42 north of east. After traveling in a straight line for 2.4 km, they stop and discover that they have been traveling 22 north of east, because their guide misread his compass. What are the magnitude and direction (relative to due east) of the displacement vector now required to bring the team to the research station?
KINEMATICS 5 Kinematic Variables: 3 Equations: o? = ?0+ ?0? +1 oDisplacement, ? or ? 2??2 oFinal velocity, ??or ? o? = ?0+ ?? o?2= ?0 oInitial Velocity, ??or ?? 2+ 2?(? ?0) oAcceleration, ? oTime, ?
KEEP IN MIND Write down everything you have Separate it based on directions and cases Maximum height? vfy= 0 ??= 0 The final for one part of the problem could become the initial for another Time depends on the y portion of the problem Note your positive and negative directions Draw everything!
PRACTICE PROBLEM 2 A jetliner, traveling northward, is landing with a speed of 69m/s. Once the jet touches down, it has 750m of runway in which to reduce its speed to 6.1m/s. Compute the average acceleration (magnitude and direction) of the plane during landing.
PRACTICE PROBLEM 3 A tennis ball is thrown horizontally with and initial speed of 10 m/s. If the ball travels 40m horizontally, how far down did the tennis ball drop?
FORCES AND NEWTONS LAWS 1stLaw: An object continues in a state of rest or a state of motion at a constant speed along a straight line unless compelled to change state by a net force An object won t want to change what it s doing without a force 2ndLaw: When a net external force acts on an object, the acceleration that results is directly proportional to the net force and has a magnitude that is inversely proportional to the mass. The direction of acceleration is the same as the direction of the net force. Basically, ? = ?? 3rdLaw: Whenever one body exerts a force on a second body, the second body exerts and oppositely directed force of equal magnitude on the first body Equal and opposite reactions
TIPS FOR FORCE PROBLEMS Draw a free body diagram. It always helps to see things Write down literally everything Equilibrium = no acceleration, therefore, ? = 0. Use this to your advantage Mass is not always important. It may cancel in the end Normal force is perpendicular to the surface. Not always mg! Friction force is opposite to motion Tension is along a rope Gravitational force is straight down
PRACTICE PROBLEM 4 A 912-kg car is being driven down a straight, level road at a constant speed of 31.5 m/s. When the driver sees a police cruiser ahead, she removes her foot from the accelerator. After 8.00 s, the speed of the car is 24.6 m/s, which is the posted speed limit. What is the magnitude of the average net force acting on the car during the 8.00 s interval?
PRACTICE PROBLEM 5 A child decides to go sledding on a snowy day. The child is initially moving at 4.9 m/s, but eventually slows to a stop. Assuming that the coefficient of kinetic friction is equal to 0.10, and the child s weight is 50kg, what is the friction force? What is the child s acceleration?
PRACTICE PROBLEM 6 A worker stands still on a roof sloped at an angle of 36 above the horizontal. He is prevented from slipping by a static frictional force of 390 N. Find the mass of the worker.
CIRCULAR MOTION Uniform Circular motion has a constant speed, but a changing velocity. Centripetal acceleration points inward, towards the center of the circular motion.
HELPFUL EQUATIONS ? =2?? ? (commonly used for circular orbits) ?? ? (also used for circular orbits. ? = 6.67408 10 11 m3 kg 1 s 2) ??=?2 ? = ? ??= ???= ??2 ? ?? can also be denoted as ???? * Unbanked curves depend on static friction force to provide the centripetal force, whereas on a frictionless banked curve the centripetal force is provided by the horizontal component of normal force (think back to your ramp problems!)
PRACTICE PROBLEM 7 A swing ride at a carnival consists of chairs that are swung in a circle by 15.0-m cables attached to a vertical rotating pole, as the drawing shows. Suppose the total mass of a chair and its occupant is 179 kg. What is the Tension of the cable attached to the chair? What is the speed of the chair?
PRACTICE PROBLEM 8 Civil Engineers generally bank curves on roads in such a manner that a car going around the curve at the recommended speed does not have to rely on friction between its tires and the road surface in order to round the curve. Suppose that the radius of curvature of a given curve is 60m, and the recommended speed is 40km/hr. At what angle should the curve be banked?
PRACTICE PROBLEM 9 Scientists determine the masses of planets by observing the effect of the gravitational field of those planets on nearby objects - mainly upon their moons. By measuring the orbital period and orbital radius of a moon about a planet, Newton's laws of motion can be used to determine the mass of the planet. Phobos, a moon of the planet Mars, was discovered in 1877. It's orbital radius is 9380 km and its orbital period is 0.319 days (2.77 x 104seconds). Determine the mass of Mars based on this data.
WORK AND ENERGY Work = Force * distance * cos? Work can also be measured as change in energy of a system. - Potential Energy (mgh) - Kinetic Energy (1 2m?2)
CONSERVATION OF ENERGY Energy is conserved if the system is only acted on by a conservative force ???= 0 = ?? + ?? Conservative: Nonconservative: Gravity Static and Kinetic friction Elastic spring force Air resistance Electric force Tension Normal force Propulsion of a rocket
PRACTICE PROBLEM 10 A motorcyclist is trying to leap across a canyon by driving horizontally off a 70m high cliff at 38.0 m/s to hit the 35m high cliff on the other side. Ignoring air resistance, find the speed with which the cycle strikes the ground on the other side.
IMPULSE AND MOMENTUM Impulse = Average force * time the force acts ? = ? ? Linear Momentum = mass * velocity ? = ?? Impluse = change in linear momentum ? = ? *Note: If avg external force is 0, linear momentum is conserved.
COLLISIONS Three major types to recognize: 1.) Elastic Objects bounce off of each other Kinetic energy and linear momentum conserved 2.) Inelastic objects may bounce off, but not perfectly Only linear momentum conserved, not kinetic energy 3.) Perfectly Inelastic objects stick together after collision Only linear momentum conserved, not kinetic energy
PRACTICE PROBLEM 11 Starting from rest, two skaters push off against each other on ice where friction is negligible. One is a 54-kg woman and one is a 88-kg man. The woman moves away with a speed of+2.5 m/s. Find the recoil velocity of the man.
ROTATIONAL KINEMATICS Work exactly the same way as linear kinematics Angular Displacement ? ? = ?0+ ?? ? =1 2?0+ ? ? ?2= ?0 ? = ?0? +1 Angular Accelerations Final Angular Velocity ? or ?? 2+ 2?? Initial Angular Velocity ?0or ?? 2??2 Elapsed time ?
CONVERSIONS ??= ?? ??= ?? ? = ?? ? = arc length All are tangental
PRACTICE PROBLEM 12 The wheels of a bicycle have an angular velocity of 20rad/s . Then, the brakes are applied. In coming to rest, each wheel makes an angular displacement of 15.92rev. How much time does it take for the bike to come to rest? What is the angular acceleration (in rad/s2) of the wheels?
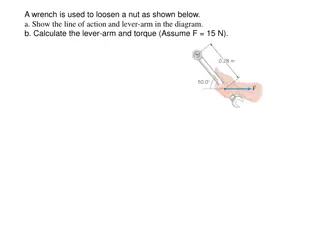
ROTATIONAL DYNAMICS Also known as torque Most problems deal with relating the two equations for torque together. ? = ?? = ?????? ? is measured from the lever arm to the direction of the force The maximum torque will be when ? = 90 , or when ???? = 1
ROTATIONAL DYNAMICS Rotational Kinetic Energy: Angular Momentum: ???= ?? =1 ? = ?? 2??2 Always conserved when no outside torques act on the system ? must be expressed in rad/s Side note: The torque is the rate of change of the angular momentum
THINGS TO REMEMBER If something is in equilibrium: ? = 0 ? = 0 ? = 0 ? = 0 In general, ? = ??2 for the whole system Formulas for specific moments of inertia will be provided
PRACTICE PROBLEM 13 A hiker, who weighs 985 N, is strolling through the woods and crosses a small horizontal bridge. The bridge is uniform, weighs 3610 N, and rests on two concrete supports, one at each end. He stops one-fifth of the way along the bridge. What is the magnitude of the force that a concrete support exerts on the bridge at the near end and at the far end?
PRACTICE PROBLEM 14 A bowling ball encounters a 0.760-m vertical rise on the way back to the ball rack, as the drawing illustrates. Ignore frictional losses and assume that the mass of the ball is distributed uniformly. The translational speed of the ball is 3.50 m/s at the bottom of the rise. Find the translational speed at the top.
PRACTICE PROBLEM 15 Two disks are rotating about the same axis. Disk A has a moment of inertia of 3.4kg ?2 and an angular velocity of 7.2 rad/s. Disk B is rotating with an angular velocity of -9.8rad/s. The two disks are then linked together without the aid of any external torques, so that they rotate as a single unit with an angular velocity of -2.4rad/s. The axis of rotation for this unit is the same as that for the separate disks. What is the moment of inertia of disk B?
HARMONIC MOTION ? is known as the spring constant (how stiff the spring is) ???????= ?? ?? This is how much force you apply to compress or stretch the string The restoring force of an ideal spring (Hooke s Law) is ? = ??