Mathematics Department Overview for New GCSE Curriculum
The Mathematics Department is implementing changes to the curriculum, including a new grading system. End-of-term tests cover specific units for each year group, and SMSC aspects are being integrated into lessons. The programme of study for Year 10 Foundation focuses on various topics and assessments throughout the academic year.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
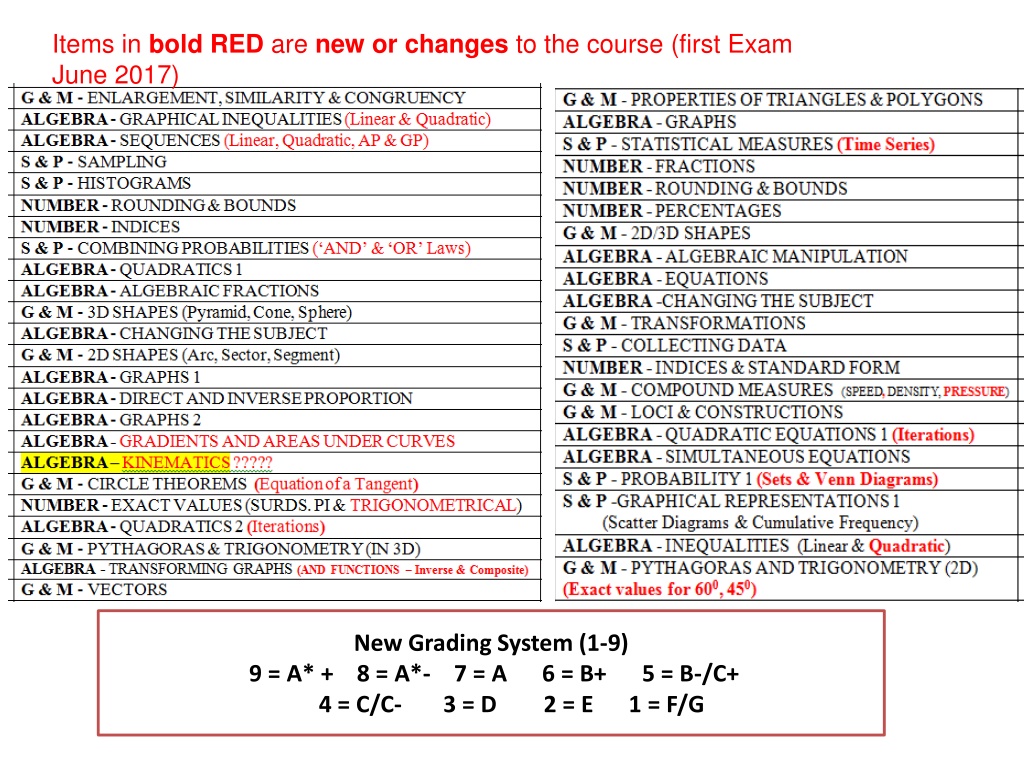
Items in bold RED are new or changes to the course (first Exam June 2017) New Grading System (1-9) 9 = A* + 8 = A*- 7 = A 6 = B+ 5 = B-/C+ 4 = C/C- 3 = D 2 = E 1 = F/G
The end of term tests cover the units in the three-year scheme of work as follows: Year 9 tests cover the below units: end of term 1: units 1 2 end of term 2: units 3 5 end of term 3: units 6 8 Year 10 tests cover the below units: end of term 4: units 9 11 end of term 5: units 12 14 end of term 6: units 15 17 Year 11 tests cover the below units: end of term 7: units 18 20 (F) and 18 19 (H)
SMSC in Mathematics The maths SOW are currently being revamped with new changes on the horizon. It is intended through various projects, mini investigations and existing activities built into lessons, SMSC, (Spiritual, Moral, Social and Cultural) will be delivered in high quality lessons. Ideas below in draft form will form the foundation addressing the issues. All the objectives and activities which are addressing SMSC are highlighted in RED. British Values The mathematics curriculum promotes the British values of tolerance and resilience on A daily basis through problem solving and understanding of complex concepts, encouraging students to persevere and try different methods to arrive at a correct solution. Teamwork through peer assessment and group work underpins the schemes of learning In the maths faculty. Students work together in all areas of the maths curriculum to support each other and build mutual respect for one another. Students are allowed to make mistakes and learn from them in all maths lessons. This fosters confidence and builds self-- esteem, it encourages students to take risks and become lifelong learners whilst using their mathematical skills in all aspects of life.
Maths Department Programme of Study for New GCSE- Year 10 Foundation (2021-22) Wk9 1 -5 Nov Wk8 18- 22Oct Wk1 2-3Sept Wk2 6-10 Sept Wk3 13-17 Sept Wk4 20-24 Sep Wk5 27-1 Oct Wk6 4-8 Oct Wk7 11-15Oct Half 9a.Real-life graphs 10a. 10b. 9b. Straight-line graphs INSET DAYS 11a.Ratio Assess ment Transformations I Transformations II Term Wk16 4-7 Jan Wk17 10-14 Jan Wk10 8 - 12 Nov Wk11 15-19 Nov Wk12 22-26 Nov Wk13 29-3 Dec Wk14 6-10 Dec Wk15 13-17 Dec Christmas Holiday 11b.Propo rtions 12.Right-angled triangles: Pythagoras and trigonometry 13a 13b Assessment Probability I Probability II Wk18 17-21 Jan Wk19 24-28 Jan Wk20 31-4 Feb Wk21 7-11 Feb Wk23 28- 4Mar Wk24 7-11 Mar Wk25 14-18 Mar Wk26 21-25 Mar Wk22 14-18 Feb Half 16a.Quadratic equations: expanding and factorising 15b.Constructions, loci and bearings 15a.Plans and elevations. 14.Multiplicative reasoning Assessment Term Wk33 23- 27 Jun Wk27 28-1 Apr Wk28 4-8 Apr Wk29 25-29 April Wk30 2-6 May Wk31 9-13 May Wk32 16-20 May Half 16b Quadratic equations: graphs . 16b Quadratic equations: graphs . 17.Circles, cylinders, cones and spheres Term Easter Holiday Wk34 6-10 Jun Wk35 13-17 Jun Wk36 20-24 Jun Wk37 27-1 Jul Wk38 4-8 Jul Wk39 11-15 July Wk39 18-22 July Start of Summer 17.Circles, cylinders, cones and spheres Work experience Mock exam Revision
Year10 Foundation TIER Autumn1TERM TIME ALLOCATION: 8-10 hours 9a.Real-life graphs KEY WORDS STARTER(S) PRIOR KNOWLEDGE Students should be able to plot coordinates and read scales Students should be able to substitute into a formula. Linear, graph, distance, time, coordinate, quadrant, real-life graph, gradient, intercept, function, solution, parallel . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT OBJECTIVES By the end of the sub-unit, students should be able to: Use input/output diagrams; (Grade G+/1) Use axes and coordinates to specify points in all four quadrants in 2D; Identify points with given coordinates and coordinates of a given point in all four quadrants; (grade G+/1) Find the coordinates of points identified by geometrical information in 2D (all four quadrants);(Grade F-/1) Find the coordinates of the midpoint of a line segment; Draw, label and scale axes; (Grade E/2) Read values from straight-line graphs for real-life situations; Draw straight line graphs for real-life situations, including ready reckoner graphs, conversion graphs, fuel bills graphs, fixed charge and cost per unit; (Grade D/3) Draw distance time graphs and velocity time graphs; Work out time intervals for graph scales; (grade C-/4) Interpret distance time graphs, and calculate: the speed of individual sections, total distance and total time; (SMSC) Interpret information presented in a range of linear and non-linear graphs; (Grade C-/4) Interpret graphs with negative values on axes; (Grade C/5) Interpret gradient as the rate of change in distance time and speed time graphs, graphs of containers filling and emptying, and unit price graphs. (Grade B-/5)(SMSC) Clip 24 Distance Tables Clip 43 Conversion graphs Clip 117 Real-life graphs KM: Proportion for real KM: Investigating proportionality(SMSC)(BV) PLENARIES/KEY QUESTIONS With distance time graphs, students struggle to understand that the perpendicular distance from the x-axis represents distance. Clear presentation of axes is important.
Year10 TIME ALLOCATION: 5-7hours Foundation TIER Autumn1TERM 9b. Straight-line graphs KEY WORDS STARTER(S) PRIOR KNOWLEDGE Linear, graph, distance, time, coordinate, quadrant, real-life graph, gradient, intercept, function, solution, parallel . Students should be able to plot coordinates and read scales Students should be able to substitute into a formula. LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT OBJECTIVES By the end of the sub-unit, students should be able to: Use function machines to find coordinates (i.e. given the input x, find the output y); (Grade E/2) Plot and draw graphs of y = a, x = a, y = x and y = x;(Grade D/3) Recognise straight-line graphs parallel to the axes; (Grade D/3) Recognise that equations of the form y = mx + c correspond to straight-line graphs in the coordinate plane; (Grade D/3) Plot and draw graphs of straight lines of the form y = mx + c using a table of values; (Grade D/3) Sketch a graph of a linear function, using the gradient and y-intercept; (Grade c-/4) Identify and interpret gradient from an equation y = mx + c; Identify parallel lines from their equations; (Grade C+/5) Plot and draw graphs of straight lines in the form ax + by = c; Find the equation of a straight line from a graph; (Grade B-/5) Find the equation of the line through one point with a given gradient; (Grade B-/5) Find approximate solutions to a linear equation from a graph; Find the gradient of a straight line from real-life graphs too. (Grade B-/5) Clip 24 Distance Tables Clip 43 Conversion graphs Clip 117 Real-life graphs KM: Matching graphs KM: Autograph 2 KM: The hare and the tortoise(SMSC)(BV) PLENARIES/KEY QUESTIONS Plot and draw the graph for y = 2x 4. Which of these lines are parallel: y = 2x + 3, y = 5x + 3, y = 2x 9, 2y = 4x 8 When not given a table of values, students rarely see the relationship between the coordinate axes(BV)
Y10 Foundation TIER Autumn2TERM TIME ALLOCATION: 5-7hours 10a. Transformations I: rotations and translations PRIOR KNOWLEDGE KEY WORDS Students should recall basic shapes. Students should be able to plot points in all four quadrants. Students should have an understanding of the concept of rotation. Students should be able to draw and recognise lines parallel to axes and y = x, y = x. Transformation, rotation, reflection, enlargement, translation, single, combination, scale factor, mirror line, centre of rotation, centre of enlargement, column vector, vector, similarity, congruent, angle, direction, coordinate, describe LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT Clip 74 Rotations Clip 75 Reflections Clip 76 Enlargements Clip 77 Translations Clip 83 Symmetries (Link with Ch1) KM: Transformation template KM: Enlargement KM: Investigating transformations with Autograph (SMSC)(BV) OBJECTIVES By the end of the sub-unit, students should be able to: Identify congruent shapes by eye; (Grade G-/0) Understand clockwise and anticlockwise;(Grade G+/1) Understand that rotations are specified by a centre, an angle and a direction of rotation;(Grade E/2) Find the centre of rotation, angle and direction of rotation and describe rotations;(Grade E/2) Describe a rotation fully using the angle, direction of turn, and centre; Rotate a shape about the origin or any other point on a coordinate grid; Draw the position of a shape after rotation about a centre (not on a coordinate grid); (Grade E/2) Identify correct rotations from a choice of diagrams; Understand that translations are specified by a distance and direction using a vector;(Grade D/3) Translate a given shape by a vector; Describe and transform 2D shapes using single translations on a coordinate grid; Use column vectors to describe translations; Understand that distances and angles are preserved under rotations and translations, so that any figure is congruent under either of these transformations.(Grade D/3) PLENARIES/KEY QUESTIONS The directions on a column vector often get mixed up. Student need to understand that the units of movement are those on the axes, and care needs to be taken to check the scale. Correct language must be used: students often use turn rather than rotate .(SMSC)(BV)
Year10 Foundation TIER Autumn2TERM 10b. Transformations II: reflections and enlargements TIME ALLOCATION: 7-9hours KEY WORDS PRIOR KNOWLEDGE Students should recall basic shapes. Students should be able to plot points in all four quadrants. Students should have an understanding of the concept of rotation. Students should be able to draw and recognise lines parallel to axes and y = x, y = x. Transformation, rotation, reflection, enlargement, translation, single, combination, scale factor, mirror line, centre of rotation, centre of enlargement, column vector, vector, similarity, congruent, angle, direction, coordinate, describe LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT OBJECTIVES By the end of the sub-unit, students should be able to: Understand that reflections are specified by a mirror line; (Grade E/2) Identify correct reflections from a choice of diagrams; (Grade E/2) Understand that reflections are specified by a mirror line;(Grade E/2) Identify the equation of a line of symmetry;(Grade D/3) Transform 2D shapes using single reflections (including those not on coordinate grids) with vertical, horizontal and diagonal mirror lines; (Grade D/3) Describe reflections on a coordinate grid; Scale a shape on a grid (without a centre specified); (Grade D/3) Understand that an enlargement is specified by a centre and a scale factor; Enlarge a given shape using (0, 0) as the centre of enlargement, and enlarge shapes with a centre other than (0, 0);(Grade D/3) Find the centre of enlargement by drawing; (Grade C-/4) Describe and transform 2D shapes using enlargements by: a positive integer scale factor;(Grade C-/4) a fractional scale factor;(Grade C-/4) Identify the scale factor of an enlargement of a shape as the ratio of the lengths of two corresponding sides, simple integer scale factors, or simple fractions; Understand that distances and angles are preserved under reflections, so that any figure is congruent under this transformation;(Grade B-/5) Understand that similar shapes are enlargements of each other and angles are preserved define similar in this unit;(Grade B-/5) Describe and transform 2D shapes using combined rotations, reflections, translations, or enlargements.(Grade C+/5) Clip 74 Rotations Clip 75 Reflections Clip 76 Enlargements Clip 77 Translations Clip 83 Symmetries (Link with Ch1) KM: Enlargement II NRICH: Who s the fairest of them all? PLENARIES/KEY QUESTIONS Describe and transform a given shape by a reflection. Convince me the scale factor is, for example, 2.5
Year10 Foundation TIER Autumn1TERM 11a. Ratio TIME ALLOCATION: 5-7hours KEY WORDS PRIOR KNOWLEDGE Students should know the four operations of number. Students should have a basic understanding of fractions as being parts of a whole . Ratio, proportion, share, parts, fraction, function, direct proportion, inverse proportion, graphical, linear, compare LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT By the end of the sub-unit, students should be able to: Understand and express the division of a quantity into a of number parts as a ratio;(Grade E/2) Write ratios in their simplest form; (Grade E/2) Write/interpret a ratio to describe a situation; Share a quantity in a given ratio including three-part ratios; Solve a ratio problem in context( Grade E/2) use a ratio to find one quantity when the other is known; use a ratio to compare a scale model to a real-life object; (Grade D/3) use a ratio to convert between measures and currencies; (Grade D/3) problems involving mixing, e.g. paint colours, cement and drawn conclusions;(Grade D/3)(SMSC) Compare ratios; (Grade C-/4) Write ratios in form 1 : m or m : 1; Write a ratio as a fraction; Write a ratio as a linear function; Write lengths, areas and volumes of two shapes as ratios in simplest form; (Grade C+/5) Express a multiplicative relationship between two quantities as a ratio or a fraction.(Grade B-/5) Clip 64 Real-life Money Questions Clip 94 Ratio 2 Clip 50 Value for Money Clip 61 Ratio 1 KM: Maths to Infinity: Fractions, decimals, percentages, ratio, proportion NRICH: In proportion NRICH: Ratio or proportion? PLENARIES/KEY QUESTIONS Emphasise the importance of reading the question carefully. Include ratios with decimals 0.2 : 1. Converting imperial units to imperial units aren t specifically in the programme of study, but still useful and provide a good context for multiplicative reasoning. It is also useful generally for students to know rough metric equivalents of commonly used imperial measures, such as pounds, feet, miles and pints.(SMSC)(BV)
Year10 Foundation TIER Autumn2TERM TIME ALLOCATION: 5-7hours 11b. Proportion KEY WORDS PRIOR KNOWLEDGE Ratio, proportion, share, parts, fraction, function, direct proportion, inverse proportion, graphical, linear, compare . Students should know the four operations of number. Students should have a basic understanding of fractions as being parts of a whole . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT By the end of the sub-unit, students should be able to: Understand and use proportion as equality of ratios; Solve word problems involving direct and indirect proportion;(Grade C-/4) Work out which product is the better buy; Scale up recipes;(grade C-/4)(SMSC) Convert between currencies;(Grade B-/5)(BV)(SMSC) Find amounts for 3 people when amount for 1 given; Solve proportion problems using the unitary method;(Grade C-/4) Recognise when values are in direct proportion by reference to the graph form; (Grade B-/5) Understand inverse proportion: as x increases, y decreases (inverse graphs done in later unit); (Grade B-/5) Recognise when values are in direct proportion by reference to the graph form; (Grade C+/5) Understand direct proportion ---> relationship y = kx.(Grade C+/5) Clip 23 Proportion Clip 50 Value for Money Clip 62 Proportion Recipe Type Questions Clip 64 Real-life Money Questions KM: Stick on the Maths: Proportional reasoning PLENARIES/KEY QUESTIONS Recognise that two paints mixed red to yellow 5 : 4 and 20 : 16 are the same colour. If it takes 2 builders 10 days to build a wall, how long will it take 3 builders? (BV)(SMSC) Scale up recipes and decide if there is enough of each ingredient. Given two sets of data in a table, are they in direct proportion? Find out/prove whether two variables are in direct proportion by plotting the graph and using it as a model to read off other values. Possible link with scatter graphs. (SMSC)(BV)
Year10 Foundation TIER 12.Right-angled triangles: Pythagoras and trigonometryTIME ALLOCATION: 5-7hours Spring1 KEY WORDS PRIOR KNOWLEDGE Students should be able to rearrange simple formulae and equations, as preparation for rearranging trigonometric formulae. Students should recall basic angle facts. Students should understand when to leave an answer in surd form. Students can plot coordinates in all four quadrants and draw axes. Triangle, right angle, angle, Pythagoras Theorem, sine, cosine, tan, trigonometry, opposite, hypotenuse, adjacent, ratio, elevation, depression, length, accuracy . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT Understand, recall and use Pythagoras Theorem in 2D, including leaving answers in surd form; Given 3 sides of a triangle, justify if it is right-angled or not;(Grade C-/4) Calculate the length of the hypotenuse in a right-angled triangle, including decimal lengths and a range of units;(Grade C-/4) Find the length of a shorter side in a right-angled triangle;(Grade C/5) Apply Pythagoras Theorem with a triangle drawn on a coordinate grid;(Grade B-/5) Calculate the length of a line segment AB given pairs of points; (Grade B-/5) Understand, use and recall the trigonometric ratios sine, cosine and tan, and apply them to find angles and lengths in general triangles in 2D figures; (Grade B-/5) Use the trigonometric ratios to solve 2D problems; Find angles of elevation and depression; (Grade B-/5) Round answers to appropriate degree of accuracy, either to a given number of significant figures or decimal places, or make a sensible decision on rounding in context of question;(Grade B-/5) Know the exact values of sin and cos for = 0 , 30 , 45 , 60 and 90 ; know the exact value of tan for = 0 , 30 , 45 and 60 . KM: Stick on the Maths: Pythagoras Theorem KM: Stick on the Maths: Right Prisms Clip 118 Pythagoras Theorem Clip 119 Pythagoras- Line on a Graph Clip 147 Trigonometry PLENARIES/KEY QUESTIONS Students may need reminding about surds. Drawing the squares on the 3 sides will help to illustrate the theorem. Include examples with triangles drawn in all four quadrants. Scale drawings are not acceptable. Calculators need to be in degree mode.(SMSC) To find in right-angled triangles the exact values of sin and cos for = 0 , 30 , 45 , 60 and 90 , use triangles with angles of 30 , 45 and 60 . Use a suitable mnemonic to remember SOHCAHTOA. Use Pythagoras Theorem and trigonometry together
Year10 TIME ALLOCATION: 4-6hours Foundation TIER Spring1 13aProbability I KEY WORDS PRIOR KNOWLEDGE Students should know how to add and multiply fractions and decimals. Students should have experience of expressing one number as a fraction of another number. Probability, dependent, independent, conditional, tree diagrams, sample space, outcomes, theoretical, relative frequency, fairness, experimental . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT Distinguish between events which are impossible, unlikely, even chance, likely, and certain to occur; (Grade G+/1) Mark events and/or probabilities on a probability scale of 0 to 1; Write probabilities in words or fractions, decimals and percentages; Find the probability of an event happening using theoretical probability; (Grade F/1)(SMSC)(BV) Use theoretical models to include outcomes using dice, spinners, coins; List all outcomes for single events systematically; (Grade F/1) Work out probabilities from frequency tables; (Grade E/2) Work out probabilities from two-way tables; (Grade E/2) Record outcomes of probability experiments in tables; Add simple probabilities; Identify different mutually exclusive outcomes and know that the sum of the probabilities of all outcomes is 1; (Grade D/3) Using 1 p as the probability of an event not occurring where p is the probability of the event occurring; (Grade C-/4) Find a missing probability from a list or table including algebraic terms. (Grade C-/4) KM: Sample spaces KM: Race game NRICH: Prize Giving (frequency trees Clip 85 Two-Way Tables Clip 40 The probability Scale Clip 90 List of Outcomes Clip 91 Mutually Exclusive Events Clip 132 Experimental Probabilities PLENARIES/KEY QUESTIONS Mark events on a probability scale and use the language of probability.(SMSC) If the probability of outcomes are x, 2x, 4x, 3x calculate x.(SMSC) Calculate the probability of an event from a two-way table or frequency table. Decide if a coin, spinner or game is fair. (SMSC)
Year10 Foundation TIER Spring1 TIME ALLOCATION: 8-10hours 13b. Probability II KEY WORDS PRIOR KNOWLEDGE Students should know the four operations of number. Students should have a basic understanding of fractions as being parts of a whole . Ratio, proportion, share, parts, fraction, function, direct proportion, inverse proportion, graphical, linear, compare . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT KM: Sample spaces KM: Race game NRICH: Prize Giving (frequency trees Clip 85 Two-Way Tables Clip 40 The probability Scale Clip 90 List of Outcomes Clip 91 Mutually Exclusive Events Clip 132 Experimental Probabilities Find the probability of an event happening using relative frequency; Estimate the number of times an event will occur, given the probability and the number of trials for both experimental and theoretical probabilities; (Grade D/3) List all outcomes for combined events systematically; Use and draw sample space diagrams; (Grade C-/4)(SMSC) Work out probabilities from Venn diagrams to represent real-life situations and also abstract sets of numbers/values; Use union and intersection notation; Compare experimental data and theoretical probabilities; (Grade D/4) Compare relative frequencies from samples of different sizes; (Grade C-/4) Find the probability of successive events, such as several throws of a single dice; Use tree diagrams to calculate the probability of two independent events; (Grade B-/5) Use tree diagrams to calculate the probability of two dependent events. (Grade B-/5) PLENARIES/KEY QUESTIONS Recognise that two paints mixed red to yellow 5 : 4 and 20 : 16 are the same colour. If it takes 2 builders 10 days to build a wall, how long will it take 3 builders? (SMSC)(BV) Scale up recipes and decide if there is enough of each ingredient. Given two sets of data in a table, are they in direct proportion? Find out/prove whether two variables are in direct proportion by plotting the graph and using it as a model to read off other values. Possible link with scatter graphs. (SMSC)(BV)
Year10 Foundation TIER 14.Multiplicative reasoningTIME ALLOCATION: 6-8hours Spring2 KEY WORDS PRIOR KNOWLEDGE Students should be able to interpret scales on a range of measuring instruments. Students should be able to find a percentage of an amount and relate percentages to decimals. Students should be able to rearrange equations and use these to solve problems. Students should know speed = distance/time, density = mass/volume Ratio, proportion, best value, proportional change, compound measure, density, mass, volume, speed, distance, time, density, mass, volume, pressure, acceleration, velocity, inverse, direct . LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT Understand and use compound measures: (Grade D-C) 1. density; 2.pressure; 3.speed: convert between metric speed measures;(Grade D/3)(SMSC) read values in km/h and mph from a speedometer; calculate average speed, distance, time in miles per hour as well as metric measures; use kinematics formulae from the formulae sheet to calculate speed, acceleration (with variables defined in the question);(Grade C-/4)(SMSC) change d/t in m/s to a formula in km/h, i.e. d/t (60 60)/1000 with support; Express a given number as a percentage of another number in more complex situations; (Grade C-/4)(SMSC)(BV) Calculate percentage profit or loss;(grade C-/4) Make calculations involving repeated percentage change, not using the formula; Find the original amount given the final amount after a percentage increase or decrease;(Grade B-/5) Use compound interest;(Grade C/5)(SMSC)(BV) Use a variety of measures in ratio and proportion problems(Grade B-/5) currency conversion; rates of pay; best value; Set up, solve and interpret the answers in growth and decay problems;(grade C+/5) Understand that X is inversely proportional to Y is equivalent to X is proportional to ; Interpret equations that describe direct and inverse proportion. (Grade C+/5) NCETM: Multiplicative reasoning(SMSC)(BV) Show me an example of two quantities that will be in proportion. And another. And another (Showing a table of values such as the one Which is the faster speed: 60 km/h or 10 m/s? Explain why. (BV) PLENARIES/KEY QUESTIONS Know that measurements using real numbers depend upon the choice of unit, with speedometers and rates of change. Change m/s to km/h. Understand direct proportion as: as x increase, y increases. Understand inverse proportion as: as x increases, y decreases. Some students may think that compound interest and simple interest are the same method of calculating interest. Incomplete methods when using multipliers, i.e. reduce 80 by 15% = 80 0.15.(SMSC)(BV)
Year10 Foundation TIER 15a.Plans and elevations TIME ALLOCATION: 5-7hours Spring2 KEY WORDS PRIOR KNOWLEDGE Construct, circle, arc, sector, face, edge, vertex, two- dimensional, three-dimensional, solid, elevations, congruent, angles, regular, irregular, bearing, degree, bisect, perpendicular, loci, map, scale, plan, region Students should be able to measure and draw lines. LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT KM: Construction instruction KM: Construction challenges KM: Locus hocus pocus KM: The perpendicular bisector KM: Topple KM: An elevated position KM: Solid problems (plans and elevations) KM: Isometric interpretation (plans and elevations)(SMSC) PLENARIES/KEY QUESTIONS Be able to estimate the size of given angles. Convert fluently between metric units of length. Use bearings in a real-life context to describe the bearing between two towns on a map. Some pupils may use the wrong scale of a protractor. For example, they measure an obtuse angle as 60 rather than as 120 . Often 5 sides only are drawn for a cuboid. This is a very practical topic, and provides opportunities for some hands-on activities. Whilst not an explicit objective, it is useful for students to draw and construct nets and show how they fold to make 3D solids, allowing students to make the link between 3D shapes and their nets. This will enable students to understand that there is often more than one net that can form a 3D shape.(SMSC)(BV) Understand clockwise and anticlockwise; (Grade G+/1) Draw circles and arcs to a given radius or given the diameter; (Grade G+/1) Measure and draw lines, to the nearest mm; (Grade G+/1) Measure and draw angles, to the nearest degree; (Grade G+/1) Know and use compass directions;(Grade G+/1 ) Draw sketches of 3D solids; Know the terms face, edge and vertex; (Grade F/1) Identify and sketch planes of symmetry of 3D solids;(Grade D/3) Use isometric grids to draw 2D representations of 3D solids; Make accurate drawings of triangles and other 2D shapes using a ruler and a protractor; (Grade D/3) Construct diagrams of everyday 2D situations involving rectangles, triangles, perpendicular and parallel lines; (Grade D/3) Understand and draw front and side elevations and plans of shapes made from simple solids; (grade C-/4) Given the front and side elevations and the plan of a solid, draw a sketch of the 3D solid. (grade C-/4)(BV)(SMSC)
Year10 Foundation TIER Summer1 TIME ALLOCATION: 9-11hours 15b.Constructions, loci and bearings KEY WORDS PRIOR KNOWLEDGE Construct, circle, arc, sector, face, edge, vertex, two- dimensional, three-dimensional, solid, elevations, congruent, angles, regular, irregular, bearing, degree, bisect, perpendicular, loci, map, scale, plan, region RESOURCES/ACTIVITIES/ICT KM: Construction instruction KM: Construction challenges KM: Napoleonic challenge KM: Locus hocus pocus Students should be able to measure and draw lines. LEARNING OBJECTIVES Understand congruence, as two shapes that are the same size and shape; (Grade E/2) Visually identify shapes which are congruent; (Grade E/2) Use straight edge and a pair of compasses to do standard constructions:(Grade D/3) understand, from the experience of constructing them, that triangles satisfying SSS, SAS, ASA and RHS are unique, but SSA triangles are not;(Grade D/3) construct the perpendicular bisector of a given line; (Grade C-/4) construct the perpendicular from a point to a line; (Grade C-/4) construct the bisector of a given angle;(Grade C-/4) construct angles of 90 , 45 ; (Grade C-4) Draw and construct diagrams from given instructions, including the following: a region bounded by a circle and an intersecting line; (Grade C-/4) a given distance from a point and a given distance from a line; (Grade C/5) equal distances from two points or two line segments; (Grade C/5) regions may be defined by nearer to or greater than ; (Grade C/5)(SMSC)(BV) Find and describe regions satisfying a combination of loci; (Grade C/5) Use constructions to solve loci problems (2D only); (Grade C/5) Use and interpret maps and scale drawings; (Grade C/5) Estimate lengths using a scale diagram; (Grade C/5) Make an accurate scale drawing from a diagram;(Grade D/3) Use three-figure bearings to specify direction; (Grade D/3) Mark on a diagram the position of point B given its bearing from point A; Give a bearing between the points on a map or scaled plan; (Grade C-/4) Given the bearing of a point A from point B, work out the bearing of B from A; Use accurate drawing to solve bearings problems; Solve locus problems including bearings.(Grade C-/4)(SMSC)(BV) PLENARIES/KEY QUESTIONS Sketch the locus of point on a vertex of a rotating shape as it moves along a line, i.e. a point on the circumference or at the centre of a wheel. COMMON MISCONCEPTIONS Correct use of a protractor may be an issue. Drawings should be done in pencil. Relate loci problems to real-life scenarios, including mobile phone masts and coverage.(SMSC)(BV) Construction lines should not be erased.
Year10 Summer1 Foundation TIER 16a. Quadratic equations: expanding and factorising Time allocation:4-6 hours KEY WORDS PRIOR KNOWLEDGE Students should be able to square negative numbers. Students should be able to substitute into formulae. Students should be able to plot points on a coordinate grid. Students should be able to expand single brackets and collect like terms. LEARNING OBJECTIVES Define a quadratic expression; Multiply together two algebraic expressions with brackets;(Grade B-/5) Square a linear expression, e.g. (x + 1)2;(Grade B/5) Factorise quadratic expressions of the form x2 + bx + c; Factorise a quadratic expression x2 a2 using the difference of two squares; (Grade B-/5) Solve quadratic equations by factorising; (Grade B-/5) Find the roots of a quadratic function algebraically. Grade B- /5) Quadratic, function, solve, expand, factorise, simplify, expression, graph, curve, factor, coefficient, bracket RESOURCES/ACTIVITIES/ICT Clip 105 Solving equations Clip 106 Forming Equations KM: Stick on the Maths: Multiplying linear expressions KM: Maths to Infinity: Brackets KM: Maths to Infinity: Quadratics NRICH: Pair Products NRICH: Multiplication Square PLENARIES/KEY QUESTIONS Solve 3x2 + 4 = 100. Expand (x + 2)(x + 6). Factorise x2 + 7x + 10. Solve x2 + 7x + 10 = 0. Solve (x 3)(x + 4)= 0. COMMON MISCONCEPTIONS xterms can sometimes be collected with x2. This unit can be extended by including quadratics where a 1. Emphasise the fact that x2 and x are different types of term illustrate this with numbers.
Year10 Summer2 Foundation TIER 16b. Quadratic equations: expanding and factorising Time allocation: 3-5hours KEY WORDS PRIOR KNOWLEDGE Students should be able to square negative numbers. Students should be able to substitute into formulae. Students should be able to plot points on a coordinate grid. Students should be able to expand single brackets and collect like terms. LEARNING OBJECTIVES Generate points and plot graphs of simple quadratic functions, then more general quadratic functions; (grade C+/5) Identify the line of symmetry of a quadratic graph; (Grade B-/5) Find approximate solutions to quadratic equations using a graph; Interpret graphs of quadratic functions from real-life problems;(Grade B-/5) Identify and interpret roots, intercepts and turning points of quadratic graphs.(Grade B-/5) Quadratic, function, solve, expand, factorise, simplify, expression, graph, curve, factor, coefficient, bracket RESOURCES/ACTIVITIES/ICT NRICH: How old am I? NRICH: Golden thoughts Hwb: Algebra Fails PLENARIES/KEY QUESTIONS POSSIBLE SUCCESS CRITERIA Recognise a quadratic graph from its shape. COMMON MISCONCEPTIONS Squaring negative numbers can be a problem. NOTES The graphs should be drawn freehand and in pencil, joining points using a smooth curve. Encourage efficient use of the calculator. Extension work can be through plotting cubic and reciprocal graphs, solving simultaneous equations graphically. (BV)(SMSC)
Year10 Summer2 Foundation TIER 17: Perimeter, area and volume 2: circles, cylinders, cones and spheres Time allocation: 6-8hours KEY WORDS PRIOR KNOWLEDGE Students should know the formula for calculating the area of a rectangle. Students should know how to use the four operations on a calculator. Area, perimeter, formula, length, width, measurement, volume, circle, segment, arc, sector, cylinder, circumference, radius, diameter, pi, sphere, cone, hemisphere, segment, accuracy, surface area LEARNING OBJECTIVES RESOURCES/ACTIVITIES/ICT NRICH: Surface Area and Volume Hwb: Summerhouse and Radiators(SMSC)(BV) Clip 39 Isometric Drawing Clip 82 Nets Clip 81 Plans and Elevations Clip 34 Volume of Cuboids Clip 122 Volume of a prism PLENARIES/KEY QUESTIONS Recall terms related to a circle. Understand that answers in terms of pi are more accurate. COMMON MISCONCEPTIONS Diameter and radius are often confused and recollection which formula to use for area and circumference of circles is often poor. Emphasise the need to learn the circle formula: Cherry Pie s Delicious and Apple Pies are too are good ways to remember them. Formulae for curved surface area and volume of a sphere, and surface area and volume of a cone, will be given on the formulae sheet in the examination. Ensure that students know it is more accurate to leave answers in terms of but only when asked to do so. Recall the definition of a circle; Identify, name and draw parts of a circle including tangent, chord and segment; (Grade D/3) Recall and use formulae for the circumference of a circle and the area enclosed by a circle circumference of a circle = 2 r = d, area of a circle = r2; Find circumferences and areas enclosed by circles; (Grade C-/4) Use 3.142 or use the button on a calculator; (Grade C-/4) Give an answer to a question involving the circumference or area of a circle in terms of ; (Grade C-/4) Find radius or diameter, given area or perimeter of a circles; Find the perimeters and areas of semicircles and quarter-circles; (GradeC- /4) Calculate perimeters and areas of composite shapes made from circles and parts of circles; (Grade C/5) Calculate arc lengths, angles and areas of sectors of circles; (Grade B/5) Find the surface area of a cylinder; (Grade B/5) Find the volume of a cylinder; (Grade B/5) Find the surface area and volume of spheres, pyramids, cones and composite solids; (Grade B/5) Round answers to a given degree of accuracy. (Grade B/5)