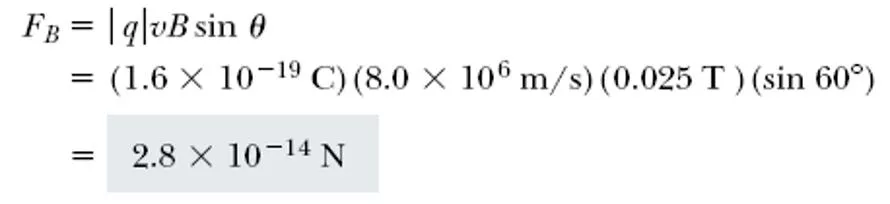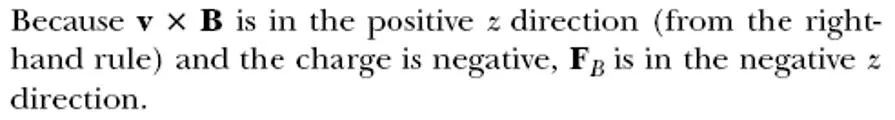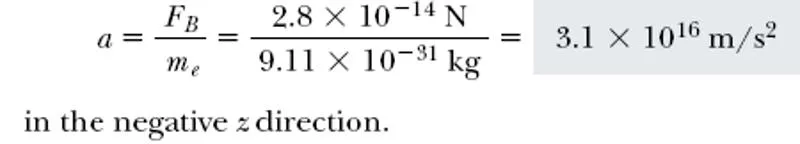Magnetic Force and Acceleration of Electrons in Television Picture Tubes

An electron in a television picture tube is analyzed as it moves towards the front of the tube in a magnetic field. The magnetic force and acceleration of the electron are calculated, along with determining the linear speed of a proton moving in a circular orbit under a magnetic field. Additionally, an experiment measuring the magnitude of a uniform magnetic field by accelerating electrons through a potential difference is discussed, including finding the field's magnitude and the angular speed of the electrons.

• Magnetic force
• Acceleration
• Electrons
• Television picture tube
• Proton

briony Follow

Uploaded on Jul 23, 2024 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

Presentation Transcript

1. Chapter 29 Examples Examples 1, 6, 7

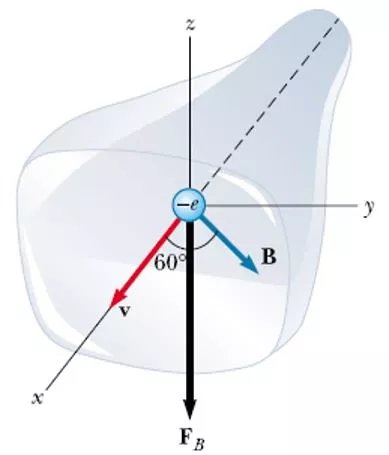
2. An electron in a television picture tube moves toward the front of the tube with a speed of 8.0 x 106 m/s along the x axis. Surrounding the neck of the tube are coils of wire that create a magnetic field of magnitude 0.025 T, directed at an angle of 60 to the x axis and lying in the xy plane. Calculate the magnetic force on and acceleration of the electron.

3. A proton is moving in a circular orbit of radius 14 cm in a uniform 0.35-T magnetic field perpendicular to the velocity of the proton. Find the linear speed of the proton.

4. Example 29.7 In an experiment designed to measure the magnitude of a uniform magnetic field, electrons are accelerated from rest through a potential difference of 350 V. The electrons travel along a curved path because of the magnetic force exerted on them, and the radius of the path is measured to be 7.5 cm. (Fig. 29.20 shows such a curved beam of electrons.) If the magnetic field is perpendicular to the beam, (A) what is the magnitude of the field? (B) What is the angular speed of the electrons? Figure 29.20 (Example 29.7) The bending of an electron beam in a magnetic field.

5. To begin analyzing the problem, we find the electron speed. For the isolated electronelectric field system, the loss of potential energy as the electron moves through the 350-V potential difference appears as an increase in the kinetic energy of the electron. Because K I = 0 and , we have Kf =1/2me v 2 we have