Effects of Shallow Water on Ship Performance and Speed
When a ship enters shallow water, various changes occur, affecting velocity, pressure, sinkage, and trim. The interaction between the ship and seabed leads to increased drag and wave resistance. The depth Froude number helps understand the impact of shallow water on ship speed. Corrections for shallow water effects are crucial, especially for high-speed vessels. Considerations for limited data on the influence of water breadth are highlighted.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author. Download presentation by click this link. If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
E N D
Presentation Transcript
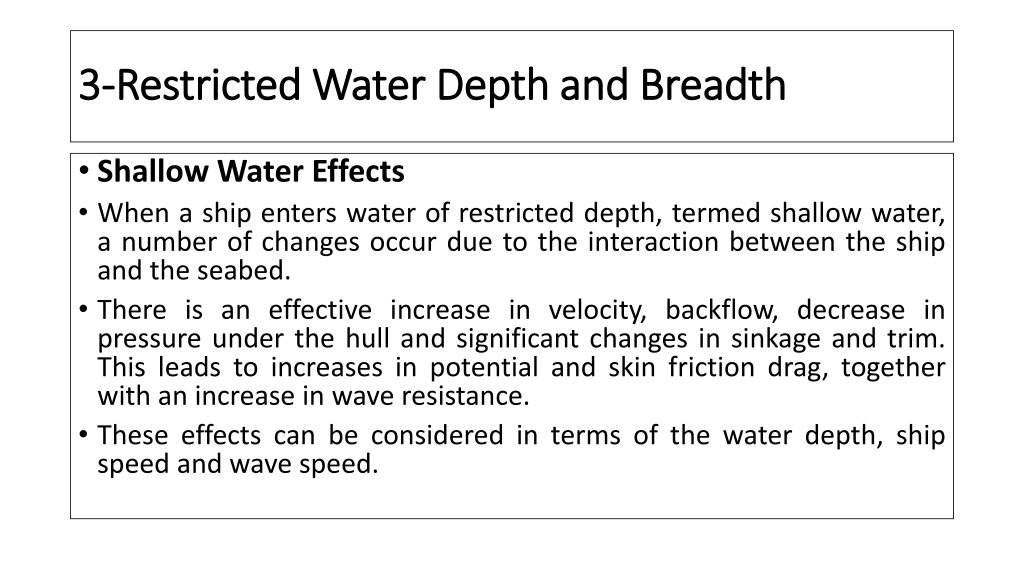
3 3- -Restricted Water Depth and Breadth Restricted Water Depth and Breadth Shallow Water Effects When a ship enters water of restricted depth, termed shallow water, a number of changes occur due to the interaction between the ship and the seabed. There is an effective increase in velocity, backflow, decrease in pressure under the hull and significant changes in sinkage and trim. This leads to increases in potential and skin friction drag, together with an increase in wave resistance. These effects can be considered in terms of the water depth, ship speed and wave speed.
Deep Water When h/ is large, g = c 2 This deep water relationship is suitable for approximately h/ 1/2. Shallow Water When h/ is small, The velocity now depends only on the water depth and waves of different wavelength propagate at the same speed. This shallow water relationship is suitable for approximately h/ 1/20 and c gh = = c gh Critical speed
It is useful to discuss the speed ranges in terms of the depth Froude number, noting that the waves travel at the same velocity, c, as the ship speed V. The depth Froude number is defined as: V gh = Fr h Notice: No transverse waves
The influence of shallow water on the resistance of high-speed displacement monohull and catamaran forms:
Blockage Speed Corrections Blockage Speed Corrections Corrections for the effect of shallow water are generally suitable for speeds up to about Frh= 0.7. They are directed at the influences of potential and skin friction drag, rather than at wave drag whose influence is weak below about Frh= 0.7.
. A commonly used correction is that due to Lackenby [6.4], shown in Figure. This amounts to a correction formula, attributable to Lackenby [6.5] of the following form: This formula is recommended by the International Towing Tank Conference (ITTC) as a correction for the trials procedure (Section 5.3). For higher speeds, a simple shallow water correction is not practicable due to changes in sinkage and trim, wave breaking and other non-linearities These data apply effectively to water of infinite breadth. A limited amount of data is available for the influence of finite breadth.
Landweber [6.8] carried out experiments and developed corrections for the effects of different sized rectangular channels. These data are presented in. An approximate curve fit to the data is. where V is the speed in deep water, Vhis the speed in shallow water of depth h and RHis the hydraulic radius, defined as the area of cross section of a channel divided by its wetted perimeter, that is: RH= bh/(b+ 2h)
Example: A cargo vessel has L = 135 m, B = 22 m and T = 9.5 m. For a given power, the vessel travels at 13 knots in deep water. Determine the speed loss, (a) when travelling at the same power in water of infinite breadth and with depth of water h = 14 m and, (b) in a river with a breadth of 200 m and depth of water h = 14 m when travelling at the same power as in deep water at 8 knots. Neglect any changes in propulsive efficiency.
Squat Squat When a ship proceeds through shallow water there is an effective increase in flow speed, backflow, under the vessel and a drop in pressure. This drop in pressure leads to squat which is made up of vertical sinkage together with trim by the bow or stern. If a vessel is travelling too fast in shallow water, squat will lead to a loss of underkeel clearance and possible grounding.
The following simple formula has been proposed by Barrass and Derrett [6.15] for estimating maximum squat maxin a confined channel such as a river: where CBis the block coefficient, SBis a blockage factor, being the ratio of the ship s cross section to the cross section of the channel, and VSis the ship speed in knots. Maximum squat will be at the bow if CB> 0.700 and at the stern if CB< 0.700. Equation (6.6) may be used for estimating preliminary values of squat and indicating whether more detailed investigations are necessary.
Wave Wash Wave Wash The waves generated by a ship propagate away from the ship and to the shore. In doing so they can have a significant impact on the safety of smaller craft and on the local environment. This is particularly important for vessels operating anywhere near the critical depth Froude number, Frh= 1.0, when very large waves are generated