DC Circuits II: Kirchhoff's Rules & Loop Equations
Today's lecture covers Kirchhoff's rules for DC circuits to analyze current flow and potential changes. The rules include the Junction Rule and Loop Rule, which help determine currents and voltages in complex circuits. Using these rules, we can simplify circuits and solve for unknown variables efficiently. The lecture also discusses solving circuit problems using Kirchhoff's laws and loop equations, demonstrating how to find total effective resistance and voltage drops in circuits.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
DC Circuits II Physics 2415 Lecture 13 Michael Fowler, UVa
Todays Topics Kirchhoff s rules Review RC Circuits Ammeters and Voltmeters
General Circuits: Kirchhoffs Rules Junction Rule: when several wires meet at a point, the total current flowing into the point must equal the total current flowing out. Charge cannot disappear, or pile up at a point. Loop Rule: the total potential (voltage) change on following wires around a loop to your starting point must be zero. (The loop rule is equivalent to saying that if you follow some random path on a hillside, and get back eventually to your starting point, your net change in height above sea level is zero.)
Using Kirchhoffs Rules Junction Rule: as you draw the circuit diagram, use this rule as much as you can to get the smallest number of different unknown currents (one current for each loop). And, the number can sometimes be reduced by symmetry. Loop Rule: the total potential (voltage) change on following wires around a loop to your starting point must be zero: that s adding voltages from batteries to the right IR for each resistance.
Clicker Question A current I flows through the network of resistances (all different) shown. What is the minimum number of unknown currents it is necessary to introduce to find the total effective resistance using Kirchhoff s laws? A. 2 B. 3 C. 4 D. 5 . I R1 R2 R3 R4 R5 I
Clicker Answer A current I flows through the network of resistances (all different) shown. What is the miminum number of unknown currents it is necessary to introduce to find the total effective resistance using Kirchhoff s laws? A. 2 B. 3 C. 4 D. 5 . I R1 R2 I-I1-I2 I1+I2 R3 I2 R5 R4 I-I1 I1 I
The Loop Equations Going round both loops clockwise, ) ) ( 2 1 1 I I R I . I ( ( + = 0 I I R I R I R R1 R2 1 4 2 3 + 1 5 I-I1-I2 I1+I2 ) R3 = 0 I R I R I2 2 2 2 3 and rearranging: ( 4 R R I + ) + = R5 R4 R I R I R I 5 R 1 + 3 2 + 4 I-I1 I1 ( ) = R R I R I 2 1 1 2 3 2 1 Set I = 1 and solve for I1, I2. I
The Loop Equations Having found I1, I2 we can find the voltage drop: along the path abc (see figure) V drops by V I R = + . I c R1 R2 ( ) I-I1-I2 + I I R I1+I2 R3 1 5 1 2 2 I2 and from V = IR we can find the resistance R of this network. If there are batteries within a network, their emf must obviously be included in equating the total voltage change on going around a loop to zero be careful about the battery direction! b R5 R4 I-I1 I1 a I
Batteries in Series and Parallel For batteries in series, the voltages add, in parallel, for identical batteries, they re equivalent to a larger battery of the same voltage. If you put batteries of different voltages in parallel, the stronger will charge the weaker.
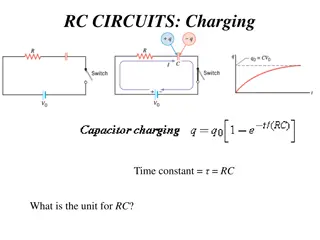
RC Circuits Video! initial charge Closing switch S connects the plates of a charged capacitor by a resistance R. How fast does the charge go down? ( ) Q t V t RI t C dQ t Q t dt RC RC = Q0 -Q0 C S R ( ) dt dQ t ( ) ( ) = = = R V(t) ( ) ( ) ( ) = = / t RC , Q t Q e 0 is called the decay time. t 0 RC 3RC 2RC
Charging a Capacitor initial charge zero On closing the switch, charge will flow from the battery into the initially empty capacitor. But how fast? . C E R = + = E / , / Q RC e / RI Q C = I + dQ dt R E / / V(t) dQ dt = ( ) / t RC 1 Q C E 0 RC 3RC 2RC
Voltmeters and Ammeters Historically, voltmeters and ammeters passed current through a small coil between the poles of a magnet, the coil being free to turn, but against a small spring. The coil formed an electromagnet when current flowed. An ammeter used a low resistance coil, all the current flowed through it. A voltmeter used a high resistance coil, in parallel with the main circuit. V A
Voltmeters and Ammeters An ammeter used a low resistance coil, all the current flowed through it: so inserting the ammeter to measure current slightly reduced the current! A voltmeter used a high resistance coil, in parallel with the main circuit. Inserting the voltmeter in parallel reduced the resistance, and therefore the voltage measured. V . A These meters are in fact obsolete they ve been replaced with DA converters, which affect the quantities being measured far less.
RC Bullet Speed As bullet penetrates gate 1, battery is cut out, capacitor C begins to discharge through R: voltage across capacitor . As bullet goes through gate 2, capacitor is isolated, discharge stops. The final voltage gives bullet time from gate 1 to gate 2. ( ) V e = / t RC V t 0 gate 2 gate 1 volts C V R 0.022 F 180k 8V
RC Bullet Speed: the Calculation As bullet penetrates gate 1, battery is cut out, capacitor C discharges through R: voltage . As bullet goes through gate 2, capacitor is isolated, discharge stops, voltage gives time from gate 1 to gate 2. Taking the initial voltage to be 8V and the final reading to be 4.6V, we have / t RC e V V = ( ) V e = / t RC V t 0 = = / 4.6/8 0.58. 0 From the diagram, = = 0.022 F, so = 4.0 10 sec. 3 180k , t R C RC So = = = 2.2 10 sec. 3 ln0.58 0.55, t 3 4 10 This gives a bullet speed of about 450m/sec.